1 results
Block perturbation of symplectic matrices in Williamson’s theorem
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 67 / Issue 1 / March 2024
- Published online by Cambridge University Press:
- 15 August 2023, pp. 201-214
- Print publication:
- March 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
-
Williamson’s theorem states that for any
$2n \times 2n$ real positive definite matrix A, there exists a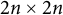 
$2n \times 2n$ real symplectic matrix S such that 
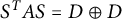$S^TAS=D \oplus D$, where D is an 
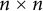$n\times n$ diagonal matrix with positive diagonal entries known as the symplectic eigenvalues of A. Let H be any 
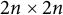$2n \times 2n$ real symmetric matrix such that the perturbed matrix 
$A+H$ is also positive definite. In this paper, we show that any symplectic matrix 
$\tilde {S}$ diagonalizing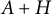 
$A+H$ in Williamson’s theorem is of the form 
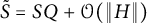$\tilde {S}=S Q+\mathcal {O}(\|H\|)$, where Q is a 
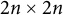$2n \times 2n$ real symplectic as well as orthogonal matrix. Moreover, Q is in symplectic block diagonal form with the block sizes given by twice the multiplicities of the symplectic eigenvalues of A. Consequently, we show that 
$\tilde {S}$ and S can be chosen so that 
$\|\tilde {S}-S\|=\mathcal {O}(\|H\|)$. Our results hold even if A has repeated symplectic eigenvalues. This generalizes the stability result of symplectic matrices for non-repeated symplectic eigenvalues given by Idel, Gaona, and Wolf [Linear Algebra Appl., 525:45–58, 2017].
