The London mathematical instrument maker Henry Sutton (c. 1624–65) has been recognised since his own time as one of the most skilled engravers in his trade in seventeenth-century England. His versatility allowed him to work directly on brass or on wood and also in reverse on a copper printing plate. Thus much of his surviving oeuvre is bound into books, although a number of his printed instruments have survived as single printed sheets, applied to a brass plate or more usually a wooden board.
The instruments of his preserved at the Whipple Museum are among those generally cited by collectors, curators, and instrument historians to justify a reputation that has continued to the present. Sutton’s reputation is the theme of this chapter: how it was promoted and established in his lifetime, and how it survived him for a century or so, not simply for connoisseurs but for mathematical practitioners. The pioneering chronicler of these practitioners, Eva Taylor, offered a very fair assessment: ‘one of the best known engravers of scales, quadrants, etc., of his day, was renowned for his accuracy and was in demand for drawing diagrams for mathematical books’.Footnote 1 Engraving skill, accuracy, and books were pillars of Sutton’s work, and this account of the renown it achieved will be intertwined with a consideration of his instruments, specifically the horary quadrants.
Sutton made a great variety of mathematical instruments, and seems to have relished those requiring sets of engraved projection lines, such as astrolabes, types of horary quadrant, and William Oughtred’s ‘horizontal instrument’. Most of the well-known museums containing seventeenth-century instruments have a few in their collections, with the Whipple Museum holding a particularly rich and varied selection. At thirteen instruments, the Whipple’s collection of Sutton material may be the largest of any museum. Further, the Whipple has been a site for scholarship on Sutton’s instruments. David Bryden’s work showed a particular interest in Sutton,Footnote 2 and latterly Boris Jardine has produced a number of in-depth studies that have shown the benefits of looking at his instruments in detail.Footnote 3
The Whipple Museum has four quadrants by Sutton, one in brass (using Gunter’s projection) and three printed on paper (using the projections associated with Sutton and considered below). Of the printed instruments, a smaller one is applied to only one side of a brass quadrant shape, whereas two larger ones each have two sides and are mounted on wood.Footnote 4
To begin with one of his instruments that is not in Cambridge but which can introduce the theme of reputation, the History of Science Museum in Oxford has a large universal astrolabe by Sutton, constructed on an orthographic (Rojas) projection and dated 1659.Footnote 5 There are a great many, very regularly engraved lines, in Sutton’s typical manner and demonstrating his celebrated skill. There is also a remarkable companion: the Museum has an early print taken directly from this instrument, which must have been inked as though it were a printer’s plate.Footnote 6 There has been discussion over the intention behind this pull and other direct prints from instruments, including others made by Sutton.Footnote 7 Being in reverse, they have no obvious use as instruments. Might they, for example, have been kept for record in the workshop or used to encourage future customers? Is it possible that the reverse print was an intermediate step to a counter-print? All these ideas have been canvassed but, whatever the intention, this astrolabe print is proof of quite exceptional skilled practice. It is much more difficult to notice any flaws in the brass plate, but any untidiness or unevenness of line will immediately be revealed by the print. While there is some leeway with a figurative print, here there is nowhere to hide faults in a challenging and detailed projection. As a demonstration of Sutton’s accuracy, skill, and command, the print is extraordinary (Figure 4.1). Making such a print was an act of bravado, while preserving it was a statement of success. If Sutton himself made this print, as seems very likely, it surely reveals a concern for reputation.
Sutton’s Quadrants
That concern is shown in a better-documented manner around the same time, one that gives us a more nuanced view of reputation in contemporary mathematics. In 1658 a book by the mathematician John Collins was published in London under the title The Sector on a Quadrant, or, a Treatise Containing the Description and Use of Three Several Quadrants.Footnote 8 Its author was described as ‘Accountant, and Student in the Mathematiques’, the printer was J. Macock, and the book was to be sold by two booksellers, George Hurlock and William Fisher, and also by ‘Henry Sutton Mathematical Instrument Maker, at his House in Thredneedle street behind the Exchange.’ The engraver of the plates is given unusual prominence on the title page and the relationship of the plates to the book is stated as ‘With large Cuts of each Quadrant, printed from the original Plates graved by Henry Sutton, either loose, or pasted upon Boards’. Thus began a complex bibliographical sequence, where the content remained much the same, but was introduced by a variety of title pages. As early as 1659 it had been decided that there were in fact four quadrants, not three, though this was not altered in the preface.Footnote 9 The new title page insisted that there were ‘Two small ones and two great ones’ and then repeated that each was ‘rendred many wayes, both general and particular’. The author was now described as ‘Accountant Philomath’. A third bookseller, Thomas Pierrepont, was added and the description of the printed instruments altered to ‘With Paper Prints of each Quadrant, either loose or pasted upon boards; to be sold at the respective places aforesaid’.Footnote 10 This reduced the former emphasis on the work of engraving and the prominence given to Sutton. There are copies containing both these title pages.
The relationship between printed instruments bound into books and separate paper instruments pulled from the same plate is a topic of interest to instrument historians, bearing as it does on the nexus of connections between engraving and the production of instruments and books. The wording on the title pages seems to imply that the instruments are supplied separately from the book, the options mentioned being ‘either loose or pasted upon boards’, but this does not preclude their inclusion in the bound book, and in fact one plate includes the instruction ‘Place this next after the Title page.’ This is one of the smaller quadrants, and neither side of either of the larger quadrants is ever found in the book, although the scales and their uses are explained there. They would have had to have been folded down to fit within the usual quarto format.
Books such as this and their plates need to be studied in parallel with the printed instruments in collections such as that in the Whipple Museum. The bound prints and those pasted onto boards were produced from the same engraved plates. Museums with collections of early books or with associated libraries have perhaps been remiss in ignoring instruments by makers represented in their collections, simply because they happen to be bound into books. In Sutton’s case the bound examples were probably not intended to have much use as instruments (though a solar declination scale, for example, could find ready applications). In the previous century, however, many such prints in books were certainly working instruments, a status emphasised by the inclusion of rotating discs and strings for reading scales.
In the sixteenth century, the instrument designer, and perhaps even the head of the workshop or print-shop, might also be the author. By the middle of the seventeenth century this was not at all common, and The Sector on a Quadrant brings a new collaborator into play, and a new relationship that would shape the venture. This book was a new departure for John Collins, who had previously published only some tables for currency exchange between England and Flanders, and England and France, and a short textbook on accountancy, An Introduction to Merchants Accounts in 1653. He had a sudden flowering in the field of mathematical instruments, with three books appearing in the late 1650s: The Sector on a Quadrant (1658), Navigation by the Mariners Plain Scale New Plain’d (1659), and Geometrical Dyalling (1659). All were linked to Sutton in some way, either as a stockist and seller, or as one of the publishers, and as the engraver for all three books.
The bibliography of these books is complex and requires more space and skill than are available here, but of the three titles it seems that The Sector on a Quadrant was the first to appear, which adds to the interest of an account of the genesis of the book, explained in unusual detail in a preface by Collins. It relates to Collins’s entry into this field, as well as to Sutton’s relationships with his clients and his quest for reputation. ‘Thou hast in this Treatise’, says Collins, ‘the Description and Uses of three several Quadrants, presented to thy View and Acceptance; and here I am to give thee an account of their Occasion and Original.’Footnote 11
The account is that a mathematical friend of Collins, Thomas Harvie, had worked out an idea for a quadrant, which he drew out on paper. It was a new design with a novel projection in the context of an horary quadrant, that would yield the time and the solar azimuth from the customary quadrant measurement of solar altitude. Harvie wanted to have one in brass for his own use, and approached Sutton, as an instrument maker. Having been told the general idea, Sutton agreed to make the instrument, and Harvie said he would come back in two weeks with the projection drawn out for him to copy in brass. Before this could happen, Collins tells us that
M. Sutton having very good practise and experience in drawing Projections, speedily found out the drawing of that Projection, either in a Quadrant or a Semicircle, without the assistance of the promised directions, and accordingly, hath drawn the shape of it for all Latitudes, and also found how the Horizontal Projection might be inverted and contrived into a Quadrant without any confusion, by reason of a reverted tail, and let me further add, that he hath taken much pains in calculating Tables for the accurate making of these and other Instruments, in their construction more difficult then any that ever were before.Footnote 12
Sutton asked Collins to write a few sheets on the use of the quadrant, for him to give to customers, when he supplied them with instruments. Once again matters were overtaken by Sutton’s enthusiasm. He became dissatisfied with the idea of a few sheets and since, as Collins says, he ‘very well understood the use as well as the making’Footnote 13 and had found many uses for his quadrant, he persuaded Collins to write a much fuller treatise. Sutton had also come up with further designs, and he continued to press ahead, engraving the plates after Collins had written the treatise and making some changes from the drawings from which Collins had been working. This meant that the text and plates, and therefore the instruments, did not quite coincide. In particular, whereas Collins had used right ascensions from current star tables, Sutton had calculated those on the quadrant for a slightly later epoch, so as to lengthen the useful life of the instruments.
We learn a great deal from this preface about the relationships (almost certainly not typical) between the client, Harvie, the instrument-maker, Sutton, and the mathematical practitioner Collins. It is not insignificant that Harvie took his commission to Sutton in the first place. This tells us something about Sutton’s reputation: he was not restricted to the standard designs, but would make a bespoke instrument on an original pattern. We learn of Sutton’s very active engagement with the process, something that might easily have gone unrecorded. That it was recorded was also surely at Sutton’s instigation. It is hard to see that Collins himself had anything to gain by the publication of this preface, though through the project itself, centred around Sutton’s engraving of some very fine plates, he did achieve a successful book. Through this and the other titles, we know that he and Sutton were in a broader productive collaboration around this time.
The importance of Sutton’s initiative survived in Collins’s memory, when he wrote as follows in a later letter to John Wallis:
At the request of Mr Sutton I wrote a despicable treatise of quadrants. His design was to demonstrate himself to be a good workman in cutting the prints of those quadrants, and thereby to obtain customers.Footnote 14
We should not set too much store by the word ‘despicable’. Aware of his humble origins and lack of formal education, Collins was inclined to refer to his work with excessive modesty, especially in writing to the renowned Professor of Geometry at Oxford. It is clear from his substantial book that Collins was thoroughly engaged with The Sector on a Quadrant.
Collins begins his account by offering two ways of thinking about the two projections to be used in the quadrants. His first way of thinking is related, he says, to how the projections ‘may be demonstrated’. In the future we may expect a more general demonstration from Harvie, but for now the projections can be thought of as deriving either from Stoeffler’s astrolabe (as he calls the ordinary astrolabe, and referring in particular to the projection of a latitude plate or tympan), or (in the second projection) from the horizontal instrument of William Oughtred.
In the former projection the circles on the quadrant are the projections of the lines of altitude and azimuth, the point of projection is the south celestial pole, and the plane of projection contains the equator. The lines on the quadrant are, unlike the astrolabe, the projection of the altitude and azimuth below the horizon as far as the tropic of Capricorn, which, however, we are then advised to call the Tropic of Cancer. This is the projection used in three of the quadrants, the first three described by Collins, two of which are generally included as prints, although this cannot be assumed and neither can the positions of the prints in the text.
In the latter projection the circles to be projected are those of declination and right ascension (or hour lines), the point of projection is the observer’s nadir, and the plane of projection contains the horizon. On the quadrant the projected lines are the arcs of these circles below the horizon and the user has to adopt a similar reversal in nomenclature between the tropics. This projection is used only for the fourth quadrant, which is never found as a print in the book.
As Collins admits, this is rather an unhelpful and counter-intuitive way of thinking about the two projections, but its purpose seems to be to relate the projections to the established work of Stoeffler and Oughtred. For Collins this constitutes a form of ‘demonstration’: these projections can be taken as established and something that simply extends them to cover a differently delimited area of the celestial sphere also partakes of that status. Surprisingly perhaps, he says that he gives this view ‘for the accommodation of Instrument makers, to whom this Derivation may seem most suitable’,Footnote 15 implying that they are the group who will want to see these new instruments within the established canon of projections of the sphere.
Collins then offers what he calls ‘a more immediate account’ of the projections, a view it seems was more readily understood. Now for the former projection he says that the point of projection is the north celestial pole, the plane of projection is equatorial, and the projected arcs are those of altitude and azimuth above the horizon and falling between the tropics, with the Tropic of Cancer being outermost on the projection and Capricorn innermost, that is, the reverse of ‘Stoeffler’s astrolabe’. For the horizontal projection, he now places the point of projection at the observer’s zenith, projecting the lines of solar declination and right ascension onto the horizontal plane. This is the reverse of Oughtred’s projection.
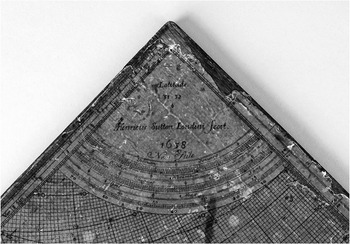
There is confusion over the naming of these quadrants, through the uses of ‘small’ and ‘universal’. Collins begins with an instrument he refers to simply as the quadrant. He describes its use at length, especially the lines and scales that allow proportional and trigonometrical calculations, i.e. the features that make this a ‘sector on a quadrant’, as many of these operations could be performed with a sector. Then he uses a further title page to announce The Description and Uses of a Great Universal Quadrant: With a Quarter of Stoflers Particular Projection upon it, Inverted, dated 1658 and describing the author as ‘Accomptant, and Student in the Mathematiques’. What is meant by ‘universal’ here is not clear (and seems to be contradicted by ‘particular’), as this instrument uses the same projection and is for a specific latitude (Figure 4.2). The previous instrument is now referred to as ‘the small Quadrant’. Collins then describes the additional features on this larger quadrant and their use.
Both of these quadrants make use of what Collins calls the ‘reverted tail’. This is a device he specifically attributes to Sutton, which is used to accommodate all the projection lines on the instrument, even though a portion as projected will fall outside the limits of a quadrant. The portions of the projected lines that fall beyond the 6 o’clock line to the north of the east or west point on the horizon (these points coincide on the ‘folded’ projection), needed for finding the time before 6 am and after 6 pm in summer, will lie outside the quadrant. However an equivalent, unused space arises from the sun being below the horizon after 6 am and before 6 pm in winter (Figure 4.3). Sutton uses this space – smaller in area but with more closely packed lines – by adding the lines of negative altitude or depression and continuing the azimuth lines to the section of sky below the horizon and bounded by the Tropic of Capricorn and the 6 am/pm line.
To understand the use of the reverted tail, we must first be familiar with the normal operation for finding time. A bead slides friction-tight on a weighted thread suspended from the apex of the quadrant and must be adjusted (‘rectified’) for date (or solar declination). This is done by stretching the thread across the date point on the calendar scales close to the apex and setting the bead to the summer or winter section of the ecliptic line on the projection. The altitude is then measured by holding the quadrant vertical, aligning the edge sights with the sun, and noting the angle on the altitude scale at the limb. The bead is then placed on the equivalent line in the projection and the time found on the hour scales at the limb, where morning and afternoon hours run in opposite directions. This is very like the method of finding time with an astrolabe, here accommodated to a quadrant.
In the geometry of the projection the lines in the reverted tail are equivalent to those that would fall outside the quadrant area, but to use them for the absent dates and times the user must set the bead on the plumb-line to the winter ecliptic line even though the thread is stretched across a summer date, and must read the time from the ‘wrong’ hour scale on the limb – the afternoon hours in the morning and vice versa. Sutton’s facility with projection allowed him to see this with ease, but his customers surely found it confusing.
Sutton seems to relish the opportunity to demonstrate his facility with projection in other ways in Collins’s book. ‘For varieties sake’ he projects quadrants for different latitudes, illustrating the unexpected behaviour of the sun at a low latitude (Barbados, 13 °N) and a high one (Greenland, 75 °N). He also provides the projection of a full semicircle for the latitude of London, its advantages, he explains, being that there is no need for the operation of a reverted tail and that the projected area is narrower, not needing to accommodate the full range of solar altitudes at a given latitude within the space of a quadrant. Sutton wants it to be clear that he has not simply engraved a drawing projected by someone else: he signs the plate ‘Henricus Sutton Londini deline= et sculp=’.Footnote 16
Collins then moves on to ‘The Description of an Universal small Pocket Quadrant’,Footnote 17 having scales on only one face. It can be small because the projection has lines only of solar altitude and the azimuth lines are not present. This allows Sutton to have summer and winter lines crossing each other, the same area of the quadrant being used for the northern and southern hemispheres and the outermost arc on the projection standing for either tropic. The Whipple Museum has an example unusually applied to a brass quadrant (Figure 4.4). A copy in the British Library of the relevant pages from Collins’s book, with the printed plate, belonged to Robert Hooke.Footnote 18
Finally, Collins describes the fourth quadrant, again a larger one and the only one based on his ‘second’ projection, which he describes as an inversion of the projection used for Oughtred’s horizontal instrument. A final title page, dated 1658, which is also the date on all the plates, announces The Description and Uses of a General Quadrant, with the Horizontal Projection, upon it Inverted, and the instrument is referred to as ‘the other great quadrant’ or ‘the horizontal quadrant’. Here again there is no plate with the book, but one surviving printed instrument is known, at the History of Science Museum in Oxford. It is not signed but is attributed to Sutton.Footnote 19 In Collins’s book there follows a table of solar right ascension and declination calculated for 1666 by Sutton.
Collins may have written to Wallis in February 1665/6 that Sutton’s intention for The Sector on a Quadrant ‘was to demonstrate himself to be a good workman in cutting the prints of those quadrants’, but the book itself shows that this was not the whole story. Otherwise there would be no reason for the unexpected preface, where, as Collins puts it, ‘I am to give thee an account of their Occasion and Original’.Footnote 20 Sutton is explicit in his ‘deline= et sculp=’ inscription that he made the projection as well as the plate, so his reputation should encompass his facility with compass and rule, as well as with the burin.Footnote 21
Sutton’s Reputation
Sutton did achieve a substantial reputation, extending to the circle of the Royal Society. Of his death in the Plague of 1665, Sir Robert Moray wrote to Henry Oldenburg in October, ‘wee all here are much troubled with the loss of poor [Anthony] Thomson & Sutton.’Footnote 22 Collins also wrote to John Wallis that on his return to London from Oxford, ‘I found wanting Mr Anthony Thompson and Mr Henrie Sutton, two of the best Mathematicall Instrument Makers.’Footnote 23 We know of communication on dialling between Sutton and ‘Doctor Richard Sterne’,Footnote 24 who in all probability was the former master of Jesus College, Cambridge, who became Archbishop of York after the Restoration. Out of favour during the Commonwealth, he earned a living as a schoolmaster.Footnote 25
In 1668 the mathematical writer Robert Anderson published his Stereometrical Propositions, which he claimed would be useful for gauging, and announced that an instrument he recommended could be had from John Marke, who, he said, ‘was formerly Servant to that incomparable Instrument maker Mr. Henry Sutton’.Footnote 26 In his letter to Wallis, Collins had mentioned Marke’s succeeding to the business, ‘We hope he may prove as good a Workeman as his deceased Master.’Footnote 27
We shall see that Sutton’s skill as an engraver was too valuable to allow his output to end with his death, but his reputation as a designer of instruments was less robust. In 1669 Robert Morden, an associate of Anderson and a maker of globes and seller of maps and instruments, published A Description & Use of a Large Quadrant, Contrived and Made by H. Sutton.Footnote 28 At this stage Sutton was understood not only to have made the quadrant but also to have contrived it. No author is credited and it is clear that this tract was meant to sit alongside the great quadrant, still available either from a stock of prints or pulled from the surviving plates. David Bryden mentions further early references to Sutton and his quadrant within the instrument trade.Footnote 29
In 1703 there appeared the first edition of John Harris’s The Description and Uses of the Celestial and Terrestrial Globes; and of Collins’s Pocket Quadrant. The uses of the globes were a staple component of Harris’s teaching, including his public lectures at the Marine Coffee House, and he explained that ‘The Description and Use of Mr. Collins’s Quadrant was occasioned by the Request of some Persons who would gladly know the best Uses of it, without being obliged to read over many Things which are little to their Purpose.’Footnote 30 Clearly The Sector on a Quadrant was no longer what was wanted. The quadrant Harris describes is the basic Sutton instrument with altitude and azimuth lines, but he neglects the verso, referring his readers to the ‘large Account’ in Collins’s book.Footnote 31 Sutton is not mentioned in any capacity. While there are four pages advertising books from the bookseller or publisher, readers are offered no advice on how to obtain a quadrant, which seems to imply that this was not difficult. The book went through a number of editions up to at least 1751.Footnote 32
In 1710 the surveyor, dialist, and teacher of mathematics, John Good,Footnote 33 brought out a much-abridged account of the Sutton quadrants in a tract titled The Description and Use of Four Several Quadrants, Two Great Ones, and Two Small Ones. Sutton would not have been happy with the distribution of credit on the title page: ‘Invented and Written by the Ingenious Mr. John Collins, and Engrav’d by the Curious Hand of Mr. Henry Sutton’.Footnote 34 The same view is repeated in the preface: all the instruments were ‘invented by the Ingenious Mr. John Collins, and Engrav’d by that unparallel’d Artist Mr. Henry Sutton, Mathematical-Instrument-Maker’. Good explains that as the original book is ‘now scarce and out of Print’, he has ‘drawn from it the usefullest Parts thereof’.
While Sutton (an instrument-maker) was ignored completely by John Harris (a successful clergyman, Royal Society fellow, and Boyle lecturerFootnote 35), even for John Good Sutton’s reputation rested on his engraving: he is ‘that unparallel’d Artist’. Since, however, he is referred to as the engraver and since Good’s text would have no purpose without the instruments, it is reasonable to assume that Sutton’s plates had survived and that prints from them could be bought, perhaps from the promoters of Good’s book. The successful chartmaker and bookseller Richard Mount was the publisher, in association with William Mount and Thomas Page. There was much acquisition of stock in books, maps, and plates between those engaged in mathematical commerce; Mount, for example, purchased the stock of instrument- and globe-maker Charles Price in 1706.Footnote 36 Price had been apprenticed to John Seller, and was in partnership for a time with John Senex.Footnote 37 For men such as these a copperplate by Henry Sutton would be a valuable commodity, and his reputation as an outstanding engraver would have helped preserve such an item more effectively than if Sutton had been remembered as an inventor or designer.
Edmond Stone described Sutton’s quadrant (the equatorial projection of altitude and azimuth lines, in two sizes) in his translation and edition of Nicolas Bion’s The Construction and Principal Uses of Mathematical Instruments, published by John Senex in 1723. This was, Stone says, one of several different quadrants ‘made by Mr. Sutton long since’ and, while ‘made by’ is ambiguous, no other designer is mentioned, while Collins is referred to only as the author of the book where they are described.Footnote 38
Good’s book appeared again in 1750, published once more by Mount (now W. and J.) and Page, and, although the title page and text generally have been reset, the attributions to Collins and Sutton are unchanged.Footnote 39 Spelling is updated and grammar corrected, but examples that were updated from the year 1657 in The Sector on a Quadrant to 1709 in the 1710 edition are repeated unchanged in 1750. The Julian calendar is assumed, even though this is in the process of being abandoned in mid-century. Can we imagine that Sutton’s plates survived still and that after a further forty years Mount and Page were still hoping to sell prints? It is hard to see why else they would have produced this revised edition, which continued to reference the work of the ‘unparallel’d Artist’.
A second and augmented edition of Stone’s Bion appeared in 1758, and several historians have noted the fulsome tribute paid to Sutton’s quadrants in the introduction to Stone’s ‘Supplement’.Footnote 40 In justification for adding English instruments to Bion’s account, the first instance Stone cites was as follows:
I soon perceived that many French Instruments of Mr Bion’s were excelled by some of ours, of the same kind in Contrivance; and as to Workmanship, I never did see one French Instrument so well framed and divided, as some of ours have been; for Example, Mr Sutton’s Quadrants, made above one hundred Years ago, are the finest divided Instruments in the World; and the Regularity and Exactness of the vast Number of Circles drawn upon them is highly delightful to behold.Footnote 41
Stone’s account is to some extent historical, and we cannot infer from his description the availability of prints from any surviving plates. A recent acquisition by the Whipple Museum, however, does give us an unexpected coda to the history of at least one and probably two of the copperplates. In 2017 an example of the large version of the equatorial quadrant, with the two prints pasted onto a shaped wooden board in the usual way, was donated to the Museum. The verso has the customary scales, with the calendars, for example, unchanged, but the quadrant itself, on the front, has an unexpected feature. The date or solar declination scale, set out towards the apex in four quadrant sections, has been skilfully replaced by one based on the Gregorian calendar and is inscribed ‘New Stile’ (Figure 4.5).Footnote 42 The legislation for adopting the Gregorian calendar was approved by Parliament in 1751 and the new calendar introduced the following year. The modification of Sutton’s plate indicates that it had survived in a practical context for somewhere around a century.
‘Sutton’s Quadrant’?
Sutton wanted to have his engagement with geometry recognised alongside his skill as an engraver. However differently we may distinguish mathematical proficiency today, it is clear that, in Sutton’s world, facility with projective technique counted as a species of mathematics. Sutton failed to achieve his dual ambition: by the eighteenth century he was not remembered as a competent geometer but as an ‘unparallel’d Artist’.
In spite of eighteenth-century instances of naming the instrument after Collins, notably by John Harris, today the equatorial instrument, at whatever size, is generally referred to as ‘Sutton’s quadrant’. There are occasional reversions to Collins and even very occasional support for ‘Harvey’s quadrant’, but Collins never claimed the instrument as his invention, and Harvey is surely too shadowy a figure and his connection too slightly documented to justify this name. The name itself might seem unimportant, but not if ‘Collins’ was introduced on the basis of a prejudice towards a mathematician and Fellow of the Royal Society over an instrument-maker. Collins wrote an account of the instrument that devoted more space to mathematical calculation than to instrumental astronomy and the odd title to his book, The Sector on a Quadrant, reflects this. It was mainly these sections that later writers stripped away.
In another sense as well, this quadrant began and remained as Sutton’s. Unlike Gunter’s quadrant, and in spite of surviving interest in published accounts, other makers did not take up Sutton’s design with any enthusiasm. There are a very few instances, but nothing substantial,Footnote 43 and engraving the projection was a challenge. In its near-exclusive use by Sutton, both living and posthumous, the quadrant embodies his geometry and his engraving together, while nothing we have seen here suggests that Sutton himself would have made this distinction.