No CrossRef data available.
Article contents
Some remarks on a quasi-steady-state approximation of the Navier-Stokes equation
Published online by Cambridge University Press: 17 February 2009
Abstract
A quasi-steady-state apprcncimation to the Navier-Stokes equation is the corresponding equation with nonhomogeneous forcing term f(x, t), but with the term Vt deleted. For solutions that are zero on the boundary, the difference z between the solution of the Navier-Stokes equation and the solution of this quasi-steady-state approximation is estimated in the L2 norm ║z║ with respect to the spatial variables. For sufficiently large viscosity or sufficiently small body force f, the inequality
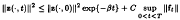
holds for 0 < t ≤ T and certain real numbres C, β > 0.
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Society 1988


