Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-27T06:40:16.953Z Has data issue: false hasContentIssue false
  • English
  • Français

Nilpotency and related properties of group extensions

Published online by Cambridge University Press:  17 April 2009

David Shield  and
Hanna Neumann
David Shield
Affiliation:
School of General Studies, Australian National University, Canberra, ACT.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Gilbert Baums lag has given sufficient conditions for a group G to be nilpotent in terms of properties of a normal subgroup H and the quotient G/H, and has shown these conditions to be necessary when G is a wreath product and H its base group. His results are extended here to give corresponding conditions for weaker properties ranging from “bounded local nilpotency” to “being an Engel group”. Again we ask whether the sufficient conditions found are necessary when G is non-trivially a wreath product, or is of the form 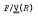the answer is “yes” for the stronger properties considered, but for the weaker ones the question remains open.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 4 , Issue 2 , April 1971 , pp. 145 - 154
Copyright
Copyright © Australian Mathematical Society 1971

References

[1]Allenby, R.B.J.T., “Adjunction of roots to groups”, (M.Sc.Tech. thesis, University of Manchester, 1963).Google Scholar
[2]Baumslag, Gilbert, “Wreath products and p-groups”, Proc. Cambridge Philos. Soc. 55 (1959), 221–231.CrossRefGoogle Scholar
[3]Golod, E.S., “Nil-algebras and residually finite p-groups” (Russian) Izv. Akad. Nauk SSSR Ser. Mat. 28 (1964), 273–276.Google Scholar
[4]Liebeck, Hans, “Concerning nilpotent wreath products”, Proc. Cambridge Philos. Soc. 58 (1962), 443–451.CrossRefGoogle Scholar
[5]Meldrum, J.D.P., “Central series in wreath products”, Proc. Cambridge Philos. Soc. 63 (1967), 551–567.CrossRefGoogle Scholar
[6]Meldrum, J.D.P., “On nilpotent wreath products”, Proc. Cambridge Philos. Soc. 68 (1970), 1–15.CrossRefGoogle Scholar
[7]Morley, Larry, “Bounds on the nilpotency class of certain semi-direct products”, submitted to Trans. Amer. Math. Soc.Google Scholar
[8]Neumann, Hanna, Varieties of groups (Ergebnisse der Mathematik und ihrer Grenzgebiete, Band 37, Springer-Verlag, Berlin, Heidelberg, New York, 1967).CrossRefGoogle Scholar
[9]Scruton, Teresa, “Bounds on the class of nilpotent wreath products”, Proc. Cambridge Philos. Soc. 62 (1966), 165–169.CrossRefGoogle Scholar
[10]Šmel'kin, A.L., “Wreath products and varieties of groups” (Russian), Izv. Akad. Nauk SSSR Ser. Mat. 29 (1965), 149–170.Google Scholar
[11]Zorn, Max, “Nilpotency of finite groups”, Bull. Amer. Math. Soc. 42 (1936), 485.Google Scholar