Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-10T16:28:26.739Z Has data issue: false hasContentIssue false
  • English
  • Français

ON A PROBLEM OF PRAEGER AND SCHNEIDER

Part of: Representation theory of groups Structure and classification of infinite or finite groups

Published online by Cambridge University Press:  27 May 2019

EGLE BETTIO  and
ENRICO JABARA
EGLE BETTIO
Affiliation:
Liceo Benedetti–Tommaseo, Castello 2835, 30122 Venezia, Italy email egle.bettio@istruzione.it
ENRICO JABARA*
Affiliation:
Dipartimento di Filosofia, Università Ca’ Foscari, Dorsoduro 3484/D, 30123 Venezia, Italy email jabara@unive.it
*
✉jabara@unive.it
Get access Rights & Permissions [Opens in a new window]

Abstract

This note provides an affirmative answer to Problem 2.6 of Praeger and Schneider [‘Group factorisations, uniform automorphisms, and permutation groups of simple diagonal type’, Israel J. Math. 228(2) (2018), 1001–1023]. We will build groups 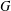$G$ (abelian, nonabelian and simple) for which there are two automorphisms 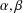$\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D6FD}$ of 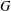$G$ such that the map

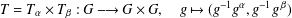$$\begin{eqnarray}T=T_{\unicode[STIX]{x1D6FC}}\times T_{\unicode[STIX]{x1D6FD}}:G\longrightarrow G\times G,\quad g\mapsto (g^{-1}g^{\unicode[STIX]{x1D6FC}},g^{-1}g^{\,\unicode[STIX]{x1D6FD}})\end{eqnarray}$$
is surjective.

Keywords

uniform automorphismsfactorisations of direct productsprimitive groups

MSC classification

Primary: 20E36: Automorphisms of infinite groups
Secondary: 20D40: Products of subgroups
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 101 , Issue 1 , February 2020 , pp. 80 - 87
Copyright
© 2019 Australian Mathematical Publishing Association Inc. 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Jabara, E., ‘Una nota sui gruppi dotati di un automorfismo uniforme di ordine potenza di un primo’, Rend. Semin. Mat. Univ. Padova 84 (1990), 217–221.Google Scholar
Lyndon, R. C. and Schupp, P. E., Combinatorial Group Theory, Classics in Mathematics (Springer, Berlin, 2001).Google Scholar
Praeger, C. E. and Schneider, C., ‘Group factorisations, uniform automorphisms, and permutation groups of simple diagonal type’, Israel J. Math. 228(2) (2018), 1001–1023.Google Scholar
Robinson, D. J. S., ‘A theorem on finitely generated hyperabelian groups’, Invent. Math. 10 (1970), 38–43.Google Scholar
Rotman, J. J., An Introduction to the Theory of Groups, 4th edn (Springer, New York, 1995).Google Scholar
Rowley, P., ‘Finite groups admitting a fixed-point-free automorphism group’, J. Algebra 174(2) (1995), 724–727.Google Scholar
Springer, T. A., Linear Algebraic Groups (Birkhäuser, Boston, 1998).Google Scholar
Zappa, G., ‘Sugli automorfismi uniformi nei gruppi di Hirsch’, Ric. Mat. 7 (1958), 3–13.Google Scholar