Article contents
On Hau's lemma
Published online by Cambridge University Press: 17 April 2009
Abstract
Let f ∈ ℤ[X] and let q be a prime power pl(l ≥ 2). Hua stated and proved that
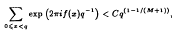
for some unspecified constant C > 0 depending on the derivative f′ of f; M denoting the maximum multiplicity of the roots of the congruence p−tf′(x) ≡ 0 (mod p), where t is an integer chosen so that the polynomial p−tf′(x) is primitive. An explicit value for C was given by Chalk for p ≥ 3. Subsequently, Ping Ding (in two successive articles) obtained better estimates for p ≥ 2.
This article provides a better result, based upon a more precise form of Hua's main lemma, previously overlooked.
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 50 , Issue 3 , December 1994 , pp. 451 - 458
- Copyright
- Copyright © Australian Mathematical Society 1994
References
- 9
- Cited by


