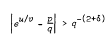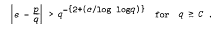Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Durand, Alain
1976.
Note on rational approximations of the exponential function at rational points.
Bulletin of the Australian Mathematical Society,
Vol. 14,
Issue. 3,
p.
449.
Durand, Alain
1980.
Simultaneous diophantine approximations and Hermite's method.
Bulletin of the Australian Mathematical Society,
Vol. 21,
Issue. 3,
p.
463.
Mahler, K
1982.
Fifty years as a mathematician.
Journal of Number Theory,
Vol. 14,
Issue. 2,
p.
121.
Leppälä, Kalle
Matala-aho, Tapani
and
Törmä, Topi
2017.
Rational approximations of the exponential function at rational points.
Journal of Number Theory,
Vol. 179,
Issue. ,
p.
220.
Begunts, Aleksandr Vladimirovich
2020.
О сходимости знакопеременных рядов,
связанных с последовательностью Битти.
Математические заметки,
Vol. 107,
Issue. 2,
p.
299.
Begunts, A. V.
2020.
On the Convergence of Alternating Series Associated with Beatty Sequences.
Mathematical Notes,
Vol. 107,
Issue. 1-2,
p.
345.