Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-28T03:12:40.814Z Has data issue: false hasContentIssue false
  • English
  • Français

Extended Chromatic Polynomials

Published online by Cambridge University Press:  20 November 2018

Andrew Sobczyk  and
James O. Gettys Jr.
Andrew Sobczyk
Affiliation:
Clemson University, Clemson, South Carolina
James O. Gettys Jr.
Affiliation:
Parker High School, Greenville, South Carolina
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let G be a finite graph with non-empty vertex set (G) and edge set 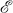(G) (see [2]). Let λ be a positive integer. Tutte [5] defines a λ-colouring of G as a mapping of (G) into the set Iλ = {1, 2, 3, …, λ} with the property that two ends of any edge are mapped onto distinct integers. The elements of Iλ are commonly called “colours.” If P(G, λ) represents the number of λ-colourings of G, it is well known that P(G, λ) can be expressed as a polynomial in λ. For this reason P(G, λ) is usually referred to as the chromatic polynomial of G.

The chromatic polynomial P(G, λ) was first suggested as an approach to the four-colour conjecture. To quote Tutte [5]: ”… many people are specially interested in the value λ = 4.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 24 , Issue 3 , June 1972 , pp. 492 - 501
Copyright
Copyright © Canadian Mathematical Society 1972

References

1. Gleason, A. M. and Greenwood, R. E., Combinatorial relations and chromatic graphs, Can. J. Math. 7 (1955), 1–7.Google Scholar
2. Frank, Harary, Graph theory (Addison-Wesley, Reading, Mass., 1969).Google Scholar
3. Ryser, H. J., Carus Monograph No. 14, Combinatorial Mathematics (Wiley, New York, 1963).Google Scholar
4. Andrew, Sobczyk, Graph-colouring and combinatorial numbers, Can. J. Math. 20 (1968), 520–534.Google Scholar
5. Tutte, W. T., Lectures on chromatic polynomials, Institute of Statistics Mimeo Series, No. 600. 25.Google Scholar