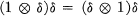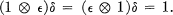Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Sweedler, Moss Eisenberg
1968.
Cohomology of algebras over Hopf algebras.
Transactions of the American Mathematical Society,
Vol. 133,
Issue. 1,
p.
205.
Heyneman, Robert G
and
Sweedler, Moss Eisenberg
1969.
Affine Hopf algebras, I.
Journal of Algebra,
Vol. 13,
Issue. 2,
p.
192.
Allen, Harry Prince
and
Sweedler, Moss Eisenberg
1969.
A theory of linear descent based upon Hopf algebraic techniques.
Journal of Algebra,
Vol. 12,
Issue. 2,
p.
242.
Gr�nenfelder, Luzius A.
1970.
Hopf-Algebren und Coradikal.
Mathematische Zeitschrift,
Vol. 116,
Issue. 2,
p.
166.
Larson, Richard Gustavus
1971.
Characters of Hopf algebras.
Journal of Algebra,
Vol. 17,
Issue. 3,
p.
352.
Feinberg, Robert B.
1977.
Characterization of incidence algebras.
Discrete Mathematics,
Vol. 17,
Issue. 1,
p.
47.
Michaelis, Walter
1980.
Lie coalgebras.
Advances in Mathematics,
Vol. 38,
Issue. 1,
p.
1.
Chin, William
1992.
Crossed products of semisimple cocommutative Hopf algebras.
Proceedings of the American Mathematical Society,
Vol. 116,
Issue. 2,
p.
321.
Grossman, R.L.
and
Larson, R.G.
1992.
Viewing hybrid systems as products of control systems and automata.
p.
2953.
Artamonov, V. A.
1994.
The structure of Hopf algebras.
Journal of Mathematical Sciences,
Vol. 71,
Issue. 2,
p.
2289.
Montgomery, Susan
and
Witherspoon, S.J.
1998.
Irreducible representations of crossed products.
Journal of Pure and Applied Algebra,
Vol. 129,
Issue. 3,
p.
315.
He, Ji-Wei
Van Oystaeyen, Fred
and
Zhang, Yinhuo
2010.
Cocommutative Calabi–Yau Hopf algebras and deformations.
Journal of Algebra,
Vol. 324,
Issue. 8,
p.
1921.