Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-16T00:27:59.892Z Has data issue: false hasContentIssue false
  • English
  • Français

A New Look at the Kummer Surface

Published online by Cambridge University Press:  20 November 2018

W. L. Edge
W. L. Edge*
Affiliation:
Minto House, Chambers Street, Edinburgh 1, Scotland
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

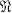Kummer's surface has the base surface F of a certain net of quadrics in [5] for a non-singular model. All the quadrics of 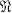have a common self-polar simplex ∑, and 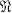can, in a double-infinity of ways, be based on a quadric Ω1 and two quadrics that Ω1 reciprocates into each other. F is invariant under harmonic inversions in the vertices and opposite bounding primes of ∑ and (§2) contains 32 lines. In §3 it is shown, conversely, that those quadrics for which a given simplex is self-polar and which contain a line of general position constitute a net of this kind.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 19 , 1967 , pp. 952 - 967
Copyright
Copyright © Canadian Mathematical Society 1967

References

1. Baker, H. F., Elementary note on the Weddle guartic surface, Proc. London Math. Soc. (2), 1 (1903), 247–261.Google Scholar
2. Baker, H. F., Multiply periodic functions (Cambridge, 1907).Google Scholar
3. Baker, H. F., Principles of geometry, Vol. IV (Cambridge, 1925 and 1940).Google Scholar
4. Baker, H. F., Principles of geometry, Vol. V (Cambridge, 1933).Google Scholar
5. Baker, H. F., Principles of geometry, Vol. VI (Cambridge, 1933).Google Scholar
6. Darboux, G., Sur la surface à seize points singuliers et les fonctions θ à deux variables, Comptes Rendus, 92 (1881), 685–688.Google Scholar
7. Edge, W. L., Humbert's plane sextics of genus 5, Proc. Cambridge Philos. Soc., 47 (1951), 483–495.Google Scholar
8. Edge, W. L., Baker's property of the Weddle surface, J. London Math. Soc., 32 (1957), 463–466.Google Scholar
9. Enriques, F. and Chisini, O., Teoria geometrica delle equazioni e delle funzioni algebriche, Vol. III (Bologna, 1924).Google Scholar
10. Hudson, R. W. H. T., Rummer's quartic surface (Cambridge, 1905).Google Scholar
11. Klein, F., Zur Theorie der Linienkomplexe des ersten und zweiten Grades, Math. Ann. 2 (1870), 198–226 ;Gesammelte Math. Werke, Vol. I (Berlin, 1921), pp. 53-80.Google Scholar
12. Plücker, J., Neue Geometrie des Raumes (Leipzig, 1868).Google Scholar
13. Reiss, M., Analytisch-geometrische Studien, Math. Ann. 2 (1870), 385–426.Google Scholar
14. Reye, T., Geometrie der Lage, Vol. III (Leipzig, 1892).Google Scholar
15. Zeuthen, H. G.. Lehrbuch der abzählenden Methoden in der Geometrie (Leipzig, 1914).Google Scholar