Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Lizama, Carlos
Miana, Pedro J.
Ponce, Rodrigo
and
Sánchez-Lajusticia, Luis
2014.
On the boundedness of generalized Cesàro operators on Sobolev spaces.
Journal of Mathematical Analysis and Applications,
Vol. 419,
Issue. 1,
p.
373.
Arvanitidis, Athanasios G.
2015.
Semigroups of composition operators on Hardy spaces of the half-plane.
Acta Scientiarum Mathematicarum,
Vol. 81,
Issue. 1-2,
p.
293.
Bonyo, J.O.
2017.
Spectral analysis of certain groups of isometries on Hardy and Bergman spaces.
Journal of Mathematical Analysis and Applications,
Vol. 456,
Issue. 2,
p.
1470.
Abadias, Luciano
and
Miana, Pedro J.
2018.
Generalized Cesàro operators, fractional finite differences and Gamma functions.
Journal of Functional Analysis,
Vol. 274,
Issue. 5,
p.
1424.
Hung, Ha Duy
Ky, Luong Dang
and
Quang, Thai Thuan
2020.
Hausdorff operators on holomorphic Hardy spaces and applications.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 150,
Issue. 3,
p.
1095.
Han, Kai Kai
and
Wang, Mao Fa
2020.
Complex Symmetric C0-semigroups on A2(ℂ+).
Acta Mathematica Sinica, English Series,
Vol. 36,
Issue. 10,
p.
1171.
Mose, S. B.
and
Bonyo, J. O.
2020.
Weighted Composition Groups on the Little Bloch Space.
Journal of Function Spaces,
Vol. 2020,
Issue. ,
p.
1.
Galé, José E.
Miana, Pedro J.
and
Sánchez–Lajusticia, Luis
2021.
RKH spaces of Brownian type defined by Cesàro–Hardy operators.
Analysis and Mathematical Physics,
Vol. 11,
Issue. 3,
Mirotin, A. R.
2021.
Hausdorff Operators on Some Spaces of Holomorphic Functions on the Unit Disc.
Complex Analysis and Operator Theory,
Vol. 15,
Issue. 5,
Miana, Pedro J.
and
Oliva-Maza, Jesús
2021.
Integral operators on Sobolev–Lebesgue spaces.
Banach Journal of Mathematical Analysis,
Vol. 15,
Issue. 3,
Mirotin, A. R.
2022.
Generalized Hausdorff-Zhu Operators on Möbius Invariant Spaces.
Complex Analysis and Operator Theory,
Vol. 16,
Issue. 7,
OYUGİ, Effie
BONYO, Job
and
AMBOGO, David
2023.
Ces\`{a}ro-Type Operator on the Dirichlet Space of the Upper Half Plane.
Communications in Advanced Mathematical Sciences,
Vol. 6,
Issue. 3,
p.
128.
Liflyand, Elijah
and
Mirotin, Adolf
2024.
Hausdorff operators: problems and solutions.
Journal of Mathematical Sciences,
Gallardo-Gutiérrez, Eva A.
and
Partington, Jonathan R.
2024.
Insights on the Cesàro operator: shift semigroups and invariant subspaces.
Journal d'Analyse Mathématique,
Vol. 152,
Issue. 2,
p.
595.
 $$C\left( f \right)\left( Z \right)=\frac{1}{Z}\int_{0}^{Z}{f\left( \zeta \right)d}\zeta,$$
$$C\left( f \right)\left( Z \right)=\frac{1}{Z}\int_{0}^{Z}{f\left( \zeta \right)d}\zeta,$$ $\mathcal{T}$ on Hardy spaces of the upper half plane. We identify
$\mathcal{T}$ on Hardy spaces of the upper half plane. We identify 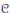 $\mathcal{C}$ and
$\mathcal{C}$ and  $\mathcal{T}$ as resolvents for appropriate semigroups of composition operators and we find the norm and the spectrum in each case. The relation of
$\mathcal{T}$ as resolvents for appropriate semigroups of composition operators and we find the norm and the spectrum in each case. The relation of 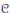 $\mathcal{C}$ and
$\mathcal{C}$ and  $\mathcal{T}$ with the corresponding Cesàro operators on Lebesgue spaces
$\mathcal{T}$ with the corresponding Cesàro operators on Lebesgue spaces  ${{L}^{p}}\left( \mathbb{R} \right)$ of the boundary line is also discussed.
${{L}^{p}}\left( \mathbb{R} \right)$ of the boundary line is also discussed.

