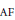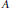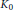Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Dean, Andrew J.
2006.
On Inductive Limit Type Actions of the Euclidean Motion Group on Stable UHF Algebras.
Canadian Mathematical Bulletin,
Vol. 49,
Issue. 2,
p.
213.
Dean, Andrew J.
2012.
Classification of Inductive Limits of Outer Actions of ℝ on Approximate Circle Algebras.
Canadian Mathematical Bulletin,
Vol. 55,
Issue. 1,
p.
73.