Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-10T17:33:42.686Z Has data issue: false hasContentIssue false
  • English
  • Français

On Homogeneous Expansions of Mixed Norm Space Functions in the Ball

Published online by Cambridge University Press:  20 November 2018

E. G. Kwon
E. G. Kwon*
Affiliation:
Department of Mathematics Education Andong National University Andong 760-749 Korea
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

For f analytic in the complex ball having the homogeneous expansion 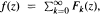conditions for f to be of Hardy space Hp or of weighted Bergman spaces are expressed in terms of lp properties of the sequence {∥Fk∥p}.

Keywords

32A3532A05homogeneous expansionHardy spacesmixed norm spaces
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 36 , Issue 1 , 01 March 1993 , pp. 78 - 86
Copyright
Copyright © Canadian Mathematical Society 1993

References

1. Ahern, P. and Bruna, J., Maximal and area characterization of Hardy-Sobolev spaces in the unit ball of Cn, Revista Mathemätica Iberoamericana 4( 1988), 123–153.Google Scholar
2. Ahern, P. and Bruna, J., On the holomorphicfunctions in the ball with absolutely continuous boundary values, Duke Math. J., to appearGoogle Scholar
3. Ahem, P. and W Rudin, Bloch functions, BMO, and boundary zeros, Indiana Univ. Math. J. 36(1987), 131–148.Google Scholar
4. Ahem, P. and W Rudin, Paley type gap theorems for Hp functions on the ball, Indiana Univ. Math. J. 37(1988), 255–275.Google Scholar
5. Alexander, H., Projective Capacity, Recent Developments in Several Complex Variables, Annals of Math. Studio 100,1981 Google Scholar
6. Beatrous, F. and Burbea, J., Holomorphic Sobolev spaces in the ball, Dissertationes Math. 276(1989), 1–57.Google Scholar
7. Duren, P. L., Theory of Hp spaces, Academic Press, New York, 1970 Google Scholar
8. Hardy, G. H. and Littlewood, J. E., Theorems concerning mean values of analytic functions or harmonic functions, Quart J. Math. Oxford Ser. 12(1941), 221–256.Google Scholar
9. Kellog, C. N., An extension of the Hausdorff-Young Theorem, Michigan Math. J. 8(1971), 121–127.Google Scholar
10. Kwak, Do Young, Hardy-Littlewood inequalities for weighted Bergman spaces, Communications of the Korean Mathematical Society 2(1987), 33–37.Google Scholar
11. Kwon, E. G., A note on the Taylor coefficients of mixed normed spaces, Bull. Austral. Math. Soc. 33(1986), 253–260.Google Scholar
12. Mateljevic’, M. and Pavlovic', M., LP -behavior of power series with positive coefficients and Hardy spaces, Proc. Amer. Math. Soc. 87(1983), 309–316.Google Scholar
13. Paley, R. E. A. C., On the lacunary coefficients of power series, Ann of Math 34(1933), 615–616.Google Scholar
14. Rudin, W., Function theory in the unit ball of Cn , Springer-Verlag, New York, 1980 Google Scholar
15. Rudin, W., New constructions of functions holomorphic in the unit ball of Cn , Conference Board of the Mathematical Science by AMS, 1985 Google Scholar
16. Ryll, J. and Wojtaszczyk, P., On homogeneous polynomials on a complex ball, Trans. Amer. Math. Soc. 276(1983),107–116.Google Scholar