Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-14T18:01:04.741Z Has data issue: false hasContentIssue false
  • English
  • Français

Rank k Vectors in Symmetry Classes of Tensors

Published online by Cambridge University Press:  20 November 2018

Ming-Huat Lim
Ming-Huat Lim*
Affiliation:
Department of Mathematics, University of Malaya, Kuala Lumpur, Malaysia
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let F be a field, G a subgroup of Sm, the symmetric group of degree m, and χ a linear character on G, i.e., a homomorphism of G into the multiplicative group of F. Let V1,...,Vm be vector spaces over F such that Vi = Vσ(i) for i=1,…,m and for all σ∈G. If W is a vector space over F, then a m-multilinear function is said to be symmetric with respect to G and χ if

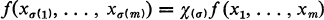
for any σ ∊ G and for arbitrary xi ∊ Vi.

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 19 , Issue 1 , 01 March 1976 , pp. 67 - 76
Copyright
Copyright © Canadian Mathematical Society 1976

Footnotes

✶

This paper arises from the author′s Ph.D. thesis written at the University of British Columbia under the supervision of Professor R. Westwick.

References

1. Boerner, H., Representations of groups (North Holland, Amsterdam, 1963).Google Scholar
2. Brawley, Joel Jr, On the ranks of bases of vector spaces of matrices, Lin. Alg. and App. 3 (1970), 51–55.Google Scholar
3. Chow, Wei-Liang, On the geometry ot algebraic homogeneous spaces, Ann. Math. 50 (1949), 32–67.Google Scholar
4. Cummings, L. J., Linear transformations of symmetric tensor spaces which preserve rank 1, Ph.D. thesis, University of British Columbia, 1967.Google Scholar
5. Cummings, L. J., Decomposable symmetric tensors, Pac. J. Math. 35 (1970), 65–77.Google Scholar
6. Greub, W. H., Multilinear algebra (Springer-Verlag, New York, 1967).Google Scholar
7. Hillel, Joel, Algebras of symmetry classes of tensors and their underlying permutation groups, J. Algebra 23 (1972), 215–227.Google Scholar
8. Lim, S. M. J., Rank k Grassmann products, Pac. J. Math. 29 (1969), 367–374.Google Scholar
9. Marcus, M., A theorem on rank with applications to mappings on symmetry classes of tensors, Bull. Amer. Math. Soc. 73 (1967), 675–677.Google Scholar
10. Marcus, M. and Gordon, W. R., Rational tensor representations of Hom(V, V) and an extension of an inequality of I. Schur, Can. J. Math. 26 (1972), 686–695.Google Scholar
11. Marcus, M. and Mine, H., Permutation on symmetry classes, J. Algebra 5 (1967), 59–71.Google Scholar
12. Singh, K., On the vanishing of a pure product in a (G, 6) space, Can. J. Math. 22 (1960), 363–371.Google Scholar
13. Westwick, R., A note on symmetry classes of tensors, J. Algebra 15 (1970), 309–311.Google Scholar
14. Westwick, R., Transformations on tensor spaces, Pac. J. Math. 14 (1967), 613–620.Google Scholar