Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-27T21:30:53.829Z Has data issue: false hasContentIssue false
  • English
  • Français

Similarité Entre Certains Quotients de A+ et des Quotients D'Algèbres Uniformes

Published online by Cambridge University Press:  20 November 2018

Konin Koua
Konin Koua*
Affiliation:
Laboratoire associé au CNRS n° 226, U.F.R. Mathématiques et Informatique, Université Bordeaux I, 351 cours de la Libération, 33405 Talence Cedex, France
Rights & Permissions [Opens in a new window]

Résumé

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let A(D) be the usual disc algebra, and let A+ be the subalgebra of A(D) consisting in those f € A(D) which have an absolutely convergent series of Taylor coefficients. Set m1 = {f € A(D) | f(1) = 0}, m = m1 ∩ A+, let G be the function 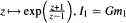and K = A+ ∩ I1.

We show that the quotient algebras m1/I1 and m/K are similar in the sense of J. Esterle.

Keywords

46J10
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 34 , Issue 4 , 01 December 1991 , pp. 485 - 491
Copyright
Copyright © Canadian Mathematical Society 1991

References

Références

1. Bennett, C. et Gilbert, J. E., Homogeneous algebras on the circle, ideals of analytic Junctions, Ann. Inst. Grenoble (3)22 (1972), 1–19.Google Scholar
2. Esterle, J., Quasimultipliers, representations of H°° and the closed ideal problem for commutative Banach algebras. Lecture Notes in Math. 975, 1983, 66–162.Google Scholar
3. Kahane, J. P., Séries de Fourier absolument convergentes. Springer-Verlag, Berlin-Heidelberg-New York, 1970.Google Scholar
4. Kahane, J. P., Idéaux primaires fermés dans certaines algèbres de Banach de fonctions analytiques. Lecture Notes in Math. 336, 1973, 5–14.Google Scholar
5. Koua, K., Similarité entre l'algèbre de Volterra et un quotient d'algèbre uniforme, (à paraître).Google Scholar
6. Strouse, E., Closed ideals in convolution algebras and the Laplace transform, Michigan J. 35(1988), 185– 196.Google Scholar