Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-10T19:34:37.883Z Has data issue: false hasContentIssue false
  • English
  • Français

A System of Operator Equations

Published online by Cambridge University Press:  20 November 2018

Bojan Magajna
Bojan Magajna*
Affiliation:
Department of Mathematics University of Ljubljana Jadranska 19, Ljubljana 61000, Yugoslavia
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 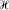be a separable Hilbert space, the algebra of all bounded operators on 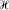and Ai, Bi, ∊ , i = 1,. . . , r. It is shown that if no nontrivial linear combination of the operators Ai, is compact, then there exist X, Y ∊ such that X Ai, Y = Bi, for all i. A related (but much milder) result is discussed in other algebras with the unique maximal ideal and an application to elementary operators is given.

Keywords

Primary 47A62Secondary 16A53
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 30 , Issue 2 , 01 June 1987 , pp. 200 - 209
Copyright
Copyright © Canadian Mathematical Society 1987

References

1. Apostol, C., Fialkow, L., Structure properties of elementary operators, Preprint.Google Scholar
2. Calkin, W., Two sided ideals and congruences in the ring of bounded operators in Hubert space, Ann. of Math. 42(1941), 839–873.Google Scholar
3. Caradus, S.R., Pfaffenberger, W.E., Yood, B., Calkin algebras and algebras of operators on Banach spaces, Marcel Dekker, Inc., New York, 1974.Google Scholar
4. Fialkow, L., Loebl, R., Elementary mappings into ideals of operators, Illinois J. Math. 28 (1984), 555–578.Google Scholar
5. Fillmore, P.A., Stampfli, J.G., Williams, J.P., On the essential numerical range, the essential spectrum, and a problem ofHalmos, Acta Sci. Math. (Szeged) 33 (1972), 179–192.Google Scholar
6. Fong, C.K., Sourour, A.R., On the operator identity ∑AK X BK=0, Can. J. Math. 31 (1979), 845–857.Google Scholar
7. Gramsh, B., Eine Idealstruktur Banachscher Operator-algebren, J. Reine Angew. Math. 225 (1967), 97–115.Google Scholar
8. Jacobson, N., Structure of rings, Amer. Math. Soc. Colloq. Publ. 37, Providence, Rhode Island, 1956.Google Scholar
9. Pierce, R.S., Associative algebras, Graduate Texts in Math. 88, Springer, New York, 1982.Google Scholar
10. Takesaki, M., Theory of operator algebras I, Springer-Verlag, New York, 1979.Google Scholar