No CrossRef data available.
Article contents
Adding Edges to Increase the Chromatic Number of a Graph
Part of:
Graph theory
Published online by Cambridge University Press: 31 March 2016
Abstract
If n ⩾ k + 1 and G is a connected n-vertex graph, then one can add 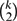 $\binom{k}{2}$ edges to G so that the resulting graph contains the complete graph K k+1. This yields that for any connected graph G with at least k + 1 vertices, one can add
$\binom{k}{2}$ edges to G so that the resulting graph contains the complete graph K k+1. This yields that for any connected graph G with at least k + 1 vertices, one can add 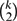 $\binom{k}{2}$ edges to G so that the resulting graph has chromatic number > k. A long time ago, Bollobás suggested that for every k ⩾ 3 there exists a k-chromatic graph Gk such that after adding to it any
$\binom{k}{2}$ edges to G so that the resulting graph has chromatic number > k. A long time ago, Bollobás suggested that for every k ⩾ 3 there exists a k-chromatic graph Gk such that after adding to it any 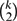 $\binom{k}{2}$ − 1 edges, the chromatic number of the resulting graph is still k. In this note we prove this conjecture.
$\binom{k}{2}$ − 1 edges, the chromatic number of the resulting graph is still k. In this note we prove this conjecture.
MSC classification
Secondary:
05C35: Extremal problems
Information
- Type
- Paper
- Information
- Copyright
- Copyright © Cambridge University Press 2016
References
[2]
Descartes, B. (1954) Solution to advanced problem No 4526. Amer. Math. Monthly
61
532.Google Scholar
[3]
Kostochka, A. and Nešetřil, J. (1999) Properties of Descartes' construction of triangle-free graphs with high chromatic number.
Combin. Probab. Comput.
8
467–472.CrossRefGoogle Scholar
[4]
Nešetřil, J. (2013) A combinatorial classic: Sparse graphs with high chromatic number. In Erdős Centennial, Springer, pp. 383–407.CrossRefGoogle Scholar

