Contents
Paper
WDM and Directed Star Arboricity
-
- Published online by Cambridge University Press:
- 05 February 2010, pp. 161-182
-
- Article
- Export citation
Application of a Generalization of Russo's Formula to Learning from Multiple Random Oracles
-
- Published online by Cambridge University Press:
- 09 July 2009, pp. 183-199
-
- Article
- Export citation
Random Sampling of Plane Partitions
-
- Published online by Cambridge University Press:
- 02 November 2009, pp. 201-226
-
- Article
- Export citation
Graph Partitioning via Adaptive Spectral Techniques
-
- Published online by Cambridge University Press:
- 13 November 2009, pp. 227-284
-
- Article
- Export citation
Directed Graphs Without Short Cycles
-
- Published online by Cambridge University Press:
- 01 October 2009, pp. 285-301
-
- Article
- Export citation
Identities and Inequalities for Tree Entropy
-
- Published online by Cambridge University Press:
- 15 December 2009, pp. 303-313
-
- Article
- Export citation
The Number of Independent Sets in a Regular Graph
-
- Published online by Cambridge University Press:
- 13 November 2009, pp. 315-320
-
- Article
- Export citation
Front Cover (OFC, IFC) and matter
CPC volume 19 issue 2 Cover and Front matter
-
- Published online by Cambridge University Press:
- 05 February 2010, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back Cover (IBC, OBC) and matter
CPC volume 19 issue 2 Cover and Back matter
-
- Published online by Cambridge University Press:
- 05 February 2010, pp. b1-b2
-
- Article
-
- You have access
- Export citation

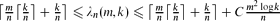 for some constant
for some constant