Refine listing
Actions for selected content:
Contents
ARTICLES
ON THE CONDITIONAL LIKELIHOOD RATIO TEST FOR SEVERAL PARAMETERS IN IV REGRESSION
-
- Published online by Cambridge University Press:
- 01 April 2009, pp. 305-335
-
- Article
- Export citation
ASYMPTOTIC THEORY FOR A FACTOR GARCH MODEL
-
- Published online by Cambridge University Press:
- 01 April 2009, pp. 336-363
-
- Article
- Export citation
FIRST-ORDER ASYMPTOTIC THEORY FOR PARAMETRIC MISSPECIFICATION TESTS OF GARCH MODELS
-
- Published online by Cambridge University Press:
- 01 April 2009, pp. 364-410
-
- Article
- Export citation
ON DISTINGUISHING BETWEEN RANDOM WALK AND CHANGE IN THE MEAN ALTERNATIVES
-
- Published online by Cambridge University Press:
- 01 April 2009, pp. 411-441
-
- Article
- Export citation
NONPARAMETRIC ADDITIVE MODELS FOR PANELS OF TIME SERIES
-
- Published online by Cambridge University Press:
- 01 April 2009, pp. 442-481
-
- Article
- Export citation
LIMIT THEORY FOR COINTEGRATED SYSTEMS WITH MODERATELY INTEGRATED AND MODERATELY EXPLOSIVE REGRESSORS
-
- Published online by Cambridge University Press:
- 01 April 2009, pp. 482-526
-
- Article
- Export citation
REGRESSION-BASED SEASONAL UNIT ROOT TESTS
-
- Published online by Cambridge University Press:
- 01 April 2009, pp. 527-560
-
- Article
- Export citation
Notes and Problems
QUASI-MAXIMUM LIKELIHOOD ESTIMATION OF SEMI-STRONG GARCH MODELS
-
- Published online by Cambridge University Press:
- 01 April 2009, pp. 561-570
-
- Article
- Export citation
ALMOST SURE BOUNDS ON THE ESTIMATION ERROR FOR OLS ESTIMATORS WHEN THE REGRESSORS INCLUDE CERTAIN MFI(1) PROCESSES
-
- Published online by Cambridge University Press:
- 01 April 2009, pp. 571-582
-
- Article
- Export citation
Front Cover (OFC, IFC) and matter
ECT volume 25 issue 2 Cover and Front matter
-
- Published online by Cambridge University Press:
- 03 March 2009, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back Cover (OBC, IBC) and matter
ECT volume 25 issue 2 Cover and Back matter
-
- Published online by Cambridge University Press:
- 03 March 2009, pp. b1-b3
-
- Article
-
- You have access
- Export citation

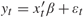 ,
, 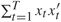 tends to infinity (a.s.) and log(
tends to infinity (a.s.) and log(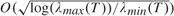 . In this note
. In this note