Article contents
Analytic continuation of holonomy germs of Riccati foliations along Brownian paths
Published online by Cambridge University Press: 11 April 2016
Abstract
Consider a Riccati foliation whose monodromy representation is non-elementary and parabolic and consider a non-invariant section of the fibration whose associated developing map is onto. We prove that any holonomy germ from any non-invariant fibre to the section can be analytically continued along a generic Brownian path. To prove this theorem, we prove a dual result about complex projective structures. Let  $\unicode[STIX]{x1D6F4}$ be a hyperbolic Riemann surface of finite type endowed with a branched complex projective structure: such a structure gives rise to a non-constant holomorphic map
$\unicode[STIX]{x1D6F4}$ be a hyperbolic Riemann surface of finite type endowed with a branched complex projective structure: such a structure gives rise to a non-constant holomorphic map  ${\mathcal{D}}:\tilde{\unicode[STIX]{x1D6F4}}\rightarrow \mathbb{C}\mathbb{P}^{1}$ , from the universal cover of
${\mathcal{D}}:\tilde{\unicode[STIX]{x1D6F4}}\rightarrow \mathbb{C}\mathbb{P}^{1}$ , from the universal cover of  $\unicode[STIX]{x1D6F4}$ to the Riemann sphere
$\unicode[STIX]{x1D6F4}$ to the Riemann sphere 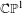 $\mathbb{C}\mathbb{P}^{1}$ , which is
$\mathbb{C}\mathbb{P}^{1}$ , which is  $\unicode[STIX]{x1D70C}$ -equivariant for a morphism
$\unicode[STIX]{x1D70C}$ -equivariant for a morphism 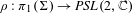 $\unicode[STIX]{x1D70C}:\unicode[STIX]{x1D70B}_{1}(\unicode[STIX]{x1D6F4})\rightarrow \mathit{PSL}(2,\mathbb{C})$ . The dual result is the following. If the monodromy representation
$\unicode[STIX]{x1D70C}:\unicode[STIX]{x1D70B}_{1}(\unicode[STIX]{x1D6F4})\rightarrow \mathit{PSL}(2,\mathbb{C})$ . The dual result is the following. If the monodromy representation  $\unicode[STIX]{x1D70C}$ is parabolic and non-elementary and if
$\unicode[STIX]{x1D70C}$ is parabolic and non-elementary and if  ${\mathcal{D}}$ is onto, then, for almost every Brownian path
${\mathcal{D}}$ is onto, then, for almost every Brownian path  $\unicode[STIX]{x1D714}$ in
$\unicode[STIX]{x1D714}$ in 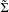 $\tilde{\unicode[STIX]{x1D6F4}}$ ,
$\tilde{\unicode[STIX]{x1D6F4}}$ ,  ${\mathcal{D}}(\unicode[STIX]{x1D714}(t))$ does not have limit when
${\mathcal{D}}(\unicode[STIX]{x1D714}(t))$ does not have limit when  $t$ goes to
$t$ goes to 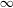 $\infty$ . If, moreover, the projective structure is of parabolic type, we also prove that, although
$\infty$ . If, moreover, the projective structure is of parabolic type, we also prove that, although 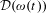 ${\mathcal{D}}(\unicode[STIX]{x1D714}(t))$ does not converge, it converges in the Cesàro sense.
${\mathcal{D}}(\unicode[STIX]{x1D714}(t))$ does not converge, it converges in the Cesàro sense.
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press, 2016
References
- 1
- Cited by

