Article contents
Non-divergence of unipotent flows on quotients of rank-one semisimple groups
Published online by Cambridge University Press: 28 December 2015
Abstract
Let 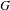 $G$ be a semisimple Lie group of rank one and
$G$ be a semisimple Lie group of rank one and 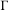 $\unicode[STIX]{x1D6E4}$ be a torsion-free discrete subgroup of
$\unicode[STIX]{x1D6E4}$ be a torsion-free discrete subgroup of 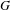 $G$ . We show that in
$G$ . We show that in 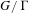 $G/\unicode[STIX]{x1D6E4}$ , given
$G/\unicode[STIX]{x1D6E4}$ , given 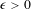 $\unicode[STIX]{x1D716}>0$ , any trajectory of a unipotent flow remains in the set of points with injectivity radius larger than
$\unicode[STIX]{x1D716}>0$ , any trajectory of a unipotent flow remains in the set of points with injectivity radius larger than  $\unicode[STIX]{x1D6FF}$ for a
$\unicode[STIX]{x1D6FF}$ for a 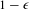 $1-\unicode[STIX]{x1D716}$ proportion of the time, for some
$1-\unicode[STIX]{x1D716}$ proportion of the time, for some 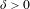 $\unicode[STIX]{x1D6FF}>0$ . The result also holds for any finitely generated discrete subgroup
$\unicode[STIX]{x1D6FF}>0$ . The result also holds for any finitely generated discrete subgroup 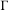 $\unicode[STIX]{x1D6E4}$ and this generalizes Dani’s quantitative non-divergence theorem [On orbits of unipotent flows on homogeneous spaces. Ergod. Th. & Dynam. Sys.4(1) (1984), 25–34] for lattices of rank-one semisimple groups. Furthermore, for a fixed
$\unicode[STIX]{x1D6E4}$ and this generalizes Dani’s quantitative non-divergence theorem [On orbits of unipotent flows on homogeneous spaces. Ergod. Th. & Dynam. Sys.4(1) (1984), 25–34] for lattices of rank-one semisimple groups. Furthermore, for a fixed 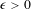 $\unicode[STIX]{x1D716}>0$ , there exists an injectivity radius
$\unicode[STIX]{x1D716}>0$ , there exists an injectivity radius  $\unicode[STIX]{x1D6FF}$ such that, for any unipotent trajectory
$\unicode[STIX]{x1D6FF}$ such that, for any unipotent trajectory 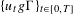 $\{u_{t}g\unicode[STIX]{x1D6E4}\}_{t\in [0,T]}$ , either it spends at least a
$\{u_{t}g\unicode[STIX]{x1D6E4}\}_{t\in [0,T]}$ , either it spends at least a 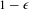 $1-\unicode[STIX]{x1D716}$ proportion of the time in the set with injectivity radius larger than
$1-\unicode[STIX]{x1D716}$ proportion of the time in the set with injectivity radius larger than  $\unicode[STIX]{x1D6FF}$ , for all large
$\unicode[STIX]{x1D6FF}$ , for all large 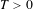 $T>0$ , or there exists a
$T>0$ , or there exists a 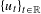 $\{u_{t}\}_{t\in \mathbb{R}}$ -normalized abelian subgroup
$\{u_{t}\}_{t\in \mathbb{R}}$ -normalized abelian subgroup  $L$ of
$L$ of 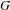 $G$ which intersects
$G$ which intersects 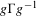 $g\unicode[STIX]{x1D6E4}g^{-1}$ in a small covolume lattice. We also extend these results to when
$g\unicode[STIX]{x1D6E4}g^{-1}$ in a small covolume lattice. We also extend these results to when  $G$ is the product of rank-one semisimple groups and
$G$ is the product of rank-one semisimple groups and 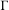 $\unicode[STIX]{x1D6E4}$ a discrete subgroup of
$\unicode[STIX]{x1D6E4}$ a discrete subgroup of  $G$ whose projection onto each non-trivial factor is torsion free.
$G$ whose projection onto each non-trivial factor is torsion free.
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press, 2015
References
- 4
- Cited by

