Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-13T06:06:14.871Z Has data issue: false hasContentIssue false
  • English
  • Français

On the distribution of free path lengths for the periodic Lorentz gas II

Published online by Cambridge University Press:  15 April 2002

François Golse  and
Bernt Wennberg
François Golse
Affiliation:
École Normale Supérieure, D.M.A., 45 rue d'Ulm, 75230 Paris Cedex 05, France. e-mail: Francois.Golse@ens.fr Institut Universitaire de France and Université Paris VII, France.
Bernt Wennberg
Affiliation:
Chalmers University of Technology, Dept. of Mathematics, 41296 Göteborg, Sweden.
Get access

Abstract

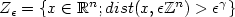Consider the domain $Z_\epsilon=\{x\in\mathbb{R}^n ; {dist}(x,\epsilon\mathbb{Z}^n)> \epsilon^\gamma\}$ and let the free path length be defined as $\tau_\epsilon(x,v)=\inf\{t> 0 ; x-tv\in Z_\epsilon\}.$ 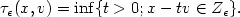In the Boltzmann-Grad scaling corresponding to $\gamma=\frac{n}{n-1}$ 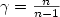, it is shownthat the limiting distribution $\phi_\epsilon$ 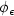of $\tau_\epsilon$ 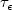is bounded from belowby an expression of the form C/t, for some C> 0. A numerical study seems toindicate that asymptotically for large t, $\phi_\epsilon\sim C/t$ 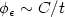.This is an extension of a previous work [J. Bourgain et al., Comm. Math. Phys.190 (1998) 491-508]. As aconsequence, it is proved that the linear Boltzmann type transport equation is inappropriate to describethe Boltzmann-Grad limit of the periodic Lorentz gas, at variance with the usualcase of a Poisson distribution of scatterers treated in [G. Gallavotti (1972)].

Keywords

Lorentz gasBoltzmann-Grad limitkinetic theorymean free path.
Type
Research Article
Information
ESAIM: Mathematical Modelling and Numerical Analysis , Volume 34 , Issue 6 , November 2000 , pp. 1151 - 1163
Copyright
© EDP Sciences, SMAI, 2000

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Boldrighini, C., Bunimovich, L.A. and Sinai, Ya.G., On the Boltzmann equation for the Lorentz gas. J. Statist. Phys. 32 (1983) 477-501. CrossRef
Bourgain, J., Golse, F. and Wennberg, B., On the distribution of free path lengths for the periodic Lorentz gas. Comm. Math. Phys. 190 (1998) 491-508. CrossRef
Bunimovich, L.A. and Sinai, Ya.G., Markov Partitions of Dispersed Billiards. Comm. Math. Phys. 73 (1980) 247-280. CrossRef
Bunimovich, L.A. and Sinai, Ya.G., Statistical properties of the Lorentz gas with periodic configurations of scatterers. Comm. Math. Phys. 78 (1981) 479-497. CrossRef
Bunimovich, L.A., Sinai, Ya.G. and Chernov, N.I., Markov partitions for two-dimensional hyperbolic billiards. Russian Math. Surveys 45 (1990) 105-152. CrossRef
Bunimovich, L.A., Sinai, Ya.G. and Chernov, N.I., Statistical properties of two-dimensional hyperbolic billiards. Russian Math. Surveys 46 (1991) 47-106. CrossRef
Dumas, H.S., Dumas, L. and Golse, F., Remarks on the notion of mean free path for a periodic array of spherical obstacles. J. Statist. Phys. 87 (1997) 943-950. CrossRef
G. Gallavotti, Rigorous theory of the Boltzmann equation in the Lorentz gas. Nota Interna No. 358, Istituto di Fisica, Università di Roma (1972).
Golse, F., Transport dans les milieux composites fortement contrastés. I. Le modèle du billard. Ann. Inst. H. Poincaré Phys. Théor. 61 (1994) 381-410.
Spohn, H., The Lorentz flight process converges to a random flight process. Comm. Math. Phys. 60 (1978) 277-290. CrossRef