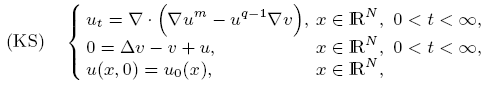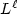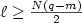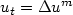Refine listing
Actions for selected content:
Contents
Research Article
Numerical analysis of Eulerian multi-fluid models in the contextof kinetic formulations for dilute evaporating sprays
-
- Published online by Cambridge University Press:
- 22 July 2006, pp. 431-468
-
- Article
- Export citation
Adaptive modeling for free-surface flows
-
- Published online by Cambridge University Press:
- 22 July 2006, pp. 469-499
-
- Article
- Export citation
On a stabilized colocated Finite Volume scheme for the Stokes problem
-
- Published online by Cambridge University Press:
- 22 July 2006, pp. 501-527
-
- Article
- Export citation
A reduced basis element methodfor the steady Stokes problem
-
- Published online by Cambridge University Press:
- 22 July 2006, pp. 529-552
-
- Article
- Export citation
Analysis of crack singularities in an aging elastic material
-
- Published online by Cambridge University Press:
- 22 July 2006, pp. 553-595
-
- Article
- Export citation
Large time behavior of solutions in super-critical cases to degenerate Keller-Segel systems
-
- Published online by Cambridge University Press:
- 22 July 2006, pp. 597-621
-
- Article
- Export citation