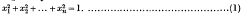Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Rankin, R. A.
1955.
On Packings of Spheres in Hilbert Space.
Proceedings of the Glasgow Mathematical Association,
Vol. 2,
Issue. 3,
p.
145.
Rankin, R. A.
1956.
On the minimal points of positive definite quadratic forms.
Mathematika,
Vol. 3,
Issue. 1,
p.
15.
Flores, I.
and
Grey, L.
1960.
Optimization of Reference Signals for Character Recognition Systems.
IEEE Transactions on Electronic Computers,
Vol. EC-9,
Issue. 1,
p.
54.
Blachman, N.
1962.
On the capacity of a band-limited channel perturbed by statistically dependent interference.
IEEE Transactions on Information Theory,
Vol. 8,
Issue. 1,
p.
48.
Blachman, N.
1962.
The effect of statistically dependent interference upon channel capacity.
IEEE Transactions on Information Theory,
Vol. 8,
Issue. 5,
p.
53.
Grey, L.
1962.
Some bounds for error-correcting codes.
IEEE Transactions on Information Theory,
Vol. 8,
Issue. 3,
p.
200.
Blachman, Nelson M.
Goldberg, Michael
Zame, Alan
DeMarr, Ralph
and
Robinson, D. A.
1963.
Mathematical Notes.
The American Mathematical Monthly,
Vol. 70,
Issue. 5,
p.
526.
Blachman, N. M.
and
Few, L.
1963.
Multiple packing of spherical caps.
Mathematika,
Vol. 10,
Issue. 1,
p.
84.
Grey, Louis D.
1963.
Another Satellite Communications Problem (Isidore Silberman).
SIAM Review,
Vol. 5,
Issue. 3,
p.
287.
Rankin, R. A.
1964.
On the minimal points of perfect quadratic forms.
Mathematische Zeitschrift,
Vol. 84,
Issue. 3,
p.
228.
Wyner, A. D.
1964.
An Improved Error Bound for Gaussian Channels.
Bell System Technical Journal,
Vol. 43,
Issue. 6,
p.
3070.
Wyner, A. D.
1965.
Capabilities of Bounded Discrepancy Decoding.
Bell System Technical Journal,
Vol. 44,
Issue. 6,
p.
1061.
1969.
Geometry of Numbers.
p.
471.
Wyner, A. D.
1969.
On Cading and Information Theory.
SIAM Review,
Vol. 11,
Issue. 3,
p.
317.
Davidson, Rollo
1970.
Sorting vectors.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 68,
Issue. 1,
p.
153.
Larman, D. G.
and
Rogers, C. A.
1972.
The realization of distances within sets in Euclidean space.
Mathematika,
Vol. 19,
Issue. 1,
p.
1.
Moore, Michael H.
1974.
An improved upper bound in the maximum dispersal problem.
Linear Algebra and its Applications,
Vol. 8,
Issue. 5,
p.
471.
Moore, Michael H.
1974.
Vector packing in finite dimensional vector spaces.
Linear Algebra and its Applications,
Vol. 8,
Issue. 3,
p.
213.
Saaty, Thomas L.
and
Alexander, Joyce M.
1975.
Optimization and the Geometry of Numbers: Packing and Covering.
SIAM Review,
Vol. 17,
Issue. 3,
p.
475.
Levenshtein, V. I.
1975.
Maximal packing density of n-dimensional Euclidean space with equal balls.
Mathematical Notes of the Academy of Sciences of the USSR,
Vol. 18,
Issue. 2,
p.
765.