Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-15T12:02:40.610Z Has data issue: false hasContentIssue false
  • English
  • Français

Generation of the lower central series II

Published online by Cambridge University Press:  18 May 2009

Robert M. Guralnick
Robert M. Guralnick
Affiliation:
Department of Mathematics, University of Southern California, Los Angeles California 90089-1113, U.S.A.
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this article, we obtain results on commutators in Sylow subgroups of the lower central series, extending the work of Dark and Newell [2], Rodney [12, 13] and Aschbacher and the author [1, 6, 7].

Some notation is required for the statement of the main results. Let r be a positive integer and define

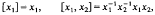
and

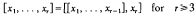
where x1, …, xr, are elements in a group G. Let ΓrG = {[x1, …, xr]∣ x1 ∈ G} be the set of r-fold commutators in G. Then Lr,G = 〈ΓrG〉 is the rth term in the lower central series of G. Set L∞G = ∩ Lr,G.

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 25 , Issue 2 , July 1984 , pp. 193 - 201
Copyright
Copyright © Glasgow Mathematical Journal Trust 1984

References

REFERENCES

1.Aschbacher, M. and Guralnick, R., Solvable generation of groups and Sylow subgroups of the lower central series, J. Algebra 77 (1982), 189–201.CrossRefGoogle Scholar
2.Dark, R. S. and Newell, M. L., On conditions for commutators to form a subgroup, J. London Math. Soc. (2) 17 (1978), 251–262.Google Scholar
3.Gallagher, P. X., The generation of the lower central series, Canad. J. Math. 17 (1965), 405–410.Google Scholar
4.Gorenstein, D., Finite groups (Harper and Row, 1968).Google Scholar
5.Guralnick, R., On a result of Schur, J. Algebra 59 (1979), 302–309.CrossRefGoogle Scholar
6.Guralnick, R., Generation of the lower central series, Glasgow Math. J. 23 (1982), 15–20.CrossRefGoogle Scholar
7.Guralnick, R., Commutators and commutator subgroups, Adv. in Math. 45 (1982), 319–330.CrossRefGoogle Scholar
8.Isaacs, I. M., Commutators and the commutator subgroup, Amer. Math. Monthly 84 (1977), 720–722.Google Scholar
9.Kappe, L.-C., Groups with a cyclic term in the lower central series, Arch. Math. (Basel) 30 (1978), 561–569.Google Scholar
10.Liebeck, H., A test for commutators, Glasgow Math. J. 17 (1976), 31–36.CrossRefGoogle Scholar
11.Macdonald, I. D., On cyclic commutator subgroups, J. London Math. Soc. 38 (1963), 419–422.CrossRefGoogle Scholar
12.Rodney, D. M., On cyclic derived subgroups, J. London Math. Soc. (2) 8 (1974), 642–646.Google Scholar
13.Rodney, D. M., Commutators and abelian groups, J. Austral. Math. Soc. Ser. A 24 (1977), 79–91.Google Scholar