Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-15T09:53:43.448Z Has data issue: false hasContentIssue false
  • English
  • Français

Kurosh's chains of associative rings

Published online by Cambridge University Press:  18 May 2009

R. R. Andruszkiewicz  and
E. R. Puczylowski
R. R. Andruszkiewicz
Affiliation:
Institute of Mathematics University of Warsaw, Bialystok Division Akademicka 2, 15-267 BialystokPoland
E. R. Puczylowski
Affiliation:
Institute of MathematicsUniversity of Warsaw Pkin, 00-901 WarsawPoland
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let N be a homomorphically closed class of associative rings. Put N1 = Nl = N and, for ordinals a ≥ 2, define Nα (Nα) to be the class of all associative rings R such that every non-zero homomorphic image of R contains a non-zero ideal (left ideal) in Nβ for some β<α. In this way we obtain a chain {Nα} ({Nα}), the union of which is equal to the lower radical class IN (lower left strong radical class IsN) determined by N. The chain {Nα} is called Kurosh's chain of N. Suliński, Anderson and Divinsky proved [7] that 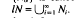. Heinicke [3] constructed an example of N for which lN ≠ Nk for k = 1, 2,. … In [1] Beidar solved the main problem in the area showing that for every natural number n ≥ 1 there exists a class N such that IN = Nn+l ≠ Nn. Some results concerning the termination of the chain {Nα} were obtained in [2,4]. In this paper we present some classes N with Nα = Nα for all α Using this and Beidar's example we prove that for every natural number n ≥ 1 there exists an N such that Nα = Nα for all α and Nn ≠ Nn+i = Nn+2. This in particular answers Question 6 of [4].

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 32 , Issue 1 , January 1990 , pp. 67 - 69
Copyright
Copyright © Glasgow Mathematical Journal Trust 1990

References

REFERENCES

1.Beidar, K. I., A chain of Kurosh may have an arbitrary finite length, Czech. Math. J. 32 (1982), 418–422.CrossRefGoogle Scholar
2.Divinsky, N., Krempa, J. and Suliński, A., Strong radical properties of alternative and associative rings, J. Algebra 17 (1971), 369–381.CrossRefGoogle Scholar
3.Heinicke, A., A note on lower radical constructions for associative rings, Canad. Math. Bull. 11 (1968), 23–30.CrossRefGoogle Scholar
4.Puczytowski, E. R., On questions concerning strong radicals of associative rings, Quaestiones Math. 10 (1987), 321–338.CrossRefGoogle Scholar
5.Sands, A. D., On M-nilpotent rings, Proc. Royal Soc. Edinburgh Sect. A 93 (1982), 63–70.CrossRefGoogle Scholar
6.Stewart, P. N., On the lower radical construction, Ada Math. Acad. Sci. Hungar. 25 (1974), 31–32.CrossRefGoogle Scholar
7.Suliński, A., Anderson, T. and Divinsky, N., Lower radical properties for associative and alternative rings, J. London Math. Soc 41 (1966), 417–424.CrossRefGoogle Scholar
8.Wiegandt, R., Radical and semisimple classes of rings, Queen's University, Kingston, Ontario, 1974.Google Scholar