Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
1978.
Classification Theory.
Vol. 92,
Issue. ,
p.
684.
Lascar, Daniel
1981.
Les modèles dénombrables d’une théorie ayant des fonctions de Skolem.
Transactions of the American Mathematical Society,
Vol. 268,
Issue. 2,
p.
345.
1986.
Logic Colloquium '84.
Vol. 120,
Issue. ,
p.
225.
Enayat, Ali
1988.
Undefinable classes and definable elements in models of set theory and arithmetic.
Proceedings of the American Mathematical Society,
Vol. 103,
Issue. 4,
p.
1216.
Knight, R. W.
2007.
Categories of Topological Spaces and Scattered Theories.
Notre Dame Journal of Formal Logic,
Vol. 48,
Issue. 1,
Mohsenipour, Shahram
2007.
A generalization of the Keisler‐Morley theorem to recursively saturated ordered structures.
Mathematical Logic Quarterly,
Vol. 53,
Issue. 3,
p.
289.
Enayat, Ali
and
Mohsenipour, Shahram
2008.
Model theory of the regularity and reflection schemes.
Archive for Mathematical Logic,
Vol. 47,
Issue. 5,
p.
447.
Tanović, Predrag
2010.
Types directed by constants.
Annals of Pure and Applied Logic,
Vol. 161,
Issue. 7,
p.
944.
Shelah, Saharon
2011.
Models of expansions of \documentclass{article}\usepackage{amssymb}\begin{document}\pagestyle{empty}${\mathbb N}$\end{document} with no end extensions.
Mathematical Logic Quarterly,
Vol. 57,
Issue. 4,
p.
341.
Mohsenipour, Shahram
2011.
Model theory of the inaccessibility scheme.
Archive for Mathematical Logic,
Vol. 50,
Issue. 7-8,
p.
697.
Baizhanov, Bektur S.
2016.
One-types in ordered theories.
Vol. 1759,
Issue. ,
p.
020073.
Malliaris, Maryanthe
and
Shelah, Saharon
2017.
Model-theoretic applications of cofinality spectrum problems.
Israel Journal of Mathematics,
Vol. 220,
Issue. 2,
p.
947.
Kurilić, Miloš S.
2019.
Vaught's conjecture for monomorphic theories.
Annals of Pure and Applied Logic,
Vol. 170,
Issue. 8,
p.
910.
Moconja, Slavko
and
Tanović, Predrag
2020.
Stationarily ordered types and the number of countable models.
Annals of Pure and Applied Logic,
Vol. 171,
Issue. 3,
p.
102765.
Clemens, John D.
and
Coskey, Samuel
2022.
New jump operators on equivalence relations.
Journal of Mathematical Logic,
Vol. 22,
Issue. 03,
Tanović, Predrag
2024.
Vaught’s Conjecture for Theories of Discretely Ordered Structures.
Notre Dame Journal of Formal Logic,
Vol. 65,
Issue. 3,

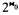 nonisomorphic countable models; and that if every model of
nonisomorphic countable models; and that if every model of 