Generalizations of the one-dimensional version of the Kruskal-Friedman theorems

Extract

The paper [Schütte + Simpson] deals with the following one-dimensional case of Friedman's extension (see in [Simpson 1]) of Kruskal's theorem ([Kruskal]). Given a natural number n, let S_n+1 be the set of all finite sequences of natural numbers <n + 1. If s₁ = (a₀,…,a_k) ∈S_n+1 and s₂ = (b₀,…,b_m) ∈S_{n + 1}, then a strictly monotone function f: {0,…, k} → {0,…, m} is called an embedding of s₁ into s₂ if the following two assertions are satisfied:

1) a_i, = b_f(i), for all i < k;

2) if f(i) < j < f(i + 1) then b_j > b_f(i+1), for all i < k, j < m.

Then for every infinite sequence s₁, s₂,…,s_k,… of elements of S_{n + 1} there exist indices i < j and an embedding of s_i into S_j. That is, S_n+1 forms a well-quasi-ordering (wqo) with respect to embeddability. For each n, this statement W(S_n+1) is provable in the standard second order conservative extension of Peano arithmetic. On the other hand, the proof-theoretic strength of the statements W(S_n+1) grows so fast that this formal theory cannot prove the limit statement ∀nW(S_n+1). The appropriate first order 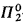-versions of these combinatory statements preserve their proof-theoretic strength, so that actually one can speak in terms of provability in Peano arithmetic. These are the main conclusions from [Schütte + Simpson].

We wish to extend this into the transfinite. That is, we take an arbitrary countable ordinal τ > 0 instead of n + 1 and try to obtain an analogous “strong” combinatory statement about finite sequences of ordinals < τ.