Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-10T22:35:28.631Z Has data issue: false hasContentIssue false
  • English
  • Français

Subgroups of small index in infinite symmetric groups. II

Published online by Cambridge University Press:  12 March 2014

Saharon Shelah  and
Simon Thomas
Saharon Shelah
Affiliation:
Institute of Mathematics, The Hebrew University, Jerusalem, Israel
Simon Thomas
Affiliation:
Department of Mathematics, Rutgers University, New Brunswick, New Jersey 08903
Get access Rights & Permissions [Opens in a new window]

Extract

Throughout this paper κ denotes an infinite cardinal, S = Sym(κ) and G is a subgroup of S. We shall be seeking the subgroups G with [S: G] < 2κ. In [2], the following result was proved.

Theorem 1. If [S: G] ≤ κthen there exists a subset Δ of k such that ∣Δ∣ < k and S(Δ) ≤ G.

Here S(Δ) = Sym(K/⊿) is the pointwise stabilizer of Δ in S.

However, the converse of Theorem 1 is not true. For if cf(κ) ≤ ∣Δ∣ < κ, then [S: S(Δ)] ≥ κcf(κ) > κ. This suggests that a substantially sharpened version of Theorem 1 may be true.

Question 1 [2]. Is it provable in ZFC, or even in ZFC with GCH, that if [S: G] ≤ κ then there is a subset Δ of κ such that ∣Δ∣ < cf(κ) and S(Δ) ≤ G?

At least two of the authors of [2] made a serious attempt to answer the above question positively. In §3, we shall see that they were essentially trying to prove that measurable cardinals do not exist.

The following result, due independently to Semmes [5] and Neumann [2], suggests a second way in which Theorem 1 might be improved.

Theorem 2. If k = ℵ0and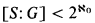then there is a finite subset Δ of k such thatS(Δ) ≤ G.

Question 2 [2]. Is it provable in ZFC that if [S: G] < 2κ then there is a subset Δ of κ such that ∣Δ∣ < κ and S(Δ) < G?

This question will be answered negatively in §4.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 54 , Issue 1 , March 1989 , pp. 95 - 99
Copyright
Copyright © Association for Symbolic Logic 1989

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Ben-David, S. and Magidor, M., The weak □* is really weaker than the full □, this Journal, vol. 51 (1986), pp. 1029–1033.Google Scholar
[2]Dixon, J. D., Neumann, P.M. and Thomas, S., Subgroups of small index in infinite symmetric groups, Bulletin of the London Mathematical Society, vol. 18 (1986), pp. 580–586.CrossRefGoogle Scholar
[3]Donder, H. D., Regularity and decomposability of uniform ultrafilters (to appear).Google Scholar
[4]Kunen, K., Set theory, North-Holland, Amsterdam, 1980.Google Scholar
[5]Semmes, S. W., Endomorphisms of infinite symmetric groups, Abstracts of Papers Presented to the American Mathematical Society, vol. 2 (1981), p. 426.Google Scholar
[6]Semmes, S. W., Infinite symmetric groups, maximal subgroups and filters, Abstracts of Papers Presented to the American Mathematical Society, vol. 3 (1982), p. 38.Google Scholar
[7]Williams, N. H., Combinatorial set theory, North-Holland, Amsterdam, 1977.Google Scholar