No CrossRef data available.
Published online by Cambridge University Press: 09 April 2009
Let B(H) be the Banach algebra of all (bounded linear) operators on an infinite-dimensional separable complex Hilbert space H and let 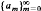 be a bounded sequence of positive real numbers. For a given injective operator A in B(H) and a non-zero vector f in H, we put
be a bounded sequence of positive real numbers. For a given injective operator A in B(H) and a non-zero vector f in H, we put 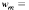
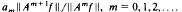 We define a weighted shift Tw with the weight sequence
We define a weighted shift Tw with the weight sequence 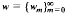 on the Hilbert space 12 of all square-summable complex sequences
on the Hilbert space 12 of all square-summable complex sequences 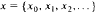 by
by 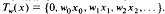 . The main object of this paper is to characterize the invariant subspace lattice of Tw under various nice conditions on the operator A and the sequence
. The main object of this paper is to characterize the invariant subspace lattice of Tw under various nice conditions on the operator A and the sequence 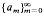 .
.