Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Chen, Yong-Zhuo
and
Lau, Ka-Sing
1989.
Some new classes of Hardy spaces.
Journal of Functional Analysis,
Vol. 84,
Issue. 2,
p.
255.
Shanzhen, Lu
and
Dachun, Yang
1995.
The central BMO spaces and Littlewood-Paley operators.
Approximation Theory and its Applications,
Vol. 11,
Issue. 3,
p.
72.
Yang, Dachun
and
Zhou, Yuan
2010.
Some new characterizations on spaces of functions with bounded mean oscillation.
Mathematische Nachrichten,
Vol. 283,
Issue. 4,
p.
588.
Izuki, Mitsuo
Sawano, Yoshihiro
and
Tsutsui, Yohei
2014.
Variable Lebesgue norm estimates for BMO functions. II.
Analysis Mathematica,
Vol. 40,
Issue. 3,
p.
215.

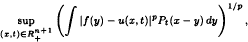
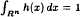 and
and 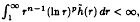 , where
, where 