Hostname: page-component-6bf8c574d5-86b6f Total loading time: 0 Render date: 2025-03-03T16:55:05.037Z Has data issue: false hasContentIssue false
  • English
  • Français

PROPER $\mathrm {CAT(0)}$ ACTIONS OF UNIPOTENT-FREE LINEAR GROUPS

Part of: Special aspects of infinite or finite groups

Published online by Cambridge University Press:  07 January 2025

Sami Douba Open the ORCID record for Sami Douba [Opens in a new window]
Sami Douba*
Affiliation:
Institut des Hautes Études Scientifiques, 35 route de Chartres, 91440 Bures-sur-Yvette, France
*
doubasami@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 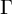$\Gamma $ be a finitely generated group of matrices over 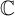$\mathbb {C}$. We construct an isometric action of 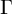$\Gamma $ on a complete 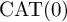$\mathrm {CAT}(0)$ space such that the restriction of this action to any subgroup of 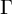$\Gamma $ containing no nontrivial unipotent elements is well behaved. As an application, we show that if M is a graph manifold that does not admit a nonpositively curved Riemannian metric, then any finite-dimensional 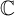$\mathbb {C}$-linear representation of 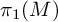$\pi _1(M)$ maps a nontrivial element of 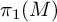$\pi _1(M)$ to a unipotent matrix. In particular, the fundamental groups of such 3-manifolds do not admit any faithful finite-dimensional unitary representations.

Keywords

geometric group theorygeometric topologylow-dimensional topology

MSC classification

Primary: 20F67: Hyperbolic groups and nonpositively curved groups
Secondary: 20F65: Geometric group theory
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 24 , Issue 2 , March 2025 , pp. 587 - 601
Check for updates
Copyright
© The Author(s), 2025. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The author was partially supported by the National Science Centre, Poland UMO-2018/30/M/ST1/00668

References

Agol, I (2018) Hyperbolic $3$ -Manifold Groups That Embed in Compact Lie Groups . MathOverflow. URL:https://mathoverflow.net/q/315430 (version: 2018-11-16).Google Scholar
Agol, I, Groves, D and Manning, J (2013) The virtual Haken conjecture. Doc. Math 18, 1045–1087.CrossRefGoogle Scholar
Alperin, RC and Shalen, PB (1982) Linear groups of finite cohomological dimension. Inventiones mathematicae 66, 89–98.CrossRefGoogle Scholar
Aschenbrenner, M, Friedl, A and Wilton, H (2015) 3-Manifold Groups , EMS Series of Lectures in Mathematics. Zürich: European Mathematical Society (EMS).Google Scholar
Bergeron, N and Wise, DT (2012) A boundary criterion for cubulation. Amer. J. Math. 134, 843–859.CrossRefGoogle Scholar
Bridson, MR (2001) On the subgroups of semihyperbolic groups. In Essays on Geometry and Related Topics, vol. 1, 2, vol. 38 of Monogr. Enseign. Math. Geneva: Enseignement Math., 85–111.Google Scholar
Bridson, MR and Haefliger, A (1999) Metric Spaces of Non-Positive Curvature, vol. 319. Springer.CrossRefGoogle Scholar
Bröcker, T and Dieck, TT (1985) Representations of Compact Lie Groups, vol. 98. Springer.CrossRefGoogle Scholar
Button, JO (2017) Properties of linear groups with restricted unipotent elements. arXiv preprint 1703.05553.Google Scholar
Button, JO (2019) Aspects of non positive curvature for linear groups with no infinite order unipotents. Groups, Geometry, and Dynamics 13, 277–293.CrossRefGoogle Scholar
Buyalo, SV and Kobel’skii, VL (1995) Geometrization of graph-manifolds. II. Isometric geometrization. Algebra i Analiz 7, 96–117.Google Scholar
Caprace, P-E and Monod, N (2009) Isometry groups of non-positively curved spaces: structure theory. Journal of Topology 2, 661–700.CrossRefGoogle Scholar
Douba, S (2022) Thin right-angled Coxeter groups in some uniform arithmetic lattices. Bull. Lond. Math. Soc. 54, 1653–1657.CrossRefGoogle Scholar
Douba, S (2023) Virtually unipotent curves in some non-NPC graph manifolds. Internat. J. Algebra Comput. 33, 1037–1054.CrossRefGoogle Scholar
Duchesne, B (2015) Superrigidity in infinite dimension and finite rank via harmonic maps. Groups, Geometry, and Dynamics 9, 133–148.CrossRefGoogle Scholar
Eberlein, P (1996) Geometry of Nonpositively Curved Manifolds. University of Chicago Press.Google Scholar
Haglund, F and Wise, DT (2008) Special cube complexes. Geom. Funct. Anal. 17, 1551–1620.CrossRefGoogle Scholar
Kahn, J and Markovic, V (2012) Immersing almost geodesic surfaces in a closed hyperbolic three manifold. Ann. of Math. (2) 175, 1127–1190.CrossRefGoogle Scholar
Kapovich, M (2001) Hyperbolic Manifolds and Discrete Groups. Boston: Birkhäuser.Google Scholar
Kapovich, M and Leeb, B (1996) Actions of discrete groups on nonpositively curved spaces. Mathematische Annalen 306, 341–352.CrossRefGoogle Scholar
Kargapolov, M and Merzljakov, JI (1979) Fundamentals of the Theory of Groups, vol. 62. Springer.CrossRefGoogle Scholar
Kleiner, B and Lott, J (2013) Geometrization of three-dimensional orbifolds via Ricci flow. Astérisque 101–177.Google Scholar
Liu, Y (2013) Virtual cubulation of nonpositively curved graph manifolds. Journal of Topology 6, 793–822.CrossRefGoogle Scholar
Margulis, GA (1984) Arithmeticity of the irreducible lattices in the semisimple groups of rank greater than $1$ . Invent. Math. 76, 93–120.CrossRefGoogle Scholar
Matsnev, D (2007) The Baum–Connes conjecture and proper group actions on affine buildings. arXiv preprint math/0703923.Google Scholar
Przytycki, P and Wise, DT (2014) Graph manifolds with boundary are virtually special. J. Topol. 7, 419–435.CrossRefGoogle Scholar
Przytycki, P and Wise, DT (2018) Mixed 3-manifolds are virtually special. Journal of the American Mathematical Society 31, 319–347.CrossRefGoogle Scholar
Radjavi, H and Rosenthal, P (2000) Simultaneous Triangularization. New York: Springer.CrossRefGoogle Scholar
Roman, S (2013) Advanced Linear Algebra, vol. 135. Springer.Google Scholar
Tits, J (1972) Free subgroups in linear groups. J. Algebra 20, 250–270.CrossRefGoogle Scholar
Wise, DT (2009) Research announcement: the structure of groups with a quasiconvex hierarchy. Electron. Res. Announc. Math. Sci. 16, 44–55.Google Scholar