Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-14T18:31:12.780Z Has data issue: false hasContentIssue false
  • English
  • Français

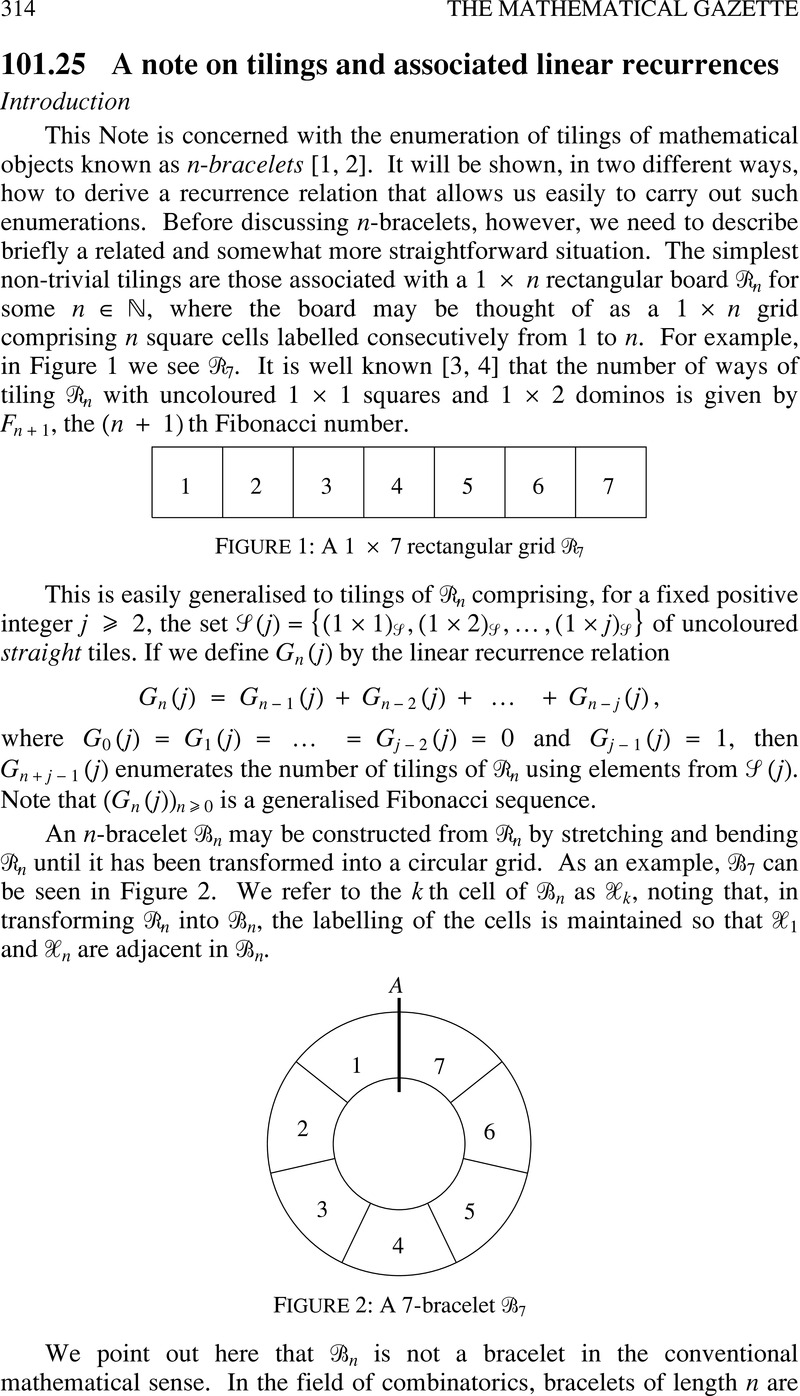
101.25 A note on tilings and associated linear recurrences

Published online by Cambridge University Press:  15 June 2017

Martin Griffiths
Martin Griffiths*
Affiliation:
Dept. of Mathematical Sciences, University of Essex, Colchester CO4 3SQ
Get access Rights & Permissions [Opens in a new window]

Abstract

An abstract is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
Image of the first page of this content. For PDF version, please use the ‘Save PDF’ preceeding this image.'
Type
Notes
Information
The Mathematical Gazette , Volume 101 , Issue 551 , July 2017 , pp. 314 - 318
Copyright
Copyright © Mathematical Association 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Benjamin, A. T. and Quinn, J. J., Fibonacci and Lucas identities through colored tilings, Utilitas Mathematica 56 (1999) pp. 137–142.Google Scholar
2. Bramham, A. and Griffiths, M., Combinatorial interpretations of some convolution identities, Fibonacci Quarterly, 54 (2016) pp. 335–339.Google Scholar
3. Benjamin, A. T. and Quinn, J. J., Proofs that really count: the art of combinatorial proof, The Mathematical Association of America (2013).Google Scholar
4. Benjamin, A. T., Eustis, A. and Plott, S.. The 99th Fibonacci identity. Electronic Journal of Combinatorics 15 (2008) pp. 1–13, R34.Google Scholar
5. Burton, D., Elementary number theory, McGraw-Hill (1998).Google Scholar
6. Hardy, G. H. and E. M. Wright, , An introduction to the theory of numbers (6th edn.), Oxford University Press (2008).Google Scholar