Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-14T07:20:05.059Z Has data issue: false hasContentIssue false
  • English
  • Français

The missing fractions in Brouncker's sequence of continued fractions for π

Published online by Cambridge University Press:  23 January 2015

Thomas J. Osler
Thomas J. Osler*
Affiliation:
Mathematics Department, Rowan University, Glassboro, NJ 08028 USA, e-mail: osler@rowan.edu
Get access Rights & Permissions [Opens in a new window]

Extract

The forgotten continued fractions

Lord Brouncker's continued fraction for π is

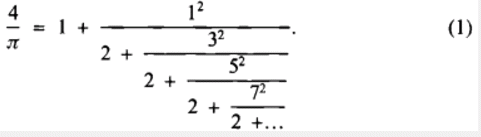
(In this paper we will use the more convenient notation This fraction first appeared in the Arithmetica Infinitorum [1] by John Wallis. In this book, along with topics that lead to Newton's calculus, Wallis derives his famous product

Wallis tells us that he showed this product to Lord Brouncker, and in turn Brouncker converted it not only into (1), but into an infinite sequence of continued fractions.

Type
Articles
Information
The Mathematical Gazette , Volume 96 , Issue 536 , July 2012 , pp. 221 - 225
Copyright
Copyright © The Mathematical Association 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Wallis, John, The Arithmetic of Infinitesimals (translated from Latin by Stedall, Jacqueline A.), Springer Verlag, New York (2004).Google Scholar
2. Osler, T. J., Morphing Lord Brouncker's continued fraction for π into the product of Wallis, Math. Gaz. 95 (March 2011) pp. 17–22.Google Scholar
3. Osler, T. J., Lord Brouncker's forgotten sequence of continued fractions for π, International Journal of Mathematical Education in Science and Technology, 41, Issue 1 (January 2010) pp. 105–110.CrossRefGoogle Scholar
4. Euler, L., De fractionibus continuis Wallisii (On the continued fractions of Wallis), originally published in Mémoires de l'académie des sciences de St-Petersbourg 5, 1815, pp. 24–44. Also see Opera Omnia: Series I, Volume 16, pp. 178-199. On the web at the Euler Archive http://www.math.dartrnouth.edu/~euler/.Google Scholar
5. Lange, L. J., An elegant continued fraction for n, The American Mathematical Monthly 106 (1999) pp. 456–458.Google Scholar
6. Perron, O., Die Lehre von den Kettenbruchen, Band II, Teubner, Stuttgart (1957). (There is a Chelsea edition of this book in which our equation (5) appears on page 255.)Google Scholar
7. Berndt, B. C., Ramanujan's Notebooks, Part II, Springer-Verlag, New York (1989).CrossRefGoogle Scholar