Here is a result which will surely make one sit up. We start with a sequence t1 = (1, 1, 2, 2, 4, 4, 8, 8, 16, 16, … ) in which each power of 2 occurs twice in succession, and produce a second sequence whose n th term is the sum of the n th and (n + 1) th terms of the original one; we get the sequence t2 = (2, 3, 4, 6, 8, 12, 16, 24, 32, … ). We repeat the same operation on the resulting sequence, and continue this, iteratively. Here are the sequences t3, t4, t5:
t3 = (5, 7, 10, 14, 20, 28, 40, 56, 80, 1l2, 160, 224, 320, 448,…),
t4 = (12, 17, 24, 34, 48, 68, 96, 136, 192, 272, 384, 544, 768,…),
t5 = (29,41, 58, 82, 116, 164,232, 328, 464, 656, 928, 1312,…).
If we compute the ratio of the second term to the first term for each ti, we get the following sequence of rational numbers,

which converges to the square root of 2. (These fractions turn out to be successive convergents to the simple continued fraction for √2; see Section IV for details.)
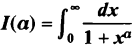 using complex numbers
using complex numbers