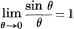Other
Thomas Archer Hirst
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 249
-
- Article
- Export citation
The Place of Mathematics in Secondary (Modern) Schools*
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 250-271
-
- Article
- Export citation
Gleanings Far and Near
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 271
-
- Article
- Export citation
Research Article
On Converse Theorems of Summability
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 272-276
-
- Article
- Export citation
A Treatment of the Exponential and Logarithmic Functions
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 277-281
-
- Article
- Export citation
A Lesson on π and on LIM (Sin θ/θ)
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 282-286
-
- Article
- Export citation
A Theorem in Uniform Convergence
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 287-290
-
- Article
- Export citation
Meeting Report
Annual General Meeting, 1947
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 290
-
- Article
- Export citation
Research Article
Solution of Cable Problem By Laplace Transform
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 291-292
-
- Article
- Export citation
Mathematical Notes
1936. The orthopole
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 293
-
- Article
- Export citation
1937. Extension of Simson's line. Sondat's theorem
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 293-295
-
- Article
- Export citation
1938. A classification of locus problems
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 295-297
-
- Article
- Export citation
1939. On the Simson lines
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 298
-
- Article
- Export citation
1940. On √a
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 298
-
- Article
- Export citation
1941. Why worry about method?
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 298
-
- Article
- Export citation
Other
Mathematical Association Teaching Committee
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 299-300
-
- Article
- Export citation
Correspondence
On Some Teaching Suggestions
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 300-302
-
- Article
-
- You have access
- Export citation
Need for a Symbol
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 302
-
- Article
-
- You have access
- Export citation
Projective Geometry
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 303
-
- Article
-
- You have access
- Export citation
Terminology in Dynamics
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 303-304
-
- Article
-
- You have access
- Export citation