No CrossRef data available.
Published online by Cambridge University Press: 09 July 2018
Let A ⊆ [1, N] be a set of integers with |A| ≫ 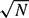 $\sqrt N$. We show that if A avoids about p/2 residue classes modulo p for each prime p, then A must correlate additively with the squares S = {n2 : 1 ≤ n ≤
$\sqrt N$. We show that if A avoids about p/2 residue classes modulo p for each prime p, then A must correlate additively with the squares S = {n2 : 1 ≤ n ≤ 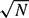 $\sqrt N$}, in the sense that we have the additive energy estimate
This is, in a sense, optimal.
$\sqrt N$}, in the sense that we have the additive energy estimate
This is, in a sense, optimal.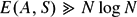 $$
E(A,S)\gg N\log N.
$$
$$
E(A,S)\gg N\log N.
$$