Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-27T09:57:12.045Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on the representation of functions by absolutely convergent fourier integrals

Published online by Cambridge University Press:  24 October 2008

H. R. Pitt
H. R. Pitt
Affiliation:
Queen's UniversityBelfast
Get access Rights & Permissions [Opens in a new window]

Extract

1. We write L for the class of integrable functions in (− ∞, ∞), V for the class of functions of bounded variation, and define A, A to be the classes of functions F(x) which may be expressed in the forms

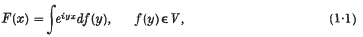
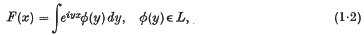
respectively.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 44 , Issue 1 , January 1948 , pp. 8 - 12
Copyright
Copyright © Cambridge Philosophical Society 1948

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

† Integrals without specified limits are over the range (− ∞, ∞).

‡ Wiener, N., The Fourier Integral (Cambridge, 1933), Lemma 67, p. 80.Google Scholar

§ Wiener, N. and Pitt, H. R., ‘On absolutely convergent Fourier-Stieltjes transforms’, Duke Math. J. 4 (1938), 420–35,CrossRefGoogle Scholar Lemma 1.

† Bochner, S., Fouriersche Integrale (Leipzig, 1932),Google Scholar Theorem 24 of § 21.

‡ Besicovitch, S., Almost Periodic Functions (Cambridge, 1932), § 3.Google Scholar