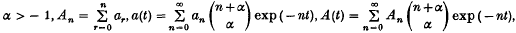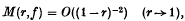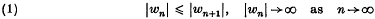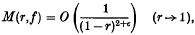Research Article
Large cycles in large labelled graphs. II
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 1-2
-
- Article
- Export citation
Jordan algebras spanned by Hermitian elements of a Banach algebra
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 3-13
-
- Article
- Export citation
Canonical extensions for embeddings of topological spheres of any codimension
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 15-24
-
- Article
- Export citation
Completeness and intertwined completeness of locally convex spaces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 25-30
-
- Article
- Export citation
Riesz spaces with the order-continuity property. I
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 31-42
-
- Article
- Export citation
The remainder in a gap Tauberian theorem for Abel summability
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 43-45
-
- Article
- Export citation
Means and coefficients of functions which omit a sequence of values
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 47-57
-
- Article
- Export citation
Spectral theory and Fuchsian groups
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 59-75
-
- Article
- Export citation
Innovation projections of a jump process and local martingales
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 77-90
-
- Article
- Export citation
On universal unfoldings of certain real functions on a Banach space
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 91-95
-
- Article
- Export citation
The wave front set of the solution of a simple initial-boundary value problem with glancing rays. II
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 97-120
-
- Article
- Export citation
Deductions from some artificial refractive index profiles based on the elementary functions
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 121-132
-
- Article
- Export citation
A generalized Poisson bracket and an associated natural one-form
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 133-142
-
- Article
- Export citation
Comersions and 1-connectedness
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 143-147
-
- Article
- Export citation
Black-hole magnetostatics
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 149-156
-
- Article
- Export citation
Sluice-gate problems with gravity
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 157-175
-
- Article
- Export citation
Front matter
PSP volume 81 issue 1 Cover and Front matter
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back matter
PSP volume 81 issue 1 Cover and Back matter
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. b1-b2
-
- Article
-
- You have access
- Export citation