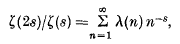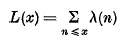Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Turán, P.
1959.
Nachtrag zu meiner Abhandlung „On some approximative Dirichlet polynomials in the theory of zeta-function of Riemann”.
Acta Mathematica Academiae Scientiarum Hungaricae,
Vol. 10,
Issue. 3-4,
p.
277.
Lehman, R. Sherman
1960.
On Liouville’s function.
Mathematics of Computation,
Vol. 14,
Issue. 72,
p.
311.
Bateman, Paul T
1966.
Lower bounds ∑ h(m)m for arithmetical functions h similar to real residue-characters.
Journal of Mathematical Analysis and Applications,
Vol. 15,
Issue. 1,
p.
2.
DAVENPORT, H.
1967.
A. E. Ingham.
Nature,
Vol. 216,
Issue. 5112,
p.
311.
Grosswald, Emil
1967.
Oscillation theorems of arithmetical functions.
Transactions of the American Mathematical Society,
Vol. 126,
Issue. 1,
p.
1.
Spira, Robert
1968.
Zeros of sections of the zeta function. II.
Mathematics of Computation,
Vol. 22,
Issue. 101,
p.
163.
Burkill, John Charles
1968.
Albert Edward Ingham, 1900-1967.
Biographical Memoirs of Fellows of the Royal Society,
Vol. 14,
Issue. ,
p.
271.
Good, I. J.
and
Churchhouse, R. F.
1968.
The Riemann hypothesis and pseudorandom features of the Möbius sequence.
Mathematics of Computation,
Vol. 22,
Issue. 104,
p.
857.
Diamond, Harold G.
1972.
The Theory of Arithmetic Functions.
Vol. 251,
Issue. ,
p.
113.
Grosswald, Emil
1972.
The Theory of Arithmetic Functions.
Vol. 251,
Issue. ,
p.
141.
Anderson, Robert J.
and
Stark, H. M.
1981.
Analytic Number Theory.
Vol. 899,
Issue. ,
p.
79.
Murty, M. Ram
and
Sankaranarayanan, A.
2002.
Averages of exponential twists of the Liouville function.
Forum Mathematicum,
Vol. 14,
Issue. 2,
Narkiewicz, Władysław
2012.
Rational Number Theory in the 20th Century.
p.
13.
Milinovich, Micah B.
and
Ng, Nathan
2012.
A note on a conjecture of Gonek.
Functiones et Approximatio Commentarii Mathematici,
Vol. 46,
Issue. 2,
MOSSINGHOFF, MICHAEL J.
and
TRUDGIAN, TIMOTHY S.
2012.
BETWEEN THE PROBLEMS OF PÓLYA AND TURÁN.
Journal of the Australian Mathematical Society,
Vol. 93,
Issue. 1-2,
p.
157.
Borwein, Peter
Choi, Stephen K. K.
and
Ganguli, Himadri
2013.
Sign Changes of the Liouville Function on Quadratics.
Canadian Mathematical Bulletin,
Vol. 56,
Issue. 2,
p.
251.
Humphries, Peter
2013.
The distribution of weighted sums of the Liouville function and Pólyaʼs conjecture.
Journal of Number Theory,
Vol. 133,
Issue. 2,
p.
545.
Mora, G.
2013.
On the asymptotically uniform distribution of the zeros of the partial sums of the Riemann zeta function.
Journal of Mathematical Analysis and Applications,
Vol. 403,
Issue. 1,
p.
120.
Berry, Don
2016.
Proof and the Virtues of Shared Enquiry.
Philosophia Mathematica,
p.
nkw022.
Sun, Zhi-Wei
2016.
On a pair of zeta functions.
International Journal of Number Theory,
Vol. 12,
Issue. 08,
p.
2323.