Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-27T21:17:03.408Z Has data issue: false hasContentIssue false
  • English
  • Français

One-class genera of positive ternary quadratic forms—II

Published online by Cambridge University Press:  26 February 2010

G. L. Watson
G. L. Watson
Affiliation:
University College, London.
Get access

Extract

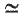Let f be a positive-definite ternary quadratic form with integer coefficients; by c(f), the class-number of f, is meant the number of classes in the genus of f. The object of this paper is to find all the f with c(f) = 1; these f are the ones for which , where f′ is an arbitrary ternary form and ∼, denote equivalence and semi-equivalence respectively. Trivially, it suffices to find the primitive f with c(f) = 1.

Type
Research Article
Information
Mathematika , Volume 22 , Issue 1 , June 1975 , pp. 1 - 11
Copyright
Copyright © University College London 1975

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Watson, G. L.. “One-class genera of positive ternary quadratic forms”, Mathematika, 19 (1972), 96–104.CrossRefGoogle Scholar
2. Watson, G. L.. “Transformations of a quadratic form which do not increase the class-number”, Proc. London Math. Soc, (3), 12 (1962), 577–587.CrossRefGoogle Scholar
3. Watson, G. L.. “Transformations of a quadratic form which do not increase the class-number (II), Acta Arithmetica, 27 (1974), 171–189.CrossRefGoogle Scholar
4. Watson, G. L.. “One-class genera of positive quaternary quadratic forms”, Acta Arithmetica, 24 (1974), 461–475.CrossRefGoogle Scholar
5. Watson, G. L.. “One-class genera of positive quadratic forms in n ≤ 5 variables”, Acta Arithmetica, 26 (1974), 309–327.CrossRefGoogle Scholar
6. Watson, G. L.. “Regular, positive, ternary quadratic forms”, J. London Math. Soc. (2) [to appear].CrossRefGoogle Scholar
7. Watson, G. L.. Integral quadratic forms, Cambridge Tracts in Mathematics and Mathematical Physics, No. 51 (Cambridge, 1960).Google Scholar