No CrossRef data available.
Article contents
Approximation numbers of composition operators on weighted besov spaces of analytic functions
Part of:
Spaces and algebras of analytic functions
Special classes of linear operators
Two-dimensional theory
Published online by Cambridge University Press: 28 February 2022
Abstract
Li et al. [A spectral radius type formula for approximation numbers of composition operators, J. Funct. Anal. 267(12) (2014), 4753-4774] proved a spectral radius type formula for the approximation numbers of composition operators on analytic Hilbert spaces with radial weights and on $H^{p}$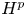 spaces, $p\geq 1$
spaces, $p\geq 1$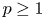 , involving Green capacity. We prove that their formula holds for a wide class of Banach spaces of analytic functions and weights.
, involving Green capacity. We prove that their formula holds for a wide class of Banach spaces of analytic functions and weights.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © The Author(s), 2022. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society
References
Aleman, A., The multiplication operator on Hilbert spaces of analytic functions, (Habilitation, FernUniversität Hagen, 1993).Google Scholar
Bao, G., Göğüş, N. G. and Pouliasis, S., On Dirichlet spaces with a class of superharmonic weights, Canad. J. Math. 70 (2018), 721–741.CrossRefGoogle Scholar
Costara, C. and Ransford, T., Which de Branges-Rovnyak spaces are Dirichlet spaces (and vice versa)?, J. Funct. Anal. 265 (2013), 3204–3218.CrossRefGoogle Scholar
Cowen, C. C. and MacCluer, B. D., Composition operators on spaces of analytic functions, Studies in Advanced Mathematics (CRC Press, 1995).Google Scholar
Dubinin, V. N., Condenser capacities and symmetrization in geometric function theory, Translated from the Russian by Nikolai G. Kruzhilin (Springer, Basel, 2014).CrossRefGoogle Scholar
El-Fallah, O., Kellay, K., Mashreghi, J. and Ransford, T., A primer on the Dirichlet space, Cambridge Tracts in Mathematics, Volume 203 (Cambridge University Press, 2014).CrossRefGoogle Scholar
El-Fallah, O., Kellay, K., Klaja, H., Mashreghi, J. and Ransford, T., Dirichlet spaces with superharmonic weights and de Branges-Rovnyak spaces, Complex Anal. Operator Theory 10 (2016), 97–107.CrossRefGoogle Scholar
Fisher, S. D., Function theory on planar domains. A second course in complex analysis, Pure and Applied Mathematics (John Wiley and Sons, 1983).Google Scholar
Götz, M., Approximating the condenser equilibrium distribution, Math. Z. 236(4) (2001), 699–715.CrossRefGoogle Scholar
Kloke, H., Punktsysteme mit extremalen Eigenschaften für ebene Kondensatoren, Dissertation Universität Dortmund, 1984.Google Scholar
Kloke, H., On the capacity of a plane condenser and conformal mapping, J. Reine Angew. Math. 358 (1985), 179–201.Google Scholar
Landkof, N. S., Foundations of Modern Potential Theory (Springer-Verlag, 1972).CrossRefGoogle Scholar
Li, D., Queffélec, H. and Rodríguez-Piazza, L., A spectral radius type formula for approximation numbers of composition operators, J. Funct. Anal. 267(12) (2014), 4753–4774.CrossRefGoogle Scholar
Richter, S., A representation theorem for cyclic analytic two-isometries, Trans. Amer. Math. Soc. 328 (1991), 325–349.CrossRefGoogle Scholar
Richter, S. and Sundberg, C., A formula for the local Dirichlet integral, Michigan Math. J. 38 (1991), 355–379.CrossRefGoogle Scholar
Sarason, D., Local Dirichlet spaces as de Branges-Rovnyak spaces, Proc. Amer. Math. Soc. 125 (1997), 2133–2139.CrossRefGoogle Scholar
Shapiro, J. H., Composition operators and classical function theory, Universitext, Tracts in Mathematics (Springer-Verlag, 1993).CrossRefGoogle Scholar
Shimorin, S. M., Complete Nevanlinna-Pick property of Dirichlet-type spaces, J. Funct. Anal. 191 (2002), 276–296.CrossRefGoogle Scholar
Siciak, J., Wiener's type regularity criteria on the complex plane, Ann. Polon. Math. 66 (1997), 203–221.CrossRefGoogle Scholar
Vuorinen, M., Conformal geometry and quasiregular mappings, Lecture Notes in Math. Volume 1319 (Springler-Verlag, 1988).CrossRefGoogle Scholar
Widom, H., Rational approximation and $n$ -dimensional diameter, J. Approx. Theory 5 (1972), 343–361.CrossRefGoogle Scholar
-dimensional diameter, J. Approx. Theory 5 (1972), 343–361.CrossRefGoogle Scholar
Wojtaszczyk, P., Banach spaces for analysts, Cambridge Studies in Advanced Mathematics, Volume 25 (Cambridge University Press, 1991).CrossRefGoogle Scholar
Zhu, K., Operator theory in function spaces, 2nd edn, Mathematical Surveys and Monographs, Volume 138 (American Mathematical Society, 2007).CrossRefGoogle Scholar


