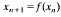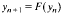Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Chow, S.-N.
and
Mallet-Paret, J.
1995.
Pattern formation and spatial chaos in lattice dynamical systems. I.
IEEE Transactions on Circuits and Systems I: Fundamental Theory and Applications,
Vol. 42,
Issue. 10,
p.
746.
Hale, Jack K.
1995.
Topological Methods in Differential Equations and Inclusions.
p.
191.
Van Vleck, Erik S.
Mallet-Paret, John
and
Cahn, John W.
1998.
Traveling Wave Solutions for Systems of ODEs on a Two-Dimensional Spatial Lattice.
SIAM Journal on Applied Mathematics,
Vol. 59,
Issue. 2,
p.
455.
Nussbaum, Roger D.
2002.
Vol. 2,
Issue. ,
p.
461.
Chow, Shui-Nee
Nussbaum, Roger D.
and
Walther, Hans-Otto
2016.
John Mallet-Paret 60.
Journal of Dynamics and Differential Equations,
Vol. 28,
Issue. 3-4,
p.
595.