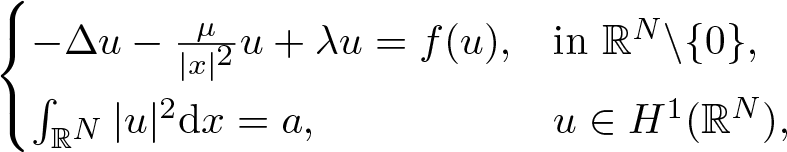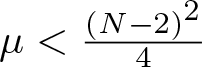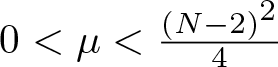1. Introduction
In recent decades, significant attention has been directed towards the exploration of standing wave solutions in the context of the time-dependent Schrödinger equation, which is formulated as follows
 \begin{align}
\begin{cases}
i \varPhi _t+\Delta \varPhi +g\left(|\varPhi|^2\right)
\varPhi=0,~t\ge0,~x\in\mathbb{R}^N, \\
\int_{\mathbb{R}^{N}}|\varPhi|^{2}\mathrm{d} x=a.
\end{cases}
\end{align}
\begin{align}
\begin{cases}
i \varPhi _t+\Delta \varPhi +g\left(|\varPhi|^2\right)
\varPhi=0,~t\ge0,~x\in\mathbb{R}^N, \\
\int_{\mathbb{R}^{N}}|\varPhi|^{2}\mathrm{d} x=a.
\end{cases}
\end{align} In this context, i represents the imaginary unit, ![]() $N\geq 3$, a > 0, and
$N\geq 3$, a > 0, and ![]() $g:\left[0, \infty\right) \rightarrow \mathbb{R}$ is a nonlinear term. The function
$g:\left[0, \infty\right) \rightarrow \mathbb{R}$ is a nonlinear term. The function ![]() $\varPhi(t,x):\mathbb{R} \times \mathbb{R}^N \rightarrow \mathbb{C}$ is the wave function. Equation (1.1) arises naturally in the time-dependent Cauchy problem given by
$\varPhi(t,x):\mathbb{R} \times \mathbb{R}^N \rightarrow \mathbb{C}$ is the wave function. Equation (1.1) arises naturally in the time-dependent Cauchy problem given by
 \begin{align}
\begin{cases}
i \varPhi _t+\Delta \varPhi+g\left(|\varPhi|^2\right) \varPhi=0, \\
\varPhi(\cdot,0)=\varphi_0 \in L^2\left(\mathbb{R}^N\right) \backslash\{0\}.
\end{cases}
\end{align}
\begin{align}
\begin{cases}
i \varPhi _t+\Delta \varPhi+g\left(|\varPhi|^2\right) \varPhi=0, \\
\varPhi(\cdot,0)=\varphi_0 \in L^2\left(\mathbb{R}^N\right) \backslash\{0\}.
\end{cases}
\end{align} The L 2-normalization condition in Eq. (1.1) stems from the conservation of the L 2-norm in Eq. (1.2). Indeed multiplying Eq. (1.2) by ![]() $\overline{\varPhi}$, integrating, and taking the imaginary part leads to
$\overline{\varPhi}$, integrating, and taking the imaginary part leads to  $\frac{d}{dt} \int_{\mathbb{R}^N}|\varPhi|^2 \mathrm{d}x = 0$ and therefore we can define
$\frac{d}{dt} \int_{\mathbb{R}^N}|\varPhi|^2 \mathrm{d}x = 0$ and therefore we can define  $\int_{\mathbb{R}^N}|\varPhi|^2 \mathrm{d}x = \int_{\mathbb{R}^N}|\varphi_0|^2 \mathrm{d}x=a$, see [Reference Bieganowski, Mederski and Schino12]. The pursuit of solutions with prescribed L 2-norms holds profound significance from both physical and mathematical vantage points. From a physical perspective, the search for solutions characterized by a predetermined L 2-norm is intricately linked with the principle of mass conservation, carrying fundamental physical interpretations across diverse domains. For instance, in the field of nonlinear optics, the L 2-norm corresponds to the power magnitude, while in Bose–Einstein condensates, it encapsulates the particle count and assumes a pivotal role in delineating the system’s behaviour (refer to [Reference Akhmediev and Ankiewicz1, Reference Frantzeskakis20, Reference Timmermans48]). From a mathematical stance, the examination of solutions with prescribed L 2-norms contributes invaluable insights into the characteristics and dynamics of these solutions, thereby fostering a deeper comprehension of stability and instability phenomena (refer to [Reference Berestycki and Cazenave8, Reference Cazenave and Lions15]).
$\int_{\mathbb{R}^N}|\varPhi|^2 \mathrm{d}x = \int_{\mathbb{R}^N}|\varphi_0|^2 \mathrm{d}x=a$, see [Reference Bieganowski, Mederski and Schino12]. The pursuit of solutions with prescribed L 2-norms holds profound significance from both physical and mathematical vantage points. From a physical perspective, the search for solutions characterized by a predetermined L 2-norm is intricately linked with the principle of mass conservation, carrying fundamental physical interpretations across diverse domains. For instance, in the field of nonlinear optics, the L 2-norm corresponds to the power magnitude, while in Bose–Einstein condensates, it encapsulates the particle count and assumes a pivotal role in delineating the system’s behaviour (refer to [Reference Akhmediev and Ankiewicz1, Reference Frantzeskakis20, Reference Timmermans48]). From a mathematical stance, the examination of solutions with prescribed L 2-norms contributes invaluable insights into the characteristics and dynamics of these solutions, thereby fostering a deeper comprehension of stability and instability phenomena (refer to [Reference Berestycki and Cazenave8, Reference Cazenave and Lions15]).
Consider the standing wave solution denoted as ![]() $\varPhi (t, x)=e^{i \lambda t} u(x)$ in Eq. (1.1), where
$\varPhi (t, x)=e^{i \lambda t} u(x)$ in Eq. (1.1), where ![]() $u: \mathbb{R}^N \rightarrow \mathbb{R}$. Subsequently, we transform Eq. (1.1) into a new form
$u: \mathbb{R}^N \rightarrow \mathbb{R}$. Subsequently, we transform Eq. (1.1) into a new form
 \begin{align}
\begin{cases}
-\Delta u +\lambda u=f(u), \quad \text{in}\quad \mathbb{R}^N , \\
\int_{\mathbb{R}^{N}}|u|^{2}\mathrm{d} x=a,
\end{cases}
\end{align}
\begin{align}
\begin{cases}
-\Delta u +\lambda u=f(u), \quad \text{in}\quad \mathbb{R}^N , \\
\int_{\mathbb{R}^{N}}|u|^{2}\mathrm{d} x=a,
\end{cases}
\end{align} where ![]() $f(u)=g\left(|u|^2\right) u$. Equation (1.3) characterizes the steady-state behaviour of the wave function. In order to analyse Eq. (1.3), we introduce the energy functional
$f(u)=g\left(|u|^2\right) u$. Equation (1.3) characterizes the steady-state behaviour of the wave function. In order to analyse Eq. (1.3), we introduce the energy functional
 \begin{equation*}
\mathcal{E}(u)=\frac{1}{2}\int_{\mathbb{R}^{N}}|\nabla u|^2\mathrm{d} x-\int_{\mathbb{R}^{N}}F(u)\mathrm{d} x,
\end{equation*}
\begin{equation*}
\mathcal{E}(u)=\frac{1}{2}\int_{\mathbb{R}^{N}}|\nabla u|^2\mathrm{d} x-\int_{\mathbb{R}^{N}}F(u)\mathrm{d} x,
\end{equation*} where  $F(u)=\int_0^{u} f(\tau) d \tau$, and
$F(u)=\int_0^{u} f(\tau) d \tau$, and ![]() $\mathcal{E}$ belongs to the class C 1 on
$\mathcal{E}$ belongs to the class C 1 on ![]() $H^1\left(\mathbb{R}^N\right)$. A critical point of
$H^1\left(\mathbb{R}^N\right)$. A critical point of ![]() $\mathcal{E}$ under the mass constraint Sa,
$\mathcal{E}$ under the mass constraint Sa,
 \begin{equation*}
S_a=\left\{u\in H^1(\mathbb{R}^N):\int_{\mathbb{R}^{N}}|u|^2 \mathrm{d} x=a\right\},
\end{equation*}
\begin{equation*}
S_a=\left\{u\in H^1(\mathbb{R}^N):\int_{\mathbb{R}^{N}}|u|^2 \mathrm{d} x=a\right\},
\end{equation*}known as the normalized solution, is a solution of Eq. (1.3).
In the case of Eq. (1.3) with ![]() $f(u)=\mu|u|^{q-2}u+|u|^{p-2}u$,
$f(u)=\mu|u|^{q-2}u+|u|^{p-2}u$, ![]() $2 \lt q\leq p\leq 2^*$, the exploration of normalized ground state solutions for Eq. (1.3) was undertaken by Soave in [Reference Soave43, Reference Soave44]. Building upon the foundational contributions of Soave, subsequent scholarly endeavours have further engaged with Eq. (1.3), as exemplified by works such as [Reference Alves, Ji and Miyagaki2, Reference Jeanjean and Le29, Reference Li36, Reference Wei and Wu49]. For the general nonlinear terms f, it is noteworthy to mention the investigation carried out by Jeanjean in [Reference Jeanjean27], who assumed that
$2 \lt q\leq p\leq 2^*$, the exploration of normalized ground state solutions for Eq. (1.3) was undertaken by Soave in [Reference Soave43, Reference Soave44]. Building upon the foundational contributions of Soave, subsequent scholarly endeavours have further engaged with Eq. (1.3), as exemplified by works such as [Reference Alves, Ji and Miyagaki2, Reference Jeanjean and Le29, Reference Li36, Reference Wei and Wu49]. For the general nonlinear terms f, it is noteworthy to mention the investigation carried out by Jeanjean in [Reference Jeanjean27], who assumed that ![]() $f:\mathbb{R}\to\mathbb{R}$ satisfies
$f:\mathbb{R}\to\mathbb{R}$ satisfies
(H1) ![]() $f\in C(\mathbb{R},\mathbb{R})$ and odd.
$f\in C(\mathbb{R},\mathbb{R})$ and odd.
(H2) There exist ![]() $\alpha,\beta\in \mathbb{R}$ satisfying
$\alpha,\beta\in \mathbb{R}$ satisfying  $2+\frac{4}{N} \lt \alpha\le\beta \lt 2^*=\frac{2N}{N-2}$ such that
$2+\frac{4}{N} \lt \alpha\le\beta \lt 2^*=\frac{2N}{N-2}$ such that
(H3) The function  $\widetilde{F}(t):=f(t)t-2F(t)$ is of class C 1 and satisfies
$\widetilde{F}(t):=f(t)t-2F(t)$ is of class C 1 and satisfies
 \begin{equation*}
\widetilde{F}^{\prime}(t)t \gt \frac{2N+4}{N}\widetilde{F}(t)~~\mathrm{for~ any}~t\neq 0.
\end{equation*}
\begin{equation*}
\widetilde{F}^{\prime}(t)t \gt \frac{2N+4}{N}\widetilde{F}(t)~~\mathrm{for~ any}~t\neq 0.
\end{equation*} and established the existence of normalized ground state solutions to Eq. (1.3) for any ![]() $N\geq 1$. Subsequently, for
$N\geq 1$. Subsequently, for ![]() $N\geq 2$, Bartsch and de Valeriola in [Reference Bartsch and de Valeriola4] obtained an infinite number of radial normalized solutions for Eq. (1.3), provided
$N\geq 2$, Bartsch and de Valeriola in [Reference Bartsch and de Valeriola4] obtained an infinite number of radial normalized solutions for Eq. (1.3), provided ![]() $(H1)$ and
$(H1)$ and ![]() $(H2)$. Furthermore, Jeanjean and Lu [Reference Jeanjean and Lu31] revisited Eq. (1.3) under the following assumptions:
$(H2)$. Furthermore, Jeanjean and Lu [Reference Jeanjean and Lu31] revisited Eq. (1.3) under the following assumptions:
(H4) ![]() $ f:\mathbb{R}\to\mathbb{R} $ is continuous.
$ f:\mathbb{R}\to\mathbb{R} $ is continuous.
(H5)  $ \lim_{s\to0}\frac{f(s)}{|s|^{1+4/N}}=0 $ and
$ \lim_{s\to0}\frac{f(s)}{|s|^{1+4/N}}=0 $ and  $ \lim_{s\to\infty}\frac{f(s)}{|s|^{(N+2)/(N-2)}}=0 $.
$ \lim_{s\to\infty}\frac{f(s)}{|s|^{(N+2)/(N-2)}}=0 $.
(H6)  $ \lim_{s\to\infty}\frac{F(s)}{|s|^{2+4/N}}=+\infty $.
$ \lim_{s\to\infty}\frac{F(s)}{|s|^{2+4/N}}=+\infty $.
(H7)  $ f(s)s \lt \frac{2N}{N-2}F(s) $ for all
$ f(s)s \lt \frac{2N}{N-2}F(s) $ for all ![]() $ s \in \mathbb{R} \setminus \{0\} $.
$ s \in \mathbb{R} \setminus \{0\} $.
(H8) The function  $ s \mapsto \frac{\widetilde{F}(s)}{|s|^{2+4/N}} $ is strictly decreasing on
$ s \mapsto \frac{\widetilde{F}(s)}{|s|^{2+4/N}} $ is strictly decreasing on ![]() $ (-\infty,0) $ and strictly increasing on
$ (-\infty,0) $ and strictly increasing on ![]() $ (0,+\infty) $.
$ (0,+\infty) $.
Due to ![]() $(H4)$–
$(H4)$–![]() $(H8)$, which do not require
$(H8)$, which do not require ![]() $ \widetilde{F} \in C^1 $, the authors established the existence of normalized ground state solutions by adapting the argument and employing techniques from Szulkin and Weth [Reference Szulkin and Weth45, Reference Szulkin and Weth46]. Subsequently, the authors extend the results of Jeanjean [Reference Jeanjean27] regarding the existence of normalized ground state solutions. For readers interested in exploring normalized solutions of Eq. (1.3), we recommend further investigations into works such as [Reference Bartsch and Soave6, Reference Bieganowski and Mederski11, Reference Hirata and Tanaka25, Reference Jeanjean, Jendrej, Le and Visciglia28, Reference Jeanjean and Lu30, Reference Jeanjean, Zhang and Zhong32, Reference Jia and Luo33, Reference Liu and Chang38, Reference Shibata42, Reference Yang51], along with the references they provide. These works offer deeper insights and additional research pertaining to this subject.
$ \widetilde{F} \in C^1 $, the authors established the existence of normalized ground state solutions by adapting the argument and employing techniques from Szulkin and Weth [Reference Szulkin and Weth45, Reference Szulkin and Weth46]. Subsequently, the authors extend the results of Jeanjean [Reference Jeanjean27] regarding the existence of normalized ground state solutions. For readers interested in exploring normalized solutions of Eq. (1.3), we recommend further investigations into works such as [Reference Bartsch and Soave6, Reference Bieganowski and Mederski11, Reference Hirata and Tanaka25, Reference Jeanjean, Jendrej, Le and Visciglia28, Reference Jeanjean and Lu30, Reference Jeanjean, Zhang and Zhong32, Reference Jia and Luo33, Reference Liu and Chang38, Reference Shibata42, Reference Yang51], along with the references they provide. These works offer deeper insights and additional research pertaining to this subject.
In a parallel vein of research, certain scholars have introduced an external potential V in Eq. (1.3), i.e.
 \begin{align}
\left\{\begin{array}{l}
-\Delta u+V(x) u+\lambda u=f(u) \ \ \ \ \text {in } \mathbb{R}^N ,\\
\int_{\mathbb{R}^N}|u|^2 \mathrm{d} x=a.
\end{array}\right.
\end{align}
\begin{align}
\left\{\begin{array}{l}
-\Delta u+V(x) u+\lambda u=f(u) \ \ \ \ \text {in } \mathbb{R}^N ,\\
\int_{\mathbb{R}^N}|u|^2 \mathrm{d} x=a.
\end{array}\right.
\end{align} For the case where ![]() $f(u)=|u|^{p-2}u$ with
$f(u)=|u|^{p-2}u$ with ![]() $2 \lt p \lt 2^*$, Pellacci et al. [Reference Pellacci, Pistoia, Vaira and Verzini40] considered the existence of normalized solutions for Eq. (1.4) if V possesses a non-degenerate critical point, who employed the Lyapunov–Schmidt reduction approach to establish the existence of normalized solutions for Eq. (1.4), contingent on the condition that a is sufficiently large and
$2 \lt p \lt 2^*$, Pellacci et al. [Reference Pellacci, Pistoia, Vaira and Verzini40] considered the existence of normalized solutions for Eq. (1.4) if V possesses a non-degenerate critical point, who employed the Lyapunov–Schmidt reduction approach to establish the existence of normalized solutions for Eq. (1.4), contingent on the condition that a is sufficiently large and  $p \lt 2+\frac{4}{N}$, or a is suitably small and
$p \lt 2+\frac{4}{N}$, or a is suitably small and  $p \gt 2+\frac{4}{N}$. Simultaneously, Bartsch et al. [Reference Bartsch, Molle, Rizzi and Verzini5] employed min-max arguments to establish the existence of normalized solutions for Eq. (1.4) with
$p \gt 2+\frac{4}{N}$. Simultaneously, Bartsch et al. [Reference Bartsch, Molle, Rizzi and Verzini5] employed min-max arguments to establish the existence of normalized solutions for Eq. (1.4) with  $2+\frac{4}{N} \lt p \lt 2^*$ and
$2+\frac{4}{N} \lt p \lt 2^*$ and ![]() $V(x) \geq 0$ tends to zero at infinity.
$V(x) \geq 0$ tends to zero at infinity.
Subsequently, the authors in [Reference Molle, Riey and Verzini39] obtained the existence of normalized solutions for Eq. (1.4), when  $2+\frac{4}{N} \lt p \lt 2^*$,
$2+\frac{4}{N} \lt p \lt 2^*$, ![]() $V(x)\leq 0$ satisfies
$V(x)\leq 0$ satisfies  $V(x) \leq \limsup \limits_{|x| \rightarrow+\infty}V(x) \lt +\infty$, and
$V(x) \leq \limsup \limits_{|x| \rightarrow+\infty}V(x) \lt +\infty$, and
 \begin{equation*}
\max \left\{\left|W\right|_{N}, |V|_{\frac{N}{2}}\right\} \lt M, \ \ \ \ \text{for~some~} M\in\mathbb{R}^+, ~\text{where}~W(x)=V(x)|x|.
\end{equation*}
\begin{equation*}
\max \left\{\left|W\right|_{N}, |V|_{\frac{N}{2}}\right\} \lt M, \ \ \ \ \text{for~some~} M\in\mathbb{R}^+, ~\text{where}~W(x)=V(x)|x|.
\end{equation*} For the general nonlinearity terms f in Eq. (1.4), Ding and Zhong [Reference Ding and Zhong18] assumed that f satisfies ![]() $(H1)$,
$(H1)$, ![]() $(H2)$, and
$(H2)$, and ![]() $(H3^\prime)$:
$(H3^\prime)$:
(H3ʹ) The functional  $\widetilde{F}(s)= f(s) s-2F(s)$ is of class C 1 and
$\widetilde{F}(s)= f(s) s-2F(s)$ is of class C 1 and
 \begin{equation*}
\widetilde{F}^{\prime}(s) s \geq \alpha \widetilde{F}(s), \text{~for any } s \in \mathbb{R},
\end{equation*}
\begin{equation*}
\widetilde{F}^{\prime}(s) s \geq \alpha \widetilde{F}(s), \text{~for any } s \in \mathbb{R},
\end{equation*}and V satisfies
(V3)  $\lim \limits_{|x| \rightarrow+\infty} V(x)=\sup _{x \in \mathbb{R}^N} V(x)=0$ and there exists some
$\lim \limits_{|x| \rightarrow+\infty} V(x)=\sup _{x \in \mathbb{R}^N} V(x)=0$ and there exists some  $\sigma_1 \in\left[0, \frac{N(\alpha-2)-4}{N(\alpha-2)}\right)$ such that
$\sigma_1 \in\left[0, \frac{N(\alpha-2)-4}{N(\alpha-2)}\right)$ such that
 \begin{equation*}
\left|\int_{\mathbb{R}^N} V(x) u^2 \mathrm{d} x\right| \leq \sigma_1|\nabla u|_2^2,\text{~for any } u \in H^1\left(\mathbb{R}^N\right).
\end{equation*}
\begin{equation*}
\left|\int_{\mathbb{R}^N} V(x) u^2 \mathrm{d} x\right| \leq \sigma_1|\nabla u|_2^2,\text{~for any } u \in H^1\left(\mathbb{R}^N\right).
\end{equation*} (V4) ![]() $\nabla V(x)$ exists a.e. in
$\nabla V(x)$ exists a.e. in ![]() $\mathbb{R}^N$ and coincides to the weak gradient of V, put
$\mathbb{R}^N$ and coincides to the weak gradient of V, put ![]() $W(x):=$
$W(x):=$  $\frac{1}{2}\langle\nabla V(x), x\rangle$. There exists some
$\frac{1}{2}\langle\nabla V(x), x\rangle$. There exists some  $0 \leq \sigma_2 \lt \min \left\{\frac{N(\alpha-2)\left(1-\sigma_1\right)}{4}-1, \frac{N}{\beta}-\frac{N-2}{2}\right\}$ such that
$0 \leq \sigma_2 \lt \min \left\{\frac{N(\alpha-2)\left(1-\sigma_1\right)}{4}-1, \frac{N}{\beta}-\frac{N-2}{2}\right\}$ such that
 \begin{equation*}
\left|\int_{\mathbb{R}^N} W(x) u^2 \mathrm{d} x\right| \leq \sigma_2|\nabla u|_2^2, \text{~for any } u \in H^1\left(\mathbb{R}^N\right).
\end{equation*}
\begin{equation*}
\left|\int_{\mathbb{R}^N} W(x) u^2 \mathrm{d} x\right| \leq \sigma_2|\nabla u|_2^2, \text{~for any } u \in H^1\left(\mathbb{R}^N\right).
\end{equation*} (V5) ![]() $\nabla W(x)$ exists a.e. in
$\nabla W(x)$ exists a.e. in ![]() $\mathbb{R}^N$ and coincides to the weak gradient of W, put
$\mathbb{R}^N$ and coincides to the weak gradient of W, put
 \begin{equation*}
Y(x):=\left(\frac{N}{2} \alpha-N\right) W(x)+\langle\nabla W(x), x\rangle,
\end{equation*}
\begin{equation*}
Y(x):=\left(\frac{N}{2} \alpha-N\right) W(x)+\langle\nabla W(x), x\rangle,
\end{equation*}  $\int_{\mathbb{R}^N} Y(x) u^2 \mathrm{d} x$ is well-defined for all
$\int_{\mathbb{R}^N} Y(x) u^2 \mathrm{d} x$ is well-defined for all ![]() $u \in H^1\left(\mathbb{R}^N\right)$ and there exists some
$u \in H^1\left(\mathbb{R}^N\right)$ and there exists some  $\sigma_3 \in\left[0, \frac{N}{2} \alpha-N-2 \right)$ such that
$\sigma_3 \in\left[0, \frac{N}{2} \alpha-N-2 \right)$ such that
 \begin{equation*}
\int_{\mathbb{R}^N} Y_{+}(x) u^2 \mathrm{d} x\leq \sigma_3|\nabla u|_2^2, \text{~for any }
u \in H^1\left(\mathbb{R}^N\right).
\end{equation*}
\begin{equation*}
\int_{\mathbb{R}^N} Y_{+}(x) u^2 \mathrm{d} x\leq \sigma_3|\nabla u|_2^2, \text{~for any }
u \in H^1\left(\mathbb{R}^N\right).
\end{equation*} Under ![]() $(H1)$,
$(H1)$, ![]() $(H2)$,
$(H2)$, ![]() $(H3^\prime)$ and
$(H3^\prime)$ and ![]() $(V3)$–
$(V3)$–![]() $(V5)$, the authors proved the existence of normalized solutions for Eq. (1.4) for any given a > 0. Li and Zou [Reference Li and Zou35] recently studied the case where
$(V5)$, the authors proved the existence of normalized solutions for Eq. (1.4) for any given a > 0. Li and Zou [Reference Li and Zou35] recently studied the case where  $V(x)=-\frac{\mu}{|x|^2}$ and
$V(x)=-\frac{\mu}{|x|^2}$ and  $f(u)=|u|^{2^*-2} u+\nu|u|^{p-2} u$, with
$f(u)=|u|^{2^*-2} u+\nu|u|^{p-2} u$, with ![]() $2 \lt p \lt 2^*$, in Eq. (1.4), which can be expressed as:
$2 \lt p \lt 2^*$, in Eq. (1.4), which can be expressed as:
 \begin{align}
\begin{cases}
-\Delta u-\frac{\mu}{|x|^2} u=\lambda u+|u|^{2^*-2} u+\nu|u|^{p-2}, & N \geq 3,\\
\int_{\mathbb{R}^N} |u|^2\mathrm{d} x =a, & u \in H^{1}\left(\mathbb{R}^N\right),
\end{cases}
\end{align}
\begin{align}
\begin{cases}
-\Delta u-\frac{\mu}{|x|^2} u=\lambda u+|u|^{2^*-2} u+\nu|u|^{p-2}, & N \geq 3,\\
\int_{\mathbb{R}^N} |u|^2\mathrm{d} x =a, & u \in H^{1}\left(\mathbb{R}^N\right),
\end{cases}
\end{align} and then found several existence results of normalized ground state solutions when ![]() $\nu \geq 0$ and non-existence results when
$\nu \geq 0$ and non-existence results when ![]() $\nu \leq 0$. Furthermore, they also consider the asymptotic behaviour of the normalized solutions as µ → 0 or ν → 0. For further findings on Eq. (1.4), please refer to [Reference Ikoma and Miyamoto26, Reference Zhou, Zhang, Zhang and Zhou52] and the corresponding references. We also note that Bieganowski, Mederski, and Schino [Reference Bieganowski, Mederski and Schino12] obtained the existence of normalized solutions for the following singular polyharmonic equation
$\nu \leq 0$. Furthermore, they also consider the asymptotic behaviour of the normalized solutions as µ → 0 or ν → 0. For further findings on Eq. (1.4), please refer to [Reference Ikoma and Miyamoto26, Reference Zhou, Zhang, Zhang and Zhou52] and the corresponding references. We also note that Bieganowski, Mederski, and Schino [Reference Bieganowski, Mederski and Schino12] obtained the existence of normalized solutions for the following singular polyharmonic equation
 \begin{align*}
\begin{cases}
(-\Delta)^m u + \frac{\mu}{|y|^{2m}} u + \lambda u = g(u), \quad x = (y, z) \in \mathbb{R}^K \times \mathbb{R}^{N-K}, \\
\int_{\mathbb{R}^N} |u|^2 \, dx = \rho \gt 0,
\end{cases}
\end{align*}
\begin{align*}
\begin{cases}
(-\Delta)^m u + \frac{\mu}{|y|^{2m}} u + \lambda u = g(u), \quad x = (y, z) \in \mathbb{R}^K \times \mathbb{R}^{N-K}, \\
\int_{\mathbb{R}^N} |u|^2 \, dx = \rho \gt 0,
\end{cases}
\end{align*}where g is Sobolev subcritical growth at infinity.
Motivated by the previous studies, we find ourselves inclined to extend our exploration into the realm of normalized solutions for Eq. (1.4) with Hardy potential. Specifically, we investigate the following equation
 \begin{align}
\begin{cases}
-\Delta u -\mu\frac{u}{|x|^2}+\lambda u =f(u), \quad &\text{in}\quad \mathbb{R}^N\backslash\{0\},\\
\int_{\mathbb{R}^{N}}|u|^{2}\mathrm{d} x=a,&u\in H^1(\mathbb{R}^{N}),
\end{cases}
\end{align}
\begin{align}
\begin{cases}
-\Delta u -\mu\frac{u}{|x|^2}+\lambda u =f(u), \quad &\text{in}\quad \mathbb{R}^N\backslash\{0\},\\
\int_{\mathbb{R}^{N}}|u|^{2}\mathrm{d} x=a,&u\in H^1(\mathbb{R}^{N}),
\end{cases}
\end{align} where ![]() $N\geq 3$,
$N\geq 3$, ![]() $\lambda \in \mathbb{R}$,
$\lambda \in \mathbb{R}$,  $\frac{1}{|x|^2} $ is the Hardy potential,
$\frac{1}{|x|^2} $ is the Hardy potential,  $\mu \lt \bar{\mu}:=\frac{(N-2)^2}{4}$, and f satisfies the following conditions:
$\mu \lt \bar{\mu}:=\frac{(N-2)^2}{4}$, and f satisfies the following conditions:
(F1) ![]() $f\in C^{1}\left(\mathbb{R},\mathbb{R}\right)$ and odd.
$f\in C^{1}\left(\mathbb{R},\mathbb{R}\right)$ and odd.
(F2) There exist β, η such that  $\limsup \limits_{|s| \rightarrow 0} \frac{F(s)}{|s|^{2+4/N}}=\beta\in[0,\infty)$ and
$\limsup \limits_{|s| \rightarrow 0} \frac{F(s)}{|s|^{2+4/N}}=\beta\in[0,\infty)$ and  $\lim \limits_{|s| \rightarrow \infty} \frac{f(s)s}{|s|^{2^{*}}}=2^*\eta \gt 0$.
$\lim \limits_{|s| \rightarrow \infty} \frac{f(s)s}{|s|^{2^{*}}}=2^*\eta \gt 0$.
(F3)  $ \frac{\widetilde{F}(s)}{|s|^{2+\frac{4}{N}}}$ is strictly increasing on
$ \frac{\widetilde{F}(s)}{|s|^{2+\frac{4}{N}}}$ is strictly increasing on ![]() $(0,+\infty)$, where
$(0,+\infty)$, where  $\widetilde{F}(s)=f(s) s-2 F(s)$.
$\widetilde{F}(s)=f(s) s-2 F(s)$.
(F4) ![]() $f(s)s \lt 2^*F(s)$ for s ≠ 0.
$f(s)s \lt 2^*F(s)$ for s ≠ 0.
(F5) There exist constants ![]() $2+4/N \lt p \lt 2^*$ and κ > 0 such that
$2+4/N \lt p \lt 2^*$ and κ > 0 such that
 \begin{align*}
F(s) \geq \frac{\kappa}{p}|s|^{p}.
\end{align*}
\begin{align*}
F(s) \geq \frac{\kappa}{p}|s|^{p}.
\end{align*}The primary focus of this problem is not only the Sobolev critical growth nonlinear term but also the presence of the so-called ‘Hardy potential’ (or ‘inverse-square potential’) in the linear part. The potential with this rate of decay is critical in non-relativistic quantum mechanics, as they represent an inter-mediate threshold between regular potentials (for which there are ordinary stationary states) and singular potentials (for which the energy is not lower-bounded and the particle falls to the centre), for more details see [Reference Ghoussoub and Robert22]. Besides, it also arises in many other areas such as nuclear physics, molecular physics, and quantum cosmology (see [Reference Berestycki and Esteban9, Reference Buryak, Di Trapani, Skryabin and Trillo14, Reference Guo and Mederski23, Reference Reed and Simon41]).
The Gagliardo–Nirenberg inequality is crucial to this study. For ![]() $2 \lt p \lt 2^*$, the inequality is given by:
$2 \lt p \lt 2^*$, the inequality is given by:
 \begin{align}
|u|_p \leq C_{N, p}|\nabla u|_2^{\gamma_p}|u|_2^{1-\gamma_p} \text {for } u \in H^1\left(\mathbb{R}^N\right),
\end{align}
\begin{align}
|u|_p \leq C_{N, p}|\nabla u|_2^{\gamma_p}|u|_2^{1-\gamma_p} \text {for } u \in H^1\left(\mathbb{R}^N\right),
\end{align} where ![]() $C_{N, p} \gt 0$ represents the optimal constant, and
$C_{N, p} \gt 0$ represents the optimal constant, and  $\gamma_p=N\left(\frac{1}{2}-\frac{1}{p}\right)$. Additionally,
$\gamma_p=N\left(\frac{1}{2}-\frac{1}{p}\right)$. Additionally, ![]() $p\gamma_p \gt 2$ holds if and only if
$p\gamma_p \gt 2$ holds if and only if  $p \gt \bar{p} :=2+\frac{4}{N}$.
$p \gt \bar{p} :=2+\frac{4}{N}$.
We introduce that the corresponding energy functional is of class C 1 in ![]() $H^1\left(\mathbb{R}^N\right)$:
$H^1\left(\mathbb{R}^N\right)$:
 \begin{align*}
\mathcal{I}_\mu(u)=\frac{1}{2} \int_{\mathbb{R}^N}|\nabla u|^2-\mu\frac{u^2}{|x|^2}\mathrm{d} x -\int_{\mathbb{R}^N} F(u) \mathrm{d} x.
\end{align*}
\begin{align*}
\mathcal{I}_\mu(u)=\frac{1}{2} \int_{\mathbb{R}^N}|\nabla u|^2-\mu\frac{u^2}{|x|^2}\mathrm{d} x -\int_{\mathbb{R}^N} F(u) \mathrm{d} x.
\end{align*} We say that ![]() $v \in S_a$ is the normalized ground state solution to Eq. (1.6) if it is a solution of Eq. (1.6) that minimizes the value of
$v \in S_a$ is the normalized ground state solution to Eq. (1.6) if it is a solution of Eq. (1.6) that minimizes the value of ![]() $ \mathcal{I}_\mu$ among all the normalized solutions of (1.6). Namely, if
$ \mathcal{I}_\mu$ among all the normalized solutions of (1.6). Namely, if
 \begin{equation*}
\left.d\mathcal{I}_\mu\right|_{S_a}(v)
=0 \quad \text {and } \quad \mathcal{I}_\mu(v)=\inf \left\{\mathcal{I}_\mu(v):\left.d\mathcal{I}_\mu\right|_{S_a}(u)=0, u \in S_a\right\}.
\end{equation*}
\begin{equation*}
\left.d\mathcal{I}_\mu\right|_{S_a}(v)
=0 \quad \text {and } \quad \mathcal{I}_\mu(v)=\inf \left\{\mathcal{I}_\mu(v):\left.d\mathcal{I}_\mu\right|_{S_a}(u)=0, u \in S_a\right\}.
\end{equation*} Since the functional ![]() $ \mathcal{I}_\mu$ remains unbounded from below on Sa, we therefore introduce the manifold
$ \mathcal{I}_\mu$ remains unbounded from below on Sa, we therefore introduce the manifold
where ![]() $P_\mu(u)$ is defined as
$P_\mu(u)$ is defined as
 \begin{align*}
P_\mu(u)=\int_{\mathbb{R}^N}|\nabla u|^2-\mu\frac{u^2}{|x|^2}
\mathrm{d} x-\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F} \left(u \right)
\mathrm{d} x.
\end{align*}
\begin{align*}
P_\mu(u)=\int_{\mathbb{R}^N}|\nabla u|^2-\mu\frac{u^2}{|x|^2}
\mathrm{d} x-\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F} \left(u \right)
\mathrm{d} x.
\end{align*} It is a widely acknowledged fact that any critical point of ![]() $\left. \mathcal{I}_\mu\right|_{S_a}$ is a member of
$\left. \mathcal{I}_\mu\right|_{S_a}$ is a member of ![]() $\mathcal{M}_\mu(a)$, from an implication of the Pohožaev identity. Furthermore, we delve into the exploration of the minimizing problem
$\mathcal{M}_\mu(a)$, from an implication of the Pohožaev identity. Furthermore, we delve into the exploration of the minimizing problem
 \begin{align*}
m_\mu(a)=\inf _{u \in \mathcal{M}_\mu(a)} \mathcal{I}_\mu(u).
\end{align*}
\begin{align*}
m_\mu(a)=\inf _{u \in \mathcal{M}_\mu(a)} \mathcal{I}_\mu(u).
\end{align*}We will now delineate the main result of this article.
Theorem 1.1. Assume that ![]() $N\geq 3$,
$N\geq 3$, ![]() $\bar{\mu} \gt \mu \gt 0$, a > 0,
$\bar{\mu} \gt \mu \gt 0$, a > 0,  $1-\frac{\mu}{\bar{\mu}} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and
$1-\frac{\mu}{\bar{\mu}} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and ![]() $(F1)$ –
$(F1)$ – ![]() $(F5)$ hold. Then, there exists
$(F5)$ hold. Then, there exists ![]() $\kappa^* \gt 0$, such that for any
$\kappa^* \gt 0$, such that for any ![]() $\kappa \geq \kappa^*$ (κ is given in
$\kappa \geq \kappa^*$ (κ is given in ![]() $(F5)$), Eq. (1.6) possesses a normalized ground state solution
$(F5)$), Eq. (1.6) possesses a normalized ground state solution ![]() $\left(u, \lambda\right)$, where u > 0 is radial and λ > 0.
$\left(u, \lambda\right)$, where u > 0 is radial and λ > 0.
The solution derived from theorem 1.1 is exponential decay at infinity and potentially blow-up at the origin. This property is stated in the following proposition.
Proposition 1.2. Let ![]() $(u,\lambda)$ be the solution obtained in theorem 1.1. Then
$(u,\lambda)$ be the solution obtained in theorem 1.1. Then
(i)
 $u \in C^2\left(\mathbb{R}^N\backslash \{0\} \right)$.
$u \in C^2\left(\mathbb{R}^N\backslash \{0\} \right)$.(ii) There exist constants C > 0 and R > 0 such that for
 $|\alpha| \leq 2$,
$|\alpha| \leq 2$,
 \begin{equation*}
\left|D^\alpha u(x)\right| \leq C \exp \left(-\sqrt{\frac{1}{2}}|x|\right), \text {for }|x| \geq R.
\end{equation*}
\begin{equation*}
\left|D^\alpha u(x)\right| \leq C \exp \left(-\sqrt{\frac{1}{2}}|x|\right), \text {for }|x| \geq R.
\end{equation*}(iii) There exist constants
 $C_{r,1} \gt 0$ and
$C_{r,1} \gt 0$ and  $C_{r,2} \gt 0$ depend on a sufficiently small r > 0 such that
$C_{r,2} \gt 0$ depend on a sufficiently small r > 0 such that
 \begin{equation*}
C_{r,2}|x|^{-\sqrt{\bar{\mu}}+\sqrt{\bar{\mu}-\mu}} \leq |u(x)| \leq C_{r,1}|x|^{-\sqrt{\bar{\mu}}+\sqrt{\bar{\mu}-\mu}},
\quad \text {for } x \in B_r \backslash\{0\} .
\end{equation*}
\begin{equation*}
C_{r,2}|x|^{-\sqrt{\bar{\mu}}+\sqrt{\bar{\mu}-\mu}} \leq |u(x)| \leq C_{r,1}|x|^{-\sqrt{\bar{\mu}}+\sqrt{\bar{\mu}-\mu}},
\quad \text {for } x \in B_r \backslash\{0\} .
\end{equation*}
In fact, the limiting equation derived from Eq. (1.6) is as follows
 \begin{align}
\begin{cases}
-\Delta u+\lambda u=f(u) & \text {in } \mathbb{R}^N, \\
\int_{\mathbb{R}^N}|u|^2 \mathrm{d} x=a,& u \in H^1\left(\mathbb{R}^N\right),
\end{cases}
\end{align}
\begin{align}
\begin{cases}
-\Delta u+\lambda u=f(u) & \text {in } \mathbb{R}^N, \\
\int_{\mathbb{R}^N}|u|^2 \mathrm{d} x=a,& u \in H^1\left(\mathbb{R}^N\right),
\end{cases}
\end{align} and the associated energy functional ![]() $\mathcal{I}_{\infty}: H^1\left(\mathbb{R}^N\right) \rightarrow \mathbb{R}$ for Eq. (1.8) is
$\mathcal{I}_{\infty}: H^1\left(\mathbb{R}^N\right) \rightarrow \mathbb{R}$ for Eq. (1.8) is
 \begin{equation*}
\mathcal{I}_{\infty}(u)=\frac{1}{2} \int_{\mathbb{R}^N}|\nabla u|^2 \mathrm{d} x-\int_{\mathbb{R}^N} F(u) \mathrm{d} x.
\end{equation*}
\begin{equation*}
\mathcal{I}_{\infty}(u)=\frac{1}{2} \int_{\mathbb{R}^N}|\nabla u|^2 \mathrm{d} x-\int_{\mathbb{R}^N} F(u) \mathrm{d} x.
\end{equation*}Any solution u of Eq. (1.6) belongs to the manifold
where ![]() $P_{\infty}(u)$ is defined as
$P_{\infty}(u)$ is defined as
 \begin{equation*}
P_{\infty}(u)=\int_{\mathbb{R}^N}|\nabla u|^2 \mathrm{d} x-\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F}(u) \mathrm{d} x.
\end{equation*}
\begin{equation*}
P_{\infty}(u)=\int_{\mathbb{R}^N}|\nabla u|^2 \mathrm{d} x-\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F}(u) \mathrm{d} x.
\end{equation*}Furthermore, we define
 \begin{equation*}
m_{\infty}(a)=\inf _{u \in \mathcal{M}_{\infty}(a)} \mathcal{I}_\mu(u).
\end{equation*}
\begin{equation*}
m_{\infty}(a)=\inf _{u \in \mathcal{M}_{\infty}(a)} \mathcal{I}_\mu(u).
\end{equation*} We then scrutinize the behaviour of solutions as the parameter ![]() $\mu\to0^+$ and derive the existence of solutions for the limiting case, i.e. Eq. (1.8).
$\mu\to0^+$ and derive the existence of solutions for the limiting case, i.e. Eq. (1.8).
Theorem 1.3. Assume that ![]() $N\geq 3$,
$N\geq 3$, ![]() $\bar{\mu} \gt \mu \gt 0$, a > 0,
$\bar{\mu} \gt \mu \gt 0$, a > 0,  $1-\frac{\mu}{\bar{\mu}} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and
$1-\frac{\mu}{\bar{\mu}} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and ![]() $(F1)$–
$(F1)$–![]() $(F5)$ hold. Let
$(F5)$ hold. Let ![]() $\left\{\left(u_{\mu_n}, \lambda_{\mu_n}\right)\right\}$ in theorem 1.1 with
$\left\{\left(u_{\mu_n}, \lambda_{\mu_n}\right)\right\}$ in theorem 1.1 with ![]() $\mu_n \rightarrow 0^+$, then
$\mu_n \rightarrow 0^+$, then ![]() $u_{\mu_n} \rightarrow u$ in
$u_{\mu_n} \rightarrow u$ in ![]() $H_r^1\left(\mathbb{R}^N\right)$ and
$H_r^1\left(\mathbb{R}^N\right)$ and ![]() $\lambda_{\mu_n} \rightarrow \lambda \gt 0$ as
$\lambda_{\mu_n} \rightarrow \lambda \gt 0$ as ![]() $\mu_n\to0^+$. Moreover,
$\mu_n\to0^+$. Moreover, ![]() $(u, \lambda)$ is a normalized ground state solution of Eq. (1.8).
$(u, \lambda)$ is a normalized ground state solution of Eq. (1.8).
Furthermore, we study the existence of solutions for µ < 0.
Theorem 1.4. Assume that ![]() $N\geq 3$,
$N\geq 3$, ![]() $0 \gt \mu$, a > 0,
$0 \gt \mu$, a > 0,  $1 \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$ and
$1 \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$ and ![]() $(F1)$–
$(F1)$–![]() $(F5)$ hold. Then
$(F5)$ hold. Then ![]() $ m_\mu(a)=m_{\infty}(a)$ and
$ m_\mu(a)=m_{\infty}(a)$ and ![]() $m_\mu(a)$ cannot be achieved. Furthermore, if κ is sufficiently large, Eq. (1.6) admits a mountain pass solution
$m_\mu(a)$ cannot be achieved. Furthermore, if κ is sufficiently large, Eq. (1.6) admits a mountain pass solution ![]() $\left(u, \lambda\right)\in H_r^1\left(\mathbb{R}^N\right)
\times \mathbb{R}^{+} $ with u > 0, whose energy is strictly greater than
$\left(u, \lambda\right)\in H_r^1\left(\mathbb{R}^N\right)
\times \mathbb{R}^{+} $ with u > 0, whose energy is strictly greater than ![]() $m_{\mu}(a)$.
$m_{\mu}(a)$.
Remark 1.5. In the case without a mass constraint, when µ < 0, there is no ground state, as demonstrated in [Reference Lions37, theorem 1.1].
It is also of significant interest to investigate the asymptotic behaviour of solutions as ![]() $\mu\rightarrow 0^-$. Consequently, we present the following theorem.
$\mu\rightarrow 0^-$. Consequently, we present the following theorem.
Theorem 1.6. Assume that ![]() $N\geq 3$,
$N\geq 3$, ![]() $0 \gt \mu$, a > 0,
$0 \gt \mu$, a > 0,  $1 \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and
$1 \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and ![]() $(F1)$–
$(F1)$–![]() $(F5)$ hold. Let the positive and radial sequence of solutions
$(F5)$ hold. Let the positive and radial sequence of solutions ![]() $\left\{\left(u_{\mu_n}, \lambda_{\mu_n}\right)\right\}$ in theorem 1.4 with
$\left\{\left(u_{\mu_n}, \lambda_{\mu_n}\right)\right\}$ in theorem 1.4 with ![]() $\mu_n \rightarrow 0^-$, then
$\mu_n \rightarrow 0^-$, then ![]() $u_{\mu_n} \rightarrow u$ in
$u_{\mu_n} \rightarrow u$ in ![]() $H_r^1\left(\mathbb{R}^N\right)$ and
$H_r^1\left(\mathbb{R}^N\right)$ and ![]() $\lambda_{\mu_n} \rightarrow \lambda \gt 0$ as
$\lambda_{\mu_n} \rightarrow \lambda \gt 0$ as ![]() $\mu_n\to0^-$. Moreover,
$\mu_n\to0^-$. Moreover, ![]() $(u, \lambda)$ is a normalized ground state solution of Eq. (1.8).
$(u, \lambda)$ is a normalized ground state solution of Eq. (1.8).
Proposition 1.7. Let u be a solution obtained in either theorem 1.3, theorem 1.4, or theorem 1.6. Then, it can be inferred that ![]() $u \in C^2\left(\mathbb{R}^N \right)$, and there exist C > 0 and R > 0 such that for
$u \in C^2\left(\mathbb{R}^N \right)$, and there exist C > 0 and R > 0 such that for ![]() $|\alpha| \leq 2$,
$|\alpha| \leq 2$,
 \begin{equation*}
\left|D^\alpha u(x)\right| \leq C \exp \left(-\sqrt{\frac{1}{2}}|x|\right) \text {for all }|x| \geq R .
\end{equation*}
\begin{equation*}
\left|D^\alpha u(x)\right| \leq C \exp \left(-\sqrt{\frac{1}{2}}|x|\right) \text {for all }|x| \geq R .
\end{equation*}Remark 1.8. To illustrate the existence of nonlinear functions that satisfy ![]() $\left(F1\right)$–
$\left(F1\right)$–![]() $\left(F5\right)$, we provide the following example:
$\left(F5\right)$, we provide the following example:
 \begin{align*}
F(s)=\beta |s|^{2+\frac{4}{N}}+\eta |s|^{2^*}+\frac{\kappa}{p}|s|^{p},
\end{align*}
\begin{align*}
F(s)=\beta |s|^{2+\frac{4}{N}}+\eta |s|^{2^*}+\frac{\kappa}{p}|s|^{p},
\end{align*} where ![]() $2+4/N \lt p \lt 2^*$.
$2+4/N \lt p \lt 2^*$.
The article is structured as follows: In §2, we give a foundation of preliminary concepts and lemmas that will be invoked in subsequent proofs, including the proof of theorem 1.1. The proofs of theorems 1.3, 1.4, and 1.6 are delineated in §3, 4, and 5, respectively.
Notation. Throughout the article, we use the following notations:
•
 $H^1\left(\mathbb{R}^N\right)$ denotes the Sobolev space equipped with the norm
$H^1\left(\mathbb{R}^N\right)$ denotes the Sobolev space equipped with the norm
 \begin{align*}
\|u\|=\left(\int_{\mathbb{R}^N}(\left|\nabla u\right|^2+u^2) \mathrm{d} x\right)^{\frac{1}{2}}.
\end{align*}
\begin{align*}
\|u\|=\left(\int_{\mathbb{R}^N}(\left|\nabla u\right|^2+u^2) \mathrm{d} x\right)^{\frac{1}{2}}.
\end{align*}•
 $H_r^1\left(\mathbb{R}^N\right):=\left\{u \in H^1\left(\mathbb{R}^N\right): u \text{~is the radial function} \right\}$.
$H_r^1\left(\mathbb{R}^N\right):=\left\{u \in H^1\left(\mathbb{R}^N\right): u \text{~is the radial function} \right\}$.•
 $L^p\left(\mathbb{R}^N\right)(1 \leq p\leq\infty)$ denotes the Lebesgue space with the norm
$L^p\left(\mathbb{R}^N\right)(1 \leq p\leq\infty)$ denotes the Lebesgue space with the norm
 \begin{align*}
|u|_p=\left(\int_{\mathbb{R}^N}|u|^p \mathrm{d} x\right)^{\frac{1}{p}},\quad |u|_{\infty}=\operatorname{ess} \sup _{x \in \mathbb{R}^N}|u(x)|.
\end{align*}
\begin{align*}
|u|_p=\left(\int_{\mathbb{R}^N}|u|^p \mathrm{d} x\right)^{\frac{1}{p}},\quad |u|_{\infty}=\operatorname{ess} \sup _{x \in \mathbb{R}^N}|u(x)|.
\end{align*}•
 $B_r(0):=\left\{x \in \mathbb{R}^N:|x| \lt r\right\}$.
$B_r(0):=\left\{x \in \mathbb{R}^N:|x| \lt r\right\}$.•
 $S_{r,a}:=\left\{u\in H_r^1(\mathbb{R}^N):\int_{\mathbb{R}^{N}}|u|^2 \mathrm{d} x=a\right\}$.
$S_{r,a}:=\left\{u\in H_r^1(\mathbb{R}^N):\int_{\mathbb{R}^{N}}|u|^2 \mathrm{d} x=a\right\}$.•
 $\mathcal{D}^{1,2}\left(\mathbb{R}^N\right):=\left\{u \in L^{2^*}\left(\mathbb{R}^N\right)
:\frac{\partial u}{\partial x_i} \in L^2\left(\mathbb{R}^N\right), i=1,2, \ldots, N\right\}$.
$\mathcal{D}^{1,2}\left(\mathbb{R}^N\right):=\left\{u \in L^{2^*}\left(\mathbb{R}^N\right)
:\frac{\partial u}{\partial x_i} \in L^2\left(\mathbb{R}^N\right), i=1,2, \ldots, N\right\}$.•
 $\mathbb{R}^+:=\left\{\alpha\in \mathbb{R}: \alpha \gt 0 \right\}$.
$\mathbb{R}^+:=\left\{\alpha\in \mathbb{R}: \alpha \gt 0 \right\}$.• C denotes a positive constant and is possibly various in different places.
2. Preliminaries
For any ![]() $N\geq 3$ and
$N\geq 3$ and ![]() $\mu \in(0, \bar{\mu})$, we define
$\mu \in(0, \bar{\mu})$, we define
 \begin{align}
S_\mu:=\inf _{u \in \mathcal{D}^{1,2}\left(\mathbb{R}^N\right) \backslash\{0\}}
\frac{\int_{\mathbb{R}^N}|\nabla u|^2-\frac{\mu}{|x|^2} u^2 \mathrm{d} x}
{\left(\int_{\mathbb{R}^N}|u|^{2^*} \mathrm{d} x\right)^{2 / 2^*}},
\end{align}
\begin{align}
S_\mu:=\inf _{u \in \mathcal{D}^{1,2}\left(\mathbb{R}^N\right) \backslash\{0\}}
\frac{\int_{\mathbb{R}^N}|\nabla u|^2-\frac{\mu}{|x|^2} u^2 \mathrm{d} x}
{\left(\int_{\mathbb{R}^N}|u|^{2^*} \mathrm{d} x\right)^{2 / 2^*}},
\end{align}as in [Reference Chen, Li and Li16, Reference Ferrero and Gazzola19]. In particular, when µ = 0, we define
 \begin{align}
S:=\inf _{u \in \mathcal{D}^{1,2}\left(\mathbb{R}^N\right) \backslash\{0\}}
\frac{\int_{\mathbb{R}^N}|\nabla u|^2\mathrm{d} x}
{\left(\int_{\mathbb{R}^N}|u|^{2^*} \mathrm{d} x\right)^{2 / 2^*}},
\end{align}
\begin{align}
S:=\inf _{u \in \mathcal{D}^{1,2}\left(\mathbb{R}^N\right) \backslash\{0\}}
\frac{\int_{\mathbb{R}^N}|\nabla u|^2\mathrm{d} x}
{\left(\int_{\mathbb{R}^N}|u|^{2^*} \mathrm{d} x\right)^{2 / 2^*}},
\end{align} see [Reference Talenti47]. Both (2.1) and (2.2) lead to the formulation of an inequality known as the Sobolev inequality. For ![]() $2 \lt p \lt 2^*$, we recall the Gagliardo–Nirenberg inequality as
$2 \lt p \lt 2^*$, we recall the Gagliardo–Nirenberg inequality as
 \begin{align*}
|u|_p \leq C_{N, p}|\nabla u|_2^{\gamma_p}|u|_2^{1-\gamma_p} \text{ for } u \in H^1\left(\mathbb{R}^N\right),
\end{align*}
\begin{align*}
|u|_p \leq C_{N, p}|\nabla u|_2^{\gamma_p}|u|_2^{1-\gamma_p} \text{ for } u \in H^1\left(\mathbb{R}^N\right),
\end{align*} where ![]() $C_{N, p} \gt 0$ represents the optimal constant,
$C_{N, p} \gt 0$ represents the optimal constant,  $\gamma_p=N\left(\frac{1}{2}-\frac{1}{p}\right)$, and
$\gamma_p=N\left(\frac{1}{2}-\frac{1}{p}\right)$, and ![]() $p\gamma_p \gt 2$ holds if and only if
$p\gamma_p \gt 2$ holds if and only if  $p \gt \bar{p}=2+\frac{4}{N}$.
$p \gt \bar{p}=2+\frac{4}{N}$.
Lemma 2.1. Assume that ![]() $N \geq 3$, and
$N \geq 3$, and ![]() $(F1)$–
$(F1)$–![]() $(F5)$ hold. Then there exists c > 0 such that
$(F5)$ hold. Then there exists c > 0 such that
 \begin{equation*}
f(s) s- \bar{p} F(s) \gt c|s|^{2^*} \quad \text {for all } s \neq 0.
\end{equation*}
\begin{equation*}
f(s) s- \bar{p} F(s) \gt c|s|^{2^*} \quad \text {for all } s \neq 0.
\end{equation*}Proof. By [Reference Jeanjean and Lu31, lemma 2.3], we have
where  $\bar{p}=2+\frac{4}{N}$. We claim that
$\bar{p}=2+\frac{4}{N}$. We claim that  $\liminf_{s\rightarrow 0}\frac{f(s) s- \bar{p} F(s)}{|s|^{2^*}} \gt 0$. Assume, for the sake of contradiction, that
$\liminf_{s\rightarrow 0}\frac{f(s) s- \bar{p} F(s)}{|s|^{2^*}} \gt 0$. Assume, for the sake of contradiction, that  $\liminf_{s\rightarrow 0}\frac{f(s) s- \bar{p} F(s)}{|s|^{2^*}} =0$. Since f is an odd function, we can deduce that
$\liminf_{s\rightarrow 0}\frac{f(s) s- \bar{p} F(s)}{|s|^{2^*}} =0$. Since f is an odd function, we can deduce that
 \begin{align}
\liminf_{s\rightarrow 0}\frac{\frac{d}{ds}\left(F(s)/s^{\bar{p}}\right)}{\frac{d}{ds}s^{2^*-p}} =0.
\end{align}
\begin{align}
\liminf_{s\rightarrow 0}\frac{\frac{d}{ds}\left(F(s)/s^{\bar{p}}\right)}{\frac{d}{ds}s^{2^*-p}} =0.
\end{align} From (2.4), it follows that  $\liminf_{s\rightarrow 0}\frac{F(s)}{s^{2^*}} =0$, which contradicts
$\liminf_{s\rightarrow 0}\frac{F(s)}{s^{2^*}} =0$, which contradicts ![]() $(F5)$. Therefore, we conclude that
$(F5)$. Therefore, we conclude that
 \begin{equation*}\liminf_{s\rightarrow 0}\frac{f(s) s- \bar{p} F(s)}{s^{2^*}} \gt 0.\end{equation*}
\begin{equation*}\liminf_{s\rightarrow 0}\frac{f(s) s- \bar{p} F(s)}{s^{2^*}} \gt 0.\end{equation*} This implies there exist ![]() $c_1 \gt 0$ and δ > 0, such that
$c_1 \gt 0$ and δ > 0, such that
 \begin{align*}
\frac{f(s) s- \bar{p} F(s)}{s^{2^*}} \geq \frac{c_1}{2}, ~~s\in(0,\delta).
\end{align*}
\begin{align*}
\frac{f(s) s- \bar{p} F(s)}{s^{2^*}} \geq \frac{c_1}{2}, ~~s\in(0,\delta).
\end{align*} Furthermore, by ![]() $(F1)$,
$(F1)$, ![]() $(F2)$, and (2.3), we have
$(F2)$, and (2.3), we have
 \begin{equation*}\frac{f(s) s- \bar{p} F(s)}{s^{2^*}}\in C([\delta,\infty),\mathbb{R}^+)\end{equation*}
\begin{equation*}\frac{f(s) s- \bar{p} F(s)}{s^{2^*}}\in C([\delta,\infty),\mathbb{R}^+)\end{equation*}with
 \begin{equation*}\lim_{s\rightarrow \infty}\frac{f(s) s- \bar{p} F(s)}{s^{2^*}} =\left(2^*-\bar{p}\right)\eta \gt 0.\end{equation*}
\begin{equation*}\lim_{s\rightarrow \infty}\frac{f(s) s- \bar{p} F(s)}{s^{2^*}} =\left(2^*-\bar{p}\right)\eta \gt 0.\end{equation*} Therefore, there exists ![]() $c_2 \gt 0$ such that
$c_2 \gt 0$ such that  $\frac{f(s) s- \bar{p} F(s)}{s^{2^*}} \geq c_2$ on
$\frac{f(s) s- \bar{p} F(s)}{s^{2^*}} \geq c_2$ on ![]() $[\delta,\infty)$. Then there exists c > 0 such that
$[\delta,\infty)$. Then there exists c > 0 such that
 \begin{equation*}
f(s) s- \bar{p} F(s) \gt c|s|^{2^*} \quad \text {for all } s \neq 0.
\end{equation*}
\begin{equation*}
f(s) s- \bar{p} F(s) \gt c|s|^{2^*} \quad \text {for all } s \neq 0.
\end{equation*}This concludes this proof.
For convenience, we define
 \begin{align}
t \star u = t^{\frac{N}{2}}u(tx), \quad \text{for any } x \in \mathbb{R}^N, ~~t\in \mathbb{R}^{+}.
\end{align}
\begin{align}
t \star u = t^{\frac{N}{2}}u(tx), \quad \text{for any } x \in \mathbb{R}^N, ~~t\in \mathbb{R}^{+}.
\end{align} It is straightforward to verify that ![]() $|t \star u|_2 = |u|_2$ for every t > 0. Specifically,
$|t \star u|_2 = |u|_2$ for every t > 0. Specifically, ![]() $u \in S_a$, then
$u \in S_a$, then ![]() $t \star u \in S_a$ for any t > 0.
$t \star u \in S_a$ for any t > 0.
Lemma 2.2. Assume that ![]() $N\geq 3$, a > 0,
$N\geq 3$, a > 0, ![]() $\bar{\mu} \gt \mu$,
$\bar{\mu} \gt \mu$,  $\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and
$\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and ![]() $(F1)$–
$(F1)$–![]() $(F5)$ hold. Then, for any
$(F5)$ hold. Then, for any ![]() $u \in S_a$,
$u \in S_a$,
(a)
 $\mathcal{I}_\mu(t \star u) \rightarrow 0^+$ as
$\mathcal{I}_\mu(t \star u) \rightarrow 0^+$ as  $t \rightarrow 0^+$,
$t \rightarrow 0^+$,(b)
 $\mathcal{I}_\mu(t \star u) \rightarrow-\infty$ as
$\mathcal{I}_\mu(t \star u) \rightarrow-\infty$ as  $t \rightarrow+\infty$.
$t \rightarrow+\infty$.
Proof. We recall the Hardy inequality as presented in [Reference Bahouri, Chemin and Danchin3, theorem 1.72]:
 \begin{align}
\int_{\mathbb{R}^N} \frac{u^2}{|x|^2} \mathrm{d} x \leq \frac{1}{\bar{\mu}} \int_{\mathbb{R}^N}|\nabla u|^2 \mathrm{d} x.
\end{align}
\begin{align}
\int_{\mathbb{R}^N} \frac{u^2}{|x|^2} \mathrm{d} x \leq \frac{1}{\bar{\mu}} \int_{\mathbb{R}^N}|\nabla u|^2 \mathrm{d} x.
\end{align} By ![]() $(F2)$, for any δ > 0, there exists
$(F2)$, for any δ > 0, there exists ![]() $C_{\delta,\eta} \gt \eta$ such that
$C_{\delta,\eta} \gt \eta$ such that
 \begin{align}
F(s) \leq \left(\delta+\beta\right)|s|^{\bar{p}}+C_{\delta,\eta}|s|^{2^*}, \quad \text{for all~} s \in \mathbb{R}.
\end{align}
\begin{align}
F(s) \leq \left(\delta+\beta\right)|s|^{\bar{p}}+C_{\delta,\eta}|s|^{2^*}, \quad \text{for all~} s \in \mathbb{R}.
\end{align} From (1.7), (2.6), (2.7), and ![]() $(F5)$, we derive that
$(F5)$, we derive that
 \begin{align*}
\mathcal{I}_\mu(t \star u)
&\geq \frac{t^2}{2}\min\left\{1,\left(1-\frac{\mu}{\bar{\mu}}\right)\right\} |\nabla u|^2_2-(\delta+\beta) t^{2} |u|^{\bar{p}}_{\bar{p}}
-C_{\delta,\eta} t^{2^*}| u|^{2^*}_{2^*}\\
&\geq \left(\frac{1}{2}\min\left\{1,\left(1-\frac{\mu}{\bar{\mu}}\right)\right\} -C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)\right) t^2|\nabla u|^2_2
-C_{\delta,\eta} t^{2^*}| u|^{2^*}_{2^*},
\end{align*}
\begin{align*}
\mathcal{I}_\mu(t \star u)
&\geq \frac{t^2}{2}\min\left\{1,\left(1-\frac{\mu}{\bar{\mu}}\right)\right\} |\nabla u|^2_2-(\delta+\beta) t^{2} |u|^{\bar{p}}_{\bar{p}}
-C_{\delta,\eta} t^{2^*}| u|^{2^*}_{2^*}\\
&\geq \left(\frac{1}{2}\min\left\{1,\left(1-\frac{\mu}{\bar{\mu}}\right)\right\} -C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)\right) t^2|\nabla u|^2_2
-C_{\delta,\eta} t^{2^*}| u|^{2^*}_{2^*},
\end{align*}and
 \begin{align*}
\mathcal{I}_\mu(t \star u) \leq \frac{t^2}{2}
\max\left\{1,\left(1-\frac{\mu}{\bar{\mu}}\right)\right\}
|\nabla u|^2_2
-\frac{\kappa}{p} t^{p\gamma_p} |u|_p^p.
\end{align*}
\begin{align*}
\mathcal{I}_\mu(t \star u) \leq \frac{t^2}{2}
\max\left\{1,\left(1-\frac{\mu}{\bar{\mu}}\right)\right\}
|\nabla u|^2_2
-\frac{\kappa}{p} t^{p\gamma_p} |u|_p^p.
\end{align*} The conclusion can be drawn that, given the condition ![]() $\bar{\mu} \gt \mu$,
$\bar{\mu} \gt \mu$, ![]() $p \gt \bar{p}$, and a sufficiently small δ,
$p \gt \bar{p}$, and a sufficiently small δ,
This concludes the proof.
Lemma 2.3. Assume that ![]() $N\geq 3$,
$N\geq 3$, ![]() $\bar{\mu} \gt \mu$,
$\bar{\mu} \gt \mu$,  $\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and
$\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and ![]() $(F1)$–
$(F1)$–![]() $(F4)$ hold. Then, for any
$(F4)$ hold. Then, for any ![]() $u \in S_a$, there exists a unique
$u \in S_a$, there exists a unique ![]() $t_u \gt 0$ such that
$t_u \gt 0$ such that ![]() $t_u \star u \in \mathcal{M}_\mu(a)$. Moreover,
$t_u \star u \in \mathcal{M}_\mu(a)$. Moreover, ![]() $ \mathcal{I}_\mu\left(t_u \star u\right) \gt \mathcal{I}_\mu(t \star u)$ for any
$ \mathcal{I}_\mu\left(t_u \star u\right) \gt \mathcal{I}_\mu(t \star u)$ for any ![]() $ t \gt 0 \,\text {with } t \neq t_u$.
$ t \gt 0 \,\text {with } t \neq t_u$.
Proof. For any ![]() $u \in S_a$, we have
$u \in S_a$, we have
 \begin{align*}
\mathcal{I}_\mu\left(t \star u\right)&=\frac{t^2}{2} \int_{\mathbb{R}^N}|\nabla u|^2
- \frac{\mu}{|x|^2}u^2 \mathrm{d} x-\int_{\mathbb{R}^N} F\left(t \star u \right) \mathrm{d} x,
\end{align*}
\begin{align*}
\mathcal{I}_\mu\left(t \star u\right)&=\frac{t^2}{2} \int_{\mathbb{R}^N}|\nabla u|^2
- \frac{\mu}{|x|^2}u^2 \mathrm{d} x-\int_{\mathbb{R}^N} F\left(t \star u \right) \mathrm{d} x,
\end{align*}and
 \begin{align}
P_\mu\left(t \star u\right)&=t^2 \int_{\mathbb{R}^N}|\nabla u|^2
-\frac{\mu}{|x|^2}u^2 \mathrm{d} x-\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F} \left(t \star u \right) \mathrm{d} x \nonumber \\
&=t^2\left(\int_{\mathbb{R}^N}|\nabla u|^2
-\frac{\mu}{|x|^2}u^2 \mathrm{d} x
-\frac{N}{2} \int_{\mathbb{R}^N} \frac{\widetilde{F}\left(t^{\frac{N}{2}} u\right)}{\left|t^{\frac{N}{2}} u\right|^{\bar{p}}}|u|^{\bar{p}} \mathrm{d} x\right).
\end{align}
\begin{align}
P_\mu\left(t \star u\right)&=t^2 \int_{\mathbb{R}^N}|\nabla u|^2
-\frac{\mu}{|x|^2}u^2 \mathrm{d} x-\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F} \left(t \star u \right) \mathrm{d} x \nonumber \\
&=t^2\left(\int_{\mathbb{R}^N}|\nabla u|^2
-\frac{\mu}{|x|^2}u^2 \mathrm{d} x
-\frac{N}{2} \int_{\mathbb{R}^N} \frac{\widetilde{F}\left(t^{\frac{N}{2}} u\right)}{\left|t^{\frac{N}{2}} u\right|^{\bar{p}}}|u|^{\bar{p}} \mathrm{d} x\right).
\end{align} It is evident that ![]() $ \mathcal{I}_\mu\left(t \star u\right)$ is of class C 1, and its derivative can be expressed as
$ \mathcal{I}_\mu\left(t \star u\right)$ is of class C 1, and its derivative can be expressed as
 \begin{align*}
\begin{split}
\frac{d}{d t} \mathcal{I}_\mu\left(t \star u\right)=t \int_{\mathbb{R}^N}|\nabla u|^2
- \frac{\mu}{|x|^2}u^2 \mathrm{d} x
-\frac{N}{2} t^{-1} \int_{\mathbb{R}^N}
\widetilde{F}\left( t \star u \right) \mathrm{d} x
=\frac{1}{t}P_\mu(t \star u).
\end{split}
\end{align*}
\begin{align*}
\begin{split}
\frac{d}{d t} \mathcal{I}_\mu\left(t \star u\right)=t \int_{\mathbb{R}^N}|\nabla u|^2
- \frac{\mu}{|x|^2}u^2 \mathrm{d} x
-\frac{N}{2} t^{-1} \int_{\mathbb{R}^N}
\widetilde{F}\left( t \star u \right) \mathrm{d} x
=\frac{1}{t}P_\mu(t \star u).
\end{split}
\end{align*}With the application of (2.6),
 \begin{align*}
t^2 \int_{\mathbb{R}^N}|\nabla u|^2
-\frac{\mu}{|x|^2}u^2 \mathrm{d} x\geq t^2\min\left\{1,1-\frac{\mu}{\bar{\mu}} \right\}|\nabla u|_2^2.
\end{align*}
\begin{align*}
t^2 \int_{\mathbb{R}^N}|\nabla u|^2
-\frac{\mu}{|x|^2}u^2 \mathrm{d} x\geq t^2\min\left\{1,1-\frac{\mu}{\bar{\mu}} \right\}|\nabla u|_2^2.
\end{align*} By ![]() $(F4),(F5)$, and (2.7), one gets
$(F4),(F5)$, and (2.7), one gets
 \begin{align*}
\begin{split}
\frac{N}{2}\int_{\mathbb{R}^N} \widetilde{F} \left( t \star u\right)\mathrm{d} x & \lt 2^* \int_{\mathbb{R}^N}F\left( t \star u \right)\mathrm{d} x\\
&\leq 2^* \left( (\delta+\beta) t^2 \int_{\mathbb{R}^N} | u|^{\bar{p}}\mathrm{d} x +C_{\delta,\eta} t^{2^*} \int_{\mathbb{R}^N}| u|^{2^*}\mathrm{d} x\right)\\
&=2^*
\left( C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)t^2
\left|\nabla u\right|_2^{2}+C_2t^{2^*}
\left|\nabla u\right|_2^{2^*}\right).
\end{split}
\end{align*}
\begin{align*}
\begin{split}
\frac{N}{2}\int_{\mathbb{R}^N} \widetilde{F} \left( t \star u\right)\mathrm{d} x & \lt 2^* \int_{\mathbb{R}^N}F\left( t \star u \right)\mathrm{d} x\\
&\leq 2^* \left( (\delta+\beta) t^2 \int_{\mathbb{R}^N} | u|^{\bar{p}}\mathrm{d} x +C_{\delta,\eta} t^{2^*} \int_{\mathbb{R}^N}| u|^{2^*}\mathrm{d} x\right)\\
&=2^*
\left( C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)t^2
\left|\nabla u\right|_2^{2}+C_2t^{2^*}
\left|\nabla u\right|_2^{2^*}\right).
\end{split}
\end{align*}It is apparent that by selecting a sufficiently small δ, we can ensure that:
 \begin{align*}
2^* C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta) \lt \min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\}.
\end{align*}
\begin{align*}
2^* C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta) \lt \min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\}.
\end{align*} Thus  $\frac{d}{d t} \mathcal{I}_\mu\left(t \star u\right)(t) \gt 0$ for sufficiently small t. Similar to lemma 2.2, we can conclude that
$\frac{d}{d t} \mathcal{I}_\mu\left(t \star u\right)(t) \gt 0$ for sufficiently small t. Similar to lemma 2.2, we can conclude that  $\frac{d}{d t} \mathcal{I}_\mu\left(t \star u\right)(t)\to-\infty$ as
$\frac{d}{d t} \mathcal{I}_\mu\left(t \star u\right)(t)\to-\infty$ as ![]() $t\to \infty$. Therefore, there exists at least one
$t\to \infty$. Therefore, there exists at least one ![]() $t_{u}\in \mathbb{R}^{+}$ such that
$t_{u}\in \mathbb{R}^{+}$ such that  $\frac{d}{d t} \mathcal{I}_\mu\left(t \star u\right)(t)=\frac{1}{t_u}P_\mu(t_u \star u)=0$, namely,
$\frac{d}{d t} \mathcal{I}_\mu\left(t \star u\right)(t)=\frac{1}{t_u}P_\mu(t_u \star u)=0$, namely, ![]() $ t_{u} \star u\in \mathcal{M}_\mu(a)$.
$ t_{u} \star u\in \mathcal{M}_\mu(a)$.
Suppose that there exists another ![]() $t_{u_1}$ such that
$t_{u_1}$ such that ![]() $t_{u_1} \star u\in \mathcal{M}_\mu(a)$. Combined with (2.8), it yields
$t_{u_1} \star u\in \mathcal{M}_\mu(a)$. Combined with (2.8), it yields
 \begin{align*}
\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(t_{u}^{\frac{N}{2}} u\right)}
{\left|t_{u}^{\frac{N}{2}} u\right|^{\bar{p}}
}|u|^{\bar{p}} \mathrm{d} x
=\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(t_{u_1}^{\frac{N}{2}} u\right)}
{\left|t_{u_1}^{\frac{N}{2}} u\right|^{\bar{p}}}|u|^{\bar{p}} \mathrm{d} x,
\end{align*}
\begin{align*}
\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(t_{u}^{\frac{N}{2}} u\right)}
{\left|t_{u}^{\frac{N}{2}} u\right|^{\bar{p}}
}|u|^{\bar{p}} \mathrm{d} x
=\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(t_{u_1}^{\frac{N}{2}} u\right)}
{\left|t_{u_1}^{\frac{N}{2}} u\right|^{\bar{p}}}|u|^{\bar{p}} \mathrm{d} x,
\end{align*} which contradicts ![]() $(F3)$. Hence, it is established that
$(F3)$. Hence, it is established that ![]() $t_{u}=t_{u_1}$. Furthermore, we can deduce that
$t_{u}=t_{u_1}$. Furthermore, we can deduce that ![]() $ \mathcal{I}_\mu\left(t_{u} \star u\right) \gt \mathcal{I}_\mu(t \star u)$ for all t > 0 with
$ \mathcal{I}_\mu\left(t_{u} \star u\right) \gt \mathcal{I}_\mu(t \star u)$ for all t > 0 with ![]() $t \neq t_u$.
$t \neq t_u$.
Lemma 2.4. Assume that ![]() $N\geq 3$,
$N\geq 3$, ![]() $\bar{\mu} \gt \mu$, a > 0,
$\bar{\mu} \gt \mu$, a > 0,  $ \min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and
$ \min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and ![]() $(F1)$–
$(F1)$–![]() $(F5)$ hold. Then,
$(F5)$ hold. Then,
(i) there exists ρ > 0, such that
 $ \inf \limits_{u\in\mathcal{M}_\mu(a)}|\nabla u|_2 \gt \rho$,
$ \inf \limits_{u\in\mathcal{M}_\mu(a)}|\nabla u|_2 \gt \rho$,(ii)
 $m_\mu(a)= \inf \limits_{u\in\mathcal{M}_\mu(a)} \mathcal{I}_\mu(u) \gt 0.$
$m_\mu(a)= \inf \limits_{u\in\mathcal{M}_\mu(a)} \mathcal{I}_\mu(u) \gt 0.$
Proof. (i) For any ![]() $u \in \mathcal{M}_\mu(a)$, combined with (1.7), (2.6), (2.7), and the Sobolev inequality,
$u \in \mathcal{M}_\mu(a)$, combined with (1.7), (2.6), (2.7), and the Sobolev inequality, ![]() $P_\mu(u)=0$ implies that
$P_\mu(u)=0$ implies that
 \begin{align*}
|\nabla u|_2^2 & =\int_{\mathbb{R}^N}\frac{\mu}{|x|^2}u^2\mathrm{d} x
+\frac{N}{2}
\int_{\mathbb{R}^N}\widetilde{F}(u)\mathrm{d} x \\
& \leq \max \left\{0, \frac{\mu}{\bar{\mu}}\right\}|\nabla u|_2^2+2^* \int_{\mathbb{R}^N} F(u) \mathrm{d} x \\
& \leq \max \left\{0, \frac{\mu}{\bar{\mu}}\right\}|\nabla u|_2^2+2^*
\left( C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)
\left|\nabla u\right|_2^{2}+C_2
\left|\nabla u\right|_2^{2^*}\right).
\end{align*}
\begin{align*}
|\nabla u|_2^2 & =\int_{\mathbb{R}^N}\frac{\mu}{|x|^2}u^2\mathrm{d} x
+\frac{N}{2}
\int_{\mathbb{R}^N}\widetilde{F}(u)\mathrm{d} x \\
& \leq \max \left\{0, \frac{\mu}{\bar{\mu}}\right\}|\nabla u|_2^2+2^* \int_{\mathbb{R}^N} F(u) \mathrm{d} x \\
& \leq \max \left\{0, \frac{\mu}{\bar{\mu}}\right\}|\nabla u|_2^2+2^*
\left( C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)
\left|\nabla u\right|_2^{2}+C_2
\left|\nabla u\right|_2^{2^*}\right).
\end{align*}Let δ enough small such that
 \begin{align}
2^*C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta) \lt \min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\}.
\end{align}
\begin{align}
2^*C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta) \lt \min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\}.
\end{align} Then there is ρ > 0 such that  $\inf \limits_{u\in\mathcal{M}_\mu(a)}|\nabla u|_2 \gt \rho$.
$\inf \limits_{u\in\mathcal{M}_\mu(a)}|\nabla u|_2 \gt \rho$.
(ii) For any ![]() $u \in \mathcal{M}_\mu(a)$, we can deduce that
$u \in \mathcal{M}_\mu(a)$, we can deduce that
 \begin{align*}
\mathcal{I}_\mu(u) \geq \mathcal{I}_\mu(t \star u) &
\geq \frac{t^2}{2}\min \left\{1, 1-\frac{\mu}{\bar{\mu}}\right\}|\nabla u|_2^2
- \left(t^2 (\delta+\beta) | u|_{\bar{p}}^{\bar{p}} +C_{\delta,\eta} t^{2^*}| u|_{2^*}^{2^*} \right) \\
& \geq \frac{t^2}{2}\min \left\{1, 1-\frac{\mu}{\bar{\mu}}\right\}|\nabla u|_2^2
\\ &\quad -C_2 t^{2^*}|\nabla u|_2^{2^*}-C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)t^2|\nabla u|_2^{2}.
\end{align*}
\begin{align*}
\mathcal{I}_\mu(u) \geq \mathcal{I}_\mu(t \star u) &
\geq \frac{t^2}{2}\min \left\{1, 1-\frac{\mu}{\bar{\mu}}\right\}|\nabla u|_2^2
- \left(t^2 (\delta+\beta) | u|_{\bar{p}}^{\bar{p}} +C_{\delta,\eta} t^{2^*}| u|_{2^*}^{2^*} \right) \\
& \geq \frac{t^2}{2}\min \left\{1, 1-\frac{\mu}{\bar{\mu}}\right\}|\nabla u|_2^2
\\ &\quad -C_2 t^{2^*}|\nabla u|_2^{2^*}-C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)t^2|\nabla u|_2^{2}.
\end{align*} By selecting  $t=\frac{\sigma}{|\nabla u|_2}$ with sufficiently small σ > 0 and taking δ sufficiently small to ensure that (2.9) holds, we can deduce that
$t=\frac{\sigma}{|\nabla u|_2}$ with sufficiently small σ > 0 and taking δ sufficiently small to ensure that (2.9) holds, we can deduce that
 \begin{align*}
\mathcal{I}_\mu(u)\geq \frac{\min \left\{1, 1-\frac{\mu}{\bar{\mu}}\right\}-2C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}\beta}{4}\sigma^2 \gt 0.
\end{align*}
\begin{align*}
\mathcal{I}_\mu(u)\geq \frac{\min \left\{1, 1-\frac{\mu}{\bar{\mu}}\right\}-2C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}\beta}{4}\sigma^2 \gt 0.
\end{align*}This concludes the proof.
Corollary 2.5. Assume that ![]() $N\geq 3$,
$N\geq 3$, ![]() $\bar{\mu} \gt \mu$, a > 0,
$\bar{\mu} \gt \mu$, a > 0,  $\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and
$\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and ![]() $(F1)$–
$(F1)$–![]() $(F5)$ hold. Then, there exists a sufficiently small ξ > 0 such that
$(F5)$ hold. Then, there exists a sufficiently small ξ > 0 such that
 \begin{equation*}
m_\mu(a) \gt \sup_{u\in\overline{C(a)}} \mathcal{I}_\mu(u) \gt 0, \quad \mathcal{I}_\mu(u) \gt 0, P_\mu(u) \gt 0~~ \mathrm{for~ any}~ u \in \overline{C(a)},
\end{equation*}
\begin{equation*}
m_\mu(a) \gt \sup_{u\in\overline{C(a)}} \mathcal{I}_\mu(u) \gt 0, \quad \mathcal{I}_\mu(u) \gt 0, P_\mu(u) \gt 0~~ \mathrm{for~ any}~ u \in \overline{C(a)},
\end{equation*} where ![]() $C(a)=\left\{u \in S_a:|\nabla u|_2^2 \lt \xi\right\}$. Furthermore,
$C(a)=\left\{u \in S_a:|\nabla u|_2^2 \lt \xi\right\}$. Furthermore, ![]() $ \mathcal{I}_\mu$ has a mountain pass geometry.
$ \mathcal{I}_\mu$ has a mountain pass geometry.
Proof. By ![]() $(F4)$, (1.7), (2.7), and the Sobolev inequality, we have
$(F4)$, (1.7), (2.7), and the Sobolev inequality, we have
 \begin{align*}
P_\mu(u)&=\int_{\mathbb{R}^N}|\nabla u|^2-\mu\frac{u^2}{|x|^2}
\mathrm{d} x-\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F} \left(u \right)
\mathrm{d} x\\
&\geq \int_{\mathbb{R}^N}|\nabla u|^2-\mu\frac{u^2}{|x|^2}
\mathrm{d} x-2^*\int_{\mathbb{R}^N}F \left(u \right)
\mathrm{d} x\\
&\geq \min \left\{1, 1-\frac{\mu}{\bar{\mu}}\right\}|\nabla u|_2^2 -2^*
\left( C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)
\left|\nabla u\right|_2^{2}+C_2
\left|\nabla u\right|_2^{2^*}\right).
\end{align*}
\begin{align*}
P_\mu(u)&=\int_{\mathbb{R}^N}|\nabla u|^2-\mu\frac{u^2}{|x|^2}
\mathrm{d} x-\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F} \left(u \right)
\mathrm{d} x\\
&\geq \int_{\mathbb{R}^N}|\nabla u|^2-\mu\frac{u^2}{|x|^2}
\mathrm{d} x-2^*\int_{\mathbb{R}^N}F \left(u \right)
\mathrm{d} x\\
&\geq \min \left\{1, 1-\frac{\mu}{\bar{\mu}}\right\}|\nabla u|_2^2 -2^*
\left( C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)
\left|\nabla u\right|_2^{2}+C_2
\left|\nabla u\right|_2^{2^*}\right).
\end{align*} Thus, ![]() $P_\mu(u) \gt 0$ when
$P_\mu(u) \gt 0$ when ![]() $u \in \overline{C(a)}$ if ξ > 0 is sufficiently small. Similarly, we can obtain that
$u \in \overline{C(a)}$ if ξ > 0 is sufficiently small. Similarly, we can obtain that ![]() $ \mathcal{I}_\mu(u) \gt 0$ when
$ \mathcal{I}_\mu(u) \gt 0$ when ![]() $u \in \overline{C(a)}$ if ξ > 0 is sufficiently small. By
$u \in \overline{C(a)}$ if ξ > 0 is sufficiently small. By ![]() $(F5)$, one can see that
$(F5)$, one can see that
 \begin{align*}
\mathcal{I}_\mu(u)\leq \left(\frac{1}{2}-\frac{1}{\bar{\mu}}\min\{\mu,0\}\right)\int_{\mathbb{R}^N}|\nabla u|^2
\mathrm{d} x,
\end{align*}
\begin{align*}
\mathcal{I}_\mu(u)\leq \left(\frac{1}{2}-\frac{1}{\bar{\mu}}\min\{\mu,0\}\right)\int_{\mathbb{R}^N}|\nabla u|^2
\mathrm{d} x,
\end{align*} which implies that  $m_\mu(a) \gt \sup_{u\in\overline{C(a)}} \mathcal{I}_\mu(u)$ for ξ > 0 small enough. Combined with lemma (2.2),
$m_\mu(a) \gt \sup_{u\in\overline{C(a)}} \mathcal{I}_\mu(u)$ for ξ > 0 small enough. Combined with lemma (2.2), ![]() $ \mathcal{I}_\mu$ has a mountain pass geometry.
$ \mathcal{I}_\mu$ has a mountain pass geometry.
Set
 \begin{equation*}
\sigma_\mu(a):=\inf _{\gamma \in \Gamma} \max _{t\in[0,1]} \mathcal{I}_\mu(\gamma(t)),
\end{equation*}
\begin{equation*}
\sigma_\mu(a):=\inf _{\gamma \in \Gamma} \max _{t\in[0,1]} \mathcal{I}_\mu(\gamma(t)),
\end{equation*}where
 \begin{equation*}
\Gamma_{\mu}:=\left\{\gamma \in C\left([0,1], S_{r,a}\right): \gamma(0) \in \overline{C(a)}, ~\mathcal{I}_\mu(\gamma(1)) \leq 0\right\}.
\end{equation*}
\begin{equation*}
\Gamma_{\mu}:=\left\{\gamma \in C\left([0,1], S_{r,a}\right): \gamma(0) \in \overline{C(a)}, ~\mathcal{I}_\mu(\gamma(1)) \leq 0\right\}.
\end{equation*} Following from the strategy in [Reference Jeanjean27], consider the functional  $
\widetilde{\mathcal{I}}_\mu: \mathbb{R}^+ \times H^1\left(\mathbb{R}^N\right) \rightarrow \mathbb{R},
$
$
\widetilde{\mathcal{I}}_\mu: \mathbb{R}^+ \times H^1\left(\mathbb{R}^N\right) \rightarrow \mathbb{R},
$
 \begin{align}
\widetilde{\mathcal{I}}_\mu(s, u):=\mathcal{I}_\mu(s \star u)=\frac{s^2}{2}\int_{\mathbb{R}^N}|\nabla u|^2
-\frac{\mu}{|x|^2}u^2 \mathrm{d} x -s^{-N} \int_{\mathbb{R}^N}F(s^{\frac{N}{2}}u) \mathrm{d} x.
\end{align}
\begin{align}
\widetilde{\mathcal{I}}_\mu(s, u):=\mathcal{I}_\mu(s \star u)=\frac{s^2}{2}\int_{\mathbb{R}^N}|\nabla u|^2
-\frac{\mu}{|x|^2}u^2 \mathrm{d} x -s^{-N} \int_{\mathbb{R}^N}F(s^{\frac{N}{2}}u) \mathrm{d} x.
\end{align} Define ![]() $ \mathcal{I}_\mu^c:=\{u \in S_{r,a}: \mathcal{I}_\mu(u) \leq c\}$, and
$ \mathcal{I}_\mu^c:=\{u \in S_{r,a}: \mathcal{I}_\mu(u) \leq c\}$, and
 \begin{equation*}
\widetilde{\sigma}_\mu(a):=\inf _{\gamma \in \Gamma} \max _{t \in [0,1]} \widetilde{\mathcal{I}}_\mu(\widetilde{\gamma}(t)),
\end{equation*}
\begin{equation*}
\widetilde{\sigma}_\mu(a):=\inf _{\gamma \in \Gamma} \max _{t \in [0,1]} \widetilde{\mathcal{I}}_\mu(\widetilde{\gamma}(t)),
\end{equation*}where
 \begin{equation*}
\widetilde{\Gamma}_{\mu}:=\left\{\widetilde{\gamma}=(\iota,\zeta) \in C\left([0,1], \mathbb{R}^+
\times S_{r,a}\right): \widetilde{\gamma}(0) \in\left(1, \overline{C(a)}\right), \widetilde{\gamma}(1) \in\left(1, \mathcal{I}_\mu^0\right)\right\}.
\end{equation*}
\begin{equation*}
\widetilde{\Gamma}_{\mu}:=\left\{\widetilde{\gamma}=(\iota,\zeta) \in C\left([0,1], \mathbb{R}^+
\times S_{r,a}\right): \widetilde{\gamma}(0) \in\left(1, \overline{C(a)}\right), \widetilde{\gamma}(1) \in\left(1, \mathcal{I}_\mu^0\right)\right\}.
\end{equation*} For any ![]() $u \in S_{r,a}$, since
$u \in S_{r,a}$, since ![]() $|\nabla(s \star u)|_2 \rightarrow 0$ as
$|\nabla(s \star u)|_2 \rightarrow 0$ as ![]() $s \rightarrow 0$, and
$s \rightarrow 0$, and ![]() $ \mathcal{I}_\mu(s \star u) \rightarrow -\infty$ as
$ \mathcal{I}_\mu(s \star u) \rightarrow -\infty$ as ![]() $s \rightarrow+\infty$, there exist
$s \rightarrow+\infty$, there exist ![]() $0 \lt s_0 \lt 1 \lt s_1$ such that
$0 \lt s_0 \lt 1 \lt s_1$ such that
where ![]() $\widetilde{\gamma}_u$ is continuous [Reference Bartsch and Soave7, lemma 3.5] and hence forms a path in
$\widetilde{\gamma}_u$ is continuous [Reference Bartsch and Soave7, lemma 3.5] and hence forms a path in ![]() $\widetilde{\Gamma}_{\mu}$. Then
$\widetilde{\Gamma}_{\mu}$. Then ![]() $\widetilde{\sigma}_\mu(a)$ is well-defined.
$\widetilde{\sigma}_\mu(a)$ is well-defined.
Lemma 2.6. Assume that ![]() $N\geq 3$,
$N\geq 3$, ![]() $\bar{\mu} \gt \mu$, a > 0,
$\bar{\mu} \gt \mu$, a > 0,  $\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and
$\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and ![]() $(F1){-}(F5)$ hold. Then
$(F1){-}(F5)$ hold. Then
 \begin{equation*}
\widetilde{\sigma}_\mu(a)=m^r_\mu(a) :=\inf _{u \in \mathcal{M}_\mu^r(a)} \mathcal{I}_\mu(u),
\end{equation*}
\begin{equation*}
\widetilde{\sigma}_\mu(a)=m^r_\mu(a) :=\inf _{u \in \mathcal{M}_\mu^r(a)} \mathcal{I}_\mu(u),
\end{equation*} where  $\mathcal{M}_\mu^r(a)=\mathcal{M}_\mu(a) \cap H_r^1\left(\mathbb{R}^N\right)$.
$\mathcal{M}_\mu^r(a)=\mathcal{M}_\mu(a) \cap H_r^1\left(\mathbb{R}^N\right)$.
Proof. Step 1: ![]() $\widetilde{\sigma}_\mu(a) \geq m^r_\mu(a).$ For any
$\widetilde{\sigma}_\mu(a) \geq m^r_\mu(a).$ For any  $\widetilde{\gamma}=(\iota, \zeta) \in \widetilde{\Gamma}_{\mu}$, by lemma 2.3, there exists
$\widetilde{\gamma}=(\iota, \zeta) \in \widetilde{\Gamma}_{\mu}$, by lemma 2.3, there exists ![]() $t_0 \in(0,1)$ such that
$t_0 \in(0,1)$ such that ![]() $\iota(t_0) \star \zeta(t_0) \in \mathcal{M}_\mu^r(a)$. Thus, we have
$\iota(t_0) \star \zeta(t_0) \in \mathcal{M}_\mu^r(a)$. Thus, we have
 \begin{equation*}
\max _{t\in [0,1]} \widetilde{\mathcal{I}}_\mu(\widetilde{\gamma}(t)) \geq \widetilde{\mathcal{I}}_\mu\left(\widetilde{\gamma}\left(t_0\right)\right)
= \mathcal{I}_\mu\left(\iota(t_0) \star \zeta(t_0)\right) \geq \inf _{u\in\mathcal{M}_\mu^r(a)} \mathcal{I}_\mu(u)=m^r_\mu (a).
\end{equation*}
\begin{equation*}
\max _{t\in [0,1]} \widetilde{\mathcal{I}}_\mu(\widetilde{\gamma}(t)) \geq \widetilde{\mathcal{I}}_\mu\left(\widetilde{\gamma}\left(t_0\right)\right)
= \mathcal{I}_\mu\left(\iota(t_0) \star \zeta(t_0)\right) \geq \inf _{u\in\mathcal{M}_\mu^r(a)} \mathcal{I}_\mu(u)=m^r_\mu (a).
\end{equation*} Hence, ![]() $\widetilde{\sigma}_\mu(a) \geq m^r_\mu(a)$.
$\widetilde{\sigma}_\mu(a) \geq m^r_\mu(a)$.
Step 2: ![]() $m^r_\mu(a) \geq \widetilde{\sigma}_\mu(a) $. For any
$m^r_\mu(a) \geq \widetilde{\sigma}_\mu(a) $. For any ![]() $u \in \mathcal{M}_\mu^r(a)$, then
$u \in \mathcal{M}_\mu^r(a)$, then ![]() $\widetilde{\gamma}_u$ defined in (2.11) is a path in
$\widetilde{\gamma}_u$ defined in (2.11) is a path in ![]() $\widetilde{\Gamma}_{\mu}$. By lemma 2.3,
$\widetilde{\Gamma}_{\mu}$. By lemma 2.3,
 \begin{equation*}
\mathcal{I}_\mu(u)=\max _{t\in[0,1]} \widetilde{\mathcal{I}}_\mu(\widetilde{\gamma}_u(t)) \geq \widetilde{\sigma}_\mu(a) .
\end{equation*}
\begin{equation*}
\mathcal{I}_\mu(u)=\max _{t\in[0,1]} \widetilde{\mathcal{I}}_\mu(\widetilde{\gamma}_u(t)) \geq \widetilde{\sigma}_\mu(a) .
\end{equation*} Thus, ![]() $m^r_\mu(a) \geq\widetilde{\sigma}_\mu(a)$.
$m^r_\mu(a) \geq\widetilde{\sigma}_\mu(a)$.
Lemma 2.7. Assume that ![]() $N\geq 3$,
$N\geq 3$, ![]() $\bar{\mu} \gt \mu$, a > 0,
$\bar{\mu} \gt \mu$, a > 0,  $\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$ and
$\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$ and ![]() $(F1)$–
$(F1)$–![]() $(F5)$ hold. Then
$(F5)$ hold. Then
Proof. Step 1: ![]() $\sigma_\mu(a) \geq \widetilde{\sigma}_\mu(a)$. Let
$\sigma_\mu(a) \geq \widetilde{\sigma}_\mu(a)$. Let ![]() $\gamma \in \Gamma_{\mu}$, define
$\gamma \in \Gamma_{\mu}$, define  $\widetilde{\gamma}(t)=(1, \gamma(t)) \in \widetilde{\Gamma}_{\mu}$. Then
$\widetilde{\gamma}(t)=(1, \gamma(t)) \in \widetilde{\Gamma}_{\mu}$. Then  $ \mathcal{I}_\mu(\gamma(t))=\widetilde{\mathcal{I}}_\mu(\widetilde{\gamma}(t))\geq \widetilde{\sigma}_\mu(a)$ for all
$ \mathcal{I}_\mu(\gamma(t))=\widetilde{\mathcal{I}}_\mu(\widetilde{\gamma}(t))\geq \widetilde{\sigma}_\mu(a)$ for all ![]() $t\geq 0$. Hence
$t\geq 0$. Hence ![]() $\sigma_\mu(a) \geq \widetilde{\sigma}_\mu(a)$.
$\sigma_\mu(a) \geq \widetilde{\sigma}_\mu(a)$.
Step 2: ![]() $ \widetilde{\sigma}_\mu(a)\geq \sigma_\mu(a) $. For all
$ \widetilde{\sigma}_\mu(a)\geq \sigma_\mu(a) $. For all  $\widetilde{\gamma}(t)=(\iota(t), \zeta(t)) \in \widetilde{\Gamma}_{\mu}$, setting
$\widetilde{\gamma}(t)=(\iota(t), \zeta(t)) \in \widetilde{\Gamma}_{\mu}$, setting ![]() $\gamma(t)=\iota(t)\star \zeta(t)$, then
$\gamma(t)=\iota(t)\star \zeta(t)$, then ![]() $\gamma \in \Gamma_{\mu}$ and
$\gamma \in \Gamma_{\mu}$ and  $\widetilde{\mathcal{I}}_\mu(\widetilde{\gamma}(t))= \mathcal{I}_\mu(\gamma(t))\geq \sigma_\mu(a)$. Therefore,
$\widetilde{\mathcal{I}}_\mu(\widetilde{\gamma}(t))= \mathcal{I}_\mu(\gamma(t))\geq \sigma_\mu(a)$. Therefore, ![]() $ \widetilde{\sigma}_\mu(a)\geq \sigma_\mu(a) $.
$ \widetilde{\sigma}_\mu(a)\geq \sigma_\mu(a) $.
Lemma 2.8. Assume that ![]() $N\geq 3$,
$N\geq 3$, ![]() $\bar{\mu} \gt \mu\geq0$, a > 0,
$\bar{\mu} \gt \mu\geq0$, a > 0,  $1-\frac{\mu}{\bar{\mu}} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$ and
$1-\frac{\mu}{\bar{\mu}} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$ and ![]() $(F1)$–
$(F1)$–![]() $(F5)$ hold. Then,
$(F5)$ hold. Then,
Proof. It suffices to show that ![]() $m_\mu(a) \geq m^r_\mu(a)$, since
$m_\mu(a) \geq m^r_\mu(a)$, since ![]() $\mathcal{M}_\mu^r(a) \subset \mathcal{M}_\mu(a)$. For any
$\mathcal{M}_\mu^r(a) \subset \mathcal{M}_\mu(a)$. For any ![]() $u \in \mathcal{M}_\mu(a)$, let
$u \in \mathcal{M}_\mu(a)$, let ![]() $v:=|u|^*$ be the Schwarz rearrangement of
$v:=|u|^*$ be the Schwarz rearrangement of ![]() $|u|$. By the properties of rearrangement, one has
$|u|$. By the properties of rearrangement, one has
By lemma 2.3, there exists ![]() $t_v \gt 0$ such that
$t_v \gt 0$ such that ![]() $t_v \star v \in \mathcal{M}_\mu^r(a)$. For any t > 0,
$t_v \star v \in \mathcal{M}_\mu^r(a)$. For any t > 0,
 \begin{equation*}
\begin{aligned}
\mathcal{I}_\mu(t \star v) & =\frac{t^2}{2} \int_{\mathbb{R}^N}|\nabla v|^2-\frac{\mu}{|x|^2}v^2 \mathrm{d} x-t^{-N} \int_{\mathbb{R}^N} F\left(t^{\frac{N}{2}} v\right) \mathrm{d} x \\
& \leq \frac{t^2}{2} \int_{\mathbb{R}^N}|\nabla u|^2-\frac{\mu}{|x|^2}u^2 \mathrm{d} x-t^{-N} \int_{\mathbb{R}^N} F\left(t^{\frac{N}{2}} u\right) \mathrm{d} x \\
& = \mathcal{I}_\mu(t \star u) .
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
\mathcal{I}_\mu(t \star v) & =\frac{t^2}{2} \int_{\mathbb{R}^N}|\nabla v|^2-\frac{\mu}{|x|^2}v^2 \mathrm{d} x-t^{-N} \int_{\mathbb{R}^N} F\left(t^{\frac{N}{2}} v\right) \mathrm{d} x \\
& \leq \frac{t^2}{2} \int_{\mathbb{R}^N}|\nabla u|^2-\frac{\mu}{|x|^2}u^2 \mathrm{d} x-t^{-N} \int_{\mathbb{R}^N} F\left(t^{\frac{N}{2}} u\right) \mathrm{d} x \\
& = \mathcal{I}_\mu(t \star u) .
\end{aligned}
\end{equation*}By lemma 2.3, we obtain
Thus, ![]() $m_\mu(a)=m^r_\mu(a)$.
$m_\mu(a)=m^r_\mu(a)$.
Lemma 2.9. [Reference Bartsch and Soave7, lemma 3.6] Assume that ![]() $N \geq 3$. For any
$N \geq 3$. For any ![]() $u \in S_a$ and t > 0, the map
$u \in S_a$ and t > 0, the map
is a linear isomorphism with inverse
 \begin{equation*}
T_{t \star u} S_a \rightarrow T_u S_a, \quad \psi \mapsto\left(\frac{1}{t}\right) \star \psi.
\end{equation*}
\begin{equation*}
T_{t \star u} S_a \rightarrow T_u S_a, \quad \psi \mapsto\left(\frac{1}{t}\right) \star \psi.
\end{equation*}Lemma 2.10. Assume that ![]() $N \geq 3$, a > 0,
$N \geq 3$, a > 0, ![]() $\bar{\mu} \gt \mu$,
$\bar{\mu} \gt \mu$,  $\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N, \bar{p}}^{\bar{p}}
\beta a^{\frac{2}{N}}$, and
$\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N, \bar{p}}^{\bar{p}}
\beta a^{\frac{2}{N}}$, and ![]() $(F 1)$–
$(F 1)$–![]() $(F 5)$ hold. Then, there exists a Pohožaev–Palais–Smale sequence
$(F 5)$ hold. Then, there exists a Pohožaev–Palais–Smale sequence ![]() $\left\{u_n\right\} \subset S_{r, a}$ for
$\left\{u_n\right\} \subset S_{r, a}$ for ![]() $ \mathcal{I}_\mu$ at the level
$ \mathcal{I}_\mu$ at the level ![]() $\sigma_\mu(a)$,
$\sigma_\mu(a)$,
 \begin{equation*}
\mathcal{I}_\mu\left(u_n\right) \rightarrow \sigma_\mu(a),\quad \left(\left. \mathcal{I}_\mu\right|_{S_{r, a}} \right)
^{\prime}\left(u_n\right) \rightarrow 0, \quad P_\mu\left(u_n\right) \rightarrow 0,
\quad \text {as } n \rightarrow \infty \text {. }
\end{equation*}
\begin{equation*}
\mathcal{I}_\mu\left(u_n\right) \rightarrow \sigma_\mu(a),\quad \left(\left. \mathcal{I}_\mu\right|_{S_{r, a}} \right)
^{\prime}\left(u_n\right) \rightarrow 0, \quad P_\mu\left(u_n\right) \rightarrow 0,
\quad \text {as } n \rightarrow \infty \text {. }
\end{equation*}Proof. By lemmas 2.6 and 2.7, we have
 \begin{equation*}
\sigma_\mu(a)=m^r_\mu(a) \gt \sup_{u\in\left(\overline{C(a)} \cup \mathcal{I}_\mu^0\right) \cap S_{r, a}}
\mathcal{I}_\mu(u)=\sup _{(s,u)\in\left((1, \overline{C(a)}) \cup\left(1, \mathcal{I}_\mu^0\right)\right)
\cap\left(\mathbb{R} \times S_{r, a}\right)} \widetilde{\mathcal{I}}_\mu(s,u).
\end{equation*}
\begin{equation*}
\sigma_\mu(a)=m^r_\mu(a) \gt \sup_{u\in\left(\overline{C(a)} \cup \mathcal{I}_\mu^0\right) \cap S_{r, a}}
\mathcal{I}_\mu(u)=\sup _{(s,u)\in\left((1, \overline{C(a)}) \cup\left(1, \mathcal{I}_\mu^0\right)\right)
\cap\left(\mathbb{R} \times S_{r, a}\right)} \widetilde{\mathcal{I}}_\mu(s,u).
\end{equation*} Using the terminology in [Reference Ghoussoub21, Section 5],  $\{\widetilde{\gamma}([0,1]): \widetilde{\gamma}\in\widetilde{\Gamma}_{\mu}\}$ is a homotopy stable family of compact subsets of
$\{\widetilde{\gamma}([0,1]): \widetilde{\gamma}\in\widetilde{\Gamma}_{\mu}\}$ is a homotopy stable family of compact subsets of ![]() $\mathbb{R} \times S_{r,a}$ with extended closed boundary
$\mathbb{R} \times S_{r,a}$ with extended closed boundary  $\left(1, \overline{C(a)}\right) \cup\left(1, \mathcal{I}_\mu^0\right)$. Furthermore, the superlevel set
$\left(1, \overline{C(a)}\right) \cup\left(1, \mathcal{I}_\mu^0\right)$. Furthermore, the superlevel set  $\{u\in S_{r,a}:\widetilde{\mathcal{I}}_\mu(u) \geq \widetilde{\sigma}_\mu(a)\}$ is a dual set for
$\{u\in S_{r,a}:\widetilde{\mathcal{I}}_\mu(u) \geq \widetilde{\sigma}_\mu(a)\}$ is a dual set for ![]() $\widetilde{\Gamma}_{\mu}$, meaning that
$\widetilde{\Gamma}_{\mu}$, meaning that ![]() $(F{^\prime}1)$ and
$(F{^\prime}1)$ and ![]() $(F{^\prime}2)$ in [Reference Ghoussoub21, theorem 5.2] are satisfied. Therefore, considering any minimizing sequence
$(F{^\prime}2)$ in [Reference Ghoussoub21, theorem 5.2] are satisfied. Therefore, considering any minimizing sequence  $\left\{\gamma_n=\left(1, \zeta_n\right)\right\} \subset \widetilde{\Gamma}_{\mu}$ for
$\left\{\gamma_n=\left(1, \zeta_n\right)\right\} \subset \widetilde{\Gamma}_{\mu}$ for ![]() $\widetilde{\sigma}_\mu(a)$, where
$\widetilde{\sigma}_\mu(a)$, where ![]() $\zeta_n(t) \geq 0$ almost everywhere in
$\zeta_n(t) \geq 0$ almost everywhere in ![]() $\mathbb{R}^N$ for
$\mathbb{R}^N$ for ![]() $t \in[0,1]$, there exists a Palais–Smale sequence
$t \in[0,1]$, there exists a Palais–Smale sequence ![]() $\left\{\left(s_n, w_n\right)\right\} \subset \mathbb{R}^+ \times S_{r,a}$ for
$\left\{\left(s_n, w_n\right)\right\} \subset \mathbb{R}^+ \times S_{r,a}$ for  $\left.\widetilde{\mathcal{I}}_\mu\right|_{\mathbb{R} \times S_{r,a}}$ at level
$\left.\widetilde{\mathcal{I}}_\mu\right|_{\mathbb{R} \times S_{r,a}}$ at level ![]() $\widetilde{\sigma}_\mu(a)$, such that as
$\widetilde{\sigma}_\mu(a)$, such that as ![]() $ n \rightarrow \infty$,
$ n \rightarrow \infty$,
 \begin{align}
\partial_s \widetilde{\mathcal{I}}_\mu\left(s_n, w_n\right) \rightarrow 0,
\end{align}
\begin{align}
\partial_s \widetilde{\mathcal{I}}_\mu\left(s_n, w_n\right) \rightarrow 0,
\end{align}and
 \begin{align}
\left\|\partial_u \widetilde{\mathcal{I}}_\mu\left(s_n, w_n\right)\right\|_{\left(T_{w_n} S_r\right)^*} \rightarrow 0 ,
\end{align}
\begin{align}
\left\|\partial_u \widetilde{\mathcal{I}}_\mu\left(s_n, w_n\right)\right\|_{\left(T_{w_n} S_r\right)^*} \rightarrow 0 ,
\end{align}with the additional property that
By (2.10) and (2.12), we have ![]() $P_\mu\left(s_n \star w_n\right)= o_n(1)$. Also by (2.13) and the boundedness of
$P_\mu\left(s_n \star w_n\right)= o_n(1)$. Also by (2.13) and the boundedness of ![]() $\left\{s_n\right\}$ due to (2.14), we obtain
$\left\{s_n\right\}$ due to (2.14), we obtain
Let ![]() $u_n:=s_n \star w_n$, based on (2.15) and lemma 2.9,
$u_n:=s_n \star w_n$, based on (2.15) and lemma 2.9, ![]() $\left\{u_n\right\} \subset S_{r,a}$ is a Palais–Smale sequence for
$\left\{u_n\right\} \subset S_{r,a}$ is a Palais–Smale sequence for  $\left. \mathcal{I}_\mu\right|_{S_{r,a}}$ at the level
$\left. \mathcal{I}_\mu\right|_{S_{r,a}}$ at the level ![]() $\sigma_\mu(a)$. Moreover,
$\sigma_\mu(a)$. Moreover, ![]() $P_\mu\left(u_n\right)=o_n(1)$.
$P_\mu\left(u_n\right)=o_n(1)$.
Lemma 2.11. Assume that ![]() $N \geq 3$, a > 0,
$N \geq 3$, a > 0, ![]() $\bar{\mu} \gt \mu$,
$\bar{\mu} \gt \mu$,  $\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N, \bar{p}}^{\bar{p}}
\beta a^{\frac{2}{N}}$, and
$\min\left\{1-\frac{\mu}{\bar{\mu}},1 \right\} \gt 2^* C_{N, \bar{p}}^{\bar{p}}
\beta a^{\frac{2}{N}}$, and ![]() $(F 1)$–
$(F 1)$–![]() $(F 5)$ hold. Then for any ϵ > 0, there exists
$(F 5)$ hold. Then for any ϵ > 0, there exists ![]() $\kappa^* \gt 0$ such that
$\kappa^* \gt 0$ such that ![]() $ \sigma_\mu(a) \lt \epsilon$ as
$ \sigma_\mu(a) \lt \epsilon$ as ![]() $\kappa \gt \kappa^*$, where κ appears in
$\kappa \gt \kappa^*$, where κ appears in ![]() $(F5)$.
$(F5)$.
Proof. For a fixed ![]() $u \in S_{r,a}$, there exist
$u \in S_{r,a}$, there exist ![]() $0 \lt s_0 \lt 1 \lt s_1$ such that
$0 \lt s_0 \lt 1 \lt s_1$ such that
By ![]() $(F5)$ and lemmas 2.6–2.8, we observe that
$(F5)$ and lemmas 2.6–2.8, we observe that
 \begin{align*}
\begin{split}
\sigma_\mu(a) &\leq \max _{t\in[0,1]} {\mathcal{I}_\mu}(\gamma_o(t)) \\
& \leq \max _{t \geq 0}\left\{\frac{t^2}{2}
\int_{\mathbb{R}^N}|\nabla u|^2 -\frac{\mu}{|x|^2}u^2 \mathrm{d} x
-\frac{\kappa}{p} t^{p\gamma_p} \int_{\mathbb{R}^N}|u|^p \mathrm{d} x\right\} \\
& \leq \max _{t \geq 0}\left\{\frac{1}{2}\max \left\{1, 1-\frac{\mu}{\bar{\mu}} \right\} t^2
\int_{\mathbb{R}^N}|\nabla u|^2 \mathrm{d} x -\frac{\kappa}{p} t^{p\gamma_p}
\int_{\mathbb{R}^N}|u|^p \mathrm{d} x\right\} \\
& \leq C\left(\frac{1}{\kappa}\right)^{\frac{2}{p\gamma_p-2}},
\end{split}
\end{align*}
\begin{align*}
\begin{split}
\sigma_\mu(a) &\leq \max _{t\in[0,1]} {\mathcal{I}_\mu}(\gamma_o(t)) \\
& \leq \max _{t \geq 0}\left\{\frac{t^2}{2}
\int_{\mathbb{R}^N}|\nabla u|^2 -\frac{\mu}{|x|^2}u^2 \mathrm{d} x
-\frac{\kappa}{p} t^{p\gamma_p} \int_{\mathbb{R}^N}|u|^p \mathrm{d} x\right\} \\
& \leq \max _{t \geq 0}\left\{\frac{1}{2}\max \left\{1, 1-\frac{\mu}{\bar{\mu}} \right\} t^2
\int_{\mathbb{R}^N}|\nabla u|^2 \mathrm{d} x -\frac{\kappa}{p} t^{p\gamma_p}
\int_{\mathbb{R}^N}|u|^p \mathrm{d} x\right\} \\
& \leq C\left(\frac{1}{\kappa}\right)^{\frac{2}{p\gamma_p-2}},
\end{split}
\end{align*} which deduces that ![]() $ \sigma_\mu(a) \lt \epsilon$ for any
$ \sigma_\mu(a) \lt \epsilon$ for any ![]() $\kappa \gt \kappa^*$ by noting that
$\kappa \gt \kappa^*$ by noting that  $p \gt \bar{p} = 2 + \frac{4}{N}$.
$p \gt \bar{p} = 2 + \frac{4}{N}$.
Proof of theorem 1.1
Consider the sequence ![]() $\{u_n\}$ arising from lemma 2.10. As the functional
$\{u_n\}$ arising from lemma 2.10. As the functional ![]() $ \mathcal{I}_\mu$ exhibits even symmetry with respect to u, we can assume un is nonnegative.
$ \mathcal{I}_\mu$ exhibits even symmetry with respect to u, we can assume un is nonnegative.
We claim that ![]() $\{u_n\}$ is bounded in
$\{u_n\}$ is bounded in ![]() $H_{r}^1\left(\mathbb{R}^N\right)$. From
$H_{r}^1\left(\mathbb{R}^N\right)$. From ![]() $(F2)$ and lemma 2.1,
$(F2)$ and lemma 2.1, ![]() $ \mathcal{I}_\mu\left(u_n\right)=\sigma_{\mu}(a)+o_n(1)$ combined with
$ \mathcal{I}_\mu\left(u_n\right)=\sigma_{\mu}(a)+o_n(1)$ combined with ![]() $P_{\mu}\left(u_n\right)=o_n(1)$ implies that there is a small enough c > 0 such that
$P_{\mu}\left(u_n\right)=o_n(1)$ implies that there is a small enough c > 0 such that
 \begin{align*}
\sigma_{\mu}(a)+o_n(1) = \mathcal{I}_\mu\left(u_n\right)-\frac{1}{2}P_{\mu}(u_n)
&= \int_{\mathbb{R}^N}\frac{N}{4} f\left(u_n\right)u_n- \frac{N+2}{2}F\left(u_n\right) \mathrm{d} x \nonumber \\
&\geq c\int_{\mathbb{R}^N}| u_n|^{2^*}\mathrm{d} x.
\end{align*}
\begin{align*}
\sigma_{\mu}(a)+o_n(1) = \mathcal{I}_\mu\left(u_n\right)-\frac{1}{2}P_{\mu}(u_n)
&= \int_{\mathbb{R}^N}\frac{N}{4} f\left(u_n\right)u_n- \frac{N+2}{2}F\left(u_n\right) \mathrm{d} x \nonumber \\
&\geq c\int_{\mathbb{R}^N}| u_n|^{2^*}\mathrm{d} x.
\end{align*} Also from ![]() $P\left(u_n\right)=o_n(1)$, we have
$P\left(u_n\right)=o_n(1)$, we have
 \begin{align*}
\left(1-\frac{\mu}{\bar{\mu}}\right)|\nabla u_n|_2^2 \leq\int_{\mathbb{R}^N}|\nabla u_n|^2-\mu\frac{u_n^2}{|x|^2}
\mathrm{d} x&=\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F} \left(u_n \right)
\mathrm{d} x\\
&\leq
2^* C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)
\left|\nabla u_n\right|_2^{2}+C_2
\left|u_n\right|_{2^*}^{2^*},
\end{align*}
\begin{align*}
\left(1-\frac{\mu}{\bar{\mu}}\right)|\nabla u_n|_2^2 \leq\int_{\mathbb{R}^N}|\nabla u_n|^2-\mu\frac{u_n^2}{|x|^2}
\mathrm{d} x&=\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F} \left(u_n \right)
\mathrm{d} x\\
&\leq
2^* C_{N,\bar{p}}^{\bar{p}}a^{\frac{2}{N}}(\delta+\beta)
\left|\nabla u_n\right|_2^{2}+C_2
\left|u_n\right|_{2^*}^{2^*},
\end{align*} which shows that ![]() $\left\{\left|\nabla u_n\right|_{2}\right\}$ is bounded, and
$\left\{\left|\nabla u_n\right|_{2}\right\}$ is bounded, and ![]() $\left\{u_n\right\} \subset S_{r,a}$, so that
$\left\{u_n\right\} \subset S_{r,a}$, so that ![]() $\{u_n\}$ is bounded in
$\{u_n\}$ is bounded in ![]() $H_{r}^1\left(\mathbb{R}^N\right)$.
$H_{r}^1\left(\mathbb{R}^N\right)$.
Therefore, there exists ![]() $u\in H_r^1\left(\mathbb{R}^N\right)$ such that, up to a subsequence,
$u\in H_r^1\left(\mathbb{R}^N\right)$ such that, up to a subsequence, ![]() $u_n \rightharpoonup u$ in
$u_n \rightharpoonup u$ in ![]() $H_r^1\left(\mathbb{R}^N\right)$,
$H_r^1\left(\mathbb{R}^N\right)$, ![]() $u_n \rightarrow u$ in
$u_n \rightarrow u$ in ![]() $L^{p}\left(\mathbb{R}^N\right)$ for
$L^{p}\left(\mathbb{R}^N\right)$ for ![]() $p\in (2,2^*)$, and
$p\in (2,2^*)$, and ![]() $u_n(x)\rightarrow u(x)$ almost everywhere in
$u_n(x)\rightarrow u(x)$ almost everywhere in ![]() $\mathbb{R}^N$. We claim that u ≠ 0, by contradiction, that u = 0. By utilizing the Strauss inequality [Reference Willem50, lemma 4.5] for the sequence
$\mathbb{R}^N$. We claim that u ≠ 0, by contradiction, that u = 0. By utilizing the Strauss inequality [Reference Willem50, lemma 4.5] for the sequence ![]() $\left\{u_n\right\}$ in
$\left\{u_n\right\}$ in ![]() $H_r^1\left(\mathbb{R}^N\right)$, it follows that
$H_r^1\left(\mathbb{R}^N\right)$, it follows that
 \begin{equation*}
|u_n(x)| \leq C_N|u_n|_2^{\frac{1}{2}}|\nabla u_n|_2^{\frac{1}{2}}|x|^{\frac{1-N}{2}} \text {a.e. on } \mathbb{R}^N.
\end{equation*}
\begin{equation*}
|u_n(x)| \leq C_N|u_n|_2^{\frac{1}{2}}|\nabla u_n|_2^{\frac{1}{2}}|x|^{\frac{1-N}{2}} \text {a.e. on } \mathbb{R}^N.
\end{equation*} Consequently, it can be deduced that ![]() $u_n(x) \rightarrow 0$ as
$u_n(x) \rightarrow 0$ as ![]() $|x| \rightarrow \infty$.
$|x| \rightarrow \infty$.
By using ![]() $(F2)$, we establish
$(F2)$, we establish
 \begin{align*}
\lim_{s\rightarrow \infty}\frac{\frac{N}{2}\widetilde{F}(s)-2^* \eta |s|^{2^*}}{|s|^{2^*}+|s|^{2}} =0, ~ ~
\lim_{s\rightarrow 0}\frac{\frac{N}{2}\widetilde{F}(s)-2^* \eta |s|^{2^*}}{|s|^{2}+|s|^{2^*}} =0.
\end{align*}
\begin{align*}
\lim_{s\rightarrow \infty}\frac{\frac{N}{2}\widetilde{F}(s)-2^* \eta |s|^{2^*}}{|s|^{2^*}+|s|^{2}} =0, ~ ~
\lim_{s\rightarrow 0}\frac{\frac{N}{2}\widetilde{F}(s)-2^* \eta |s|^{2^*}}{|s|^{2}+|s|^{2^*}} =0.
\end{align*} By the boundedness of ![]() $\{| u_n|_{2^*}\}$ and the fact that
$\{| u_n|_{2^*}\}$ and the fact that ![]() $\{u_n\}\in S_{r,a} $, we have
$\{u_n\}\in S_{r,a} $, we have
 \begin{equation*}
\int_{\mathbb{R}^N}\left|u_n\right|^{2}+\left|u_n\right|^{2^*} \mathrm{d} x \leq M, \quad \text{for some positive}~ M.
\end{equation*}
\begin{equation*}
\int_{\mathbb{R}^N}\left|u_n\right|^{2}+\left|u_n\right|^{2^*} \mathrm{d} x \leq M, \quad \text{for some positive}~ M.
\end{equation*}Consequently, by Lions Lemma [Reference Berestycki and Lions10, theorem A.I.], we can say that
 \begin{align}
\lim_{n\to\infty}\int_{\mathbb{R}^N}\frac{N}{2}\widetilde{F}(u_n)-2^* \eta |u_n|^{2^*} \mathrm{d} x=0.
\end{align}
\begin{align}
\lim_{n\to\infty}\int_{\mathbb{R}^N}\frac{N}{2}\widetilde{F}(u_n)-2^* \eta |u_n|^{2^*} \mathrm{d} x=0.
\end{align} Then by ![]() $P(u_n)\to 0$, we can deduces that
$P(u_n)\to 0$, we can deduces that
 \begin{align}
\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2-\mu\frac{u_n^2}{|x|^2} \mathrm{d} x +o_n(1)& =
\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F}(u_n)\mathrm{d} x \nonumber\\
&= 2^* \eta \int_{\mathbb{R}^N}\left| u_n\right|^{2^*}\mathrm{d} x
+\int_{\mathbb{R}^N}\frac{N}{2}\widetilde{F}(u_n)-2^*\eta|u_n|^{2^*}\mathrm{d} x\nonumber \\
& \leq 2^* \eta S_\mu^{\frac{N}{2-N}}\left(\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 -\mu\frac{u_n^2}{|x|^2} \mathrm{d} x\right)^{\frac{N}{N-2}}.
\end{align}
\begin{align}
\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2-\mu\frac{u_n^2}{|x|^2} \mathrm{d} x +o_n(1)& =
\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F}(u_n)\mathrm{d} x \nonumber\\
&= 2^* \eta \int_{\mathbb{R}^N}\left| u_n\right|^{2^*}\mathrm{d} x
+\int_{\mathbb{R}^N}\frac{N}{2}\widetilde{F}(u_n)-2^*\eta|u_n|^{2^*}\mathrm{d} x\nonumber \\
& \leq 2^* \eta S_\mu^{\frac{N}{2-N}}\left(\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 -\mu\frac{u_n^2}{|x|^2} \mathrm{d} x\right)^{\frac{N}{N-2}}.
\end{align}By using lemma 2.4, we can assume that, up to a subsequence,
 \begin{align*}
\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 -\mu\frac{u_n^2}{|x|^2} \mathrm{d} x\rightarrow l^* \gt 0.
\end{align*}
\begin{align*}
\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 -\mu\frac{u_n^2}{|x|^2} \mathrm{d} x\rightarrow l^* \gt 0.
\end{align*} By (2.17), we find that  $l^* \geq \left(2^* \eta\right)^{\frac{2-N}{2}}S_\mu^{\frac{N}{2}}$. Similarly as (2.16), we have
$l^* \geq \left(2^* \eta\right)^{\frac{2-N}{2}}S_\mu^{\frac{N}{2}}$. Similarly as (2.16), we have
 \begin{align*}
\lim_{n\to\infty}\int_{\mathbb{R}^N}f(u_n)u_n-2^*F(u_n) \mathrm{d} x=0.
\end{align*}
\begin{align*}
\lim_{n\to\infty}\int_{\mathbb{R}^N}f(u_n)u_n-2^*F(u_n) \mathrm{d} x=0.
\end{align*}This allows us to derive
 \begin{align*}
m_\mu(a)+o_n(1)&= \mathcal{I}_\mu\left(u_n\right)-\frac{1}{2^*}P_\mu\left(u_n\right)\\
& =\frac{1}{N} \int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 -\mu \frac{u_n^2}{|x|^2}\mathrm{d} x
+\frac{N-2}{4} \int_{\mathbb{R}^N}f(u_n)u_n-2^*F(u_n) \mathrm{d} x\\
& =\frac{1}{N} \int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 -\mu \frac{u_n^2}{|x|^2}\mathrm{d} x+o_n(1)\\
&\geq \frac{1}{N}\left(2^* \eta\right)^{\frac{2-N}{2}}S_\mu^{\frac{N}{2}}+o_n(1),
\end{align*}
\begin{align*}
m_\mu(a)+o_n(1)&= \mathcal{I}_\mu\left(u_n\right)-\frac{1}{2^*}P_\mu\left(u_n\right)\\
& =\frac{1}{N} \int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 -\mu \frac{u_n^2}{|x|^2}\mathrm{d} x
+\frac{N-2}{4} \int_{\mathbb{R}^N}f(u_n)u_n-2^*F(u_n) \mathrm{d} x\\
& =\frac{1}{N} \int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 -\mu \frac{u_n^2}{|x|^2}\mathrm{d} x+o_n(1)\\
&\geq \frac{1}{N}\left(2^* \eta\right)^{\frac{2-N}{2}}S_\mu^{\frac{N}{2}}+o_n(1),
\end{align*} which contradicts lemma 2.11. Hence, ![]() $u\not= 0$. By the weak lower semi-continuity, we deduce
$u\not= 0$. By the weak lower semi-continuity, we deduce
 \begin{align*}
\int_{\mathbb{R}^N}|u|^2 \mathrm{d} x =a_0 \in (0,a].
\end{align*}
\begin{align*}
\int_{\mathbb{R}^N}|u|^2 \mathrm{d} x =a_0 \in (0,a].
\end{align*} Since ![]() $\left\{u_n\right\}$ is a Palais–Smale sequence of
$\left\{u_n\right\}$ is a Palais–Smale sequence of  $\left. \mathcal{I}_\mu\right|_{S_{r, a}}$, there exists
$\left. \mathcal{I}_\mu\right|_{S_{r, a}}$, there exists ![]() $\left\{\lambda_n\right\}$ such that for any
$\left\{\lambda_n\right\}$ such that for any ![]() $\varphi \in H^1\left(\mathbb{R}^N\right)$
$\varphi \in H^1\left(\mathbb{R}^N\right)$
 \begin{align}
\int_{\mathbb{R}^N}\left(\nabla u_n \nabla \varphi-\mu\frac{u_n\varphi }{|x|^2} +\lambda_n u_n
\varphi-f\left(u_n\right) \varphi\right) \mathrm{d} x=o_n(1)\|\varphi\|.
\end{align}
\begin{align}
\int_{\mathbb{R}^N}\left(\nabla u_n \nabla \varphi-\mu\frac{u_n\varphi }{|x|^2} +\lambda_n u_n
\varphi-f\left(u_n\right) \varphi\right) \mathrm{d} x=o_n(1)\|\varphi\|.
\end{align} Setting ![]() $\varphi=u_n$ and by the boundedness of
$\varphi=u_n$ and by the boundedness of ![]() $\left\{u_n\right\}$ in
$\left\{u_n\right\}$ in ![]() $H^1\left(\mathbb{R}^N\right)$, we have
$H^1\left(\mathbb{R}^N\right)$, we have
 \begin{equation*}
-\lambda_n a=\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2-\frac{\mu}{|x|^2}u_n^2 \mathrm{d} x-\int_{\mathbb{R}^N} f\left(u_n\right) u_n \mathrm{d} x+o_n(1) .
\end{equation*}
\begin{equation*}
-\lambda_n a=\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2-\frac{\mu}{|x|^2}u_n^2 \mathrm{d} x-\int_{\mathbb{R}^N} f\left(u_n\right) u_n \mathrm{d} x+o_n(1) .
\end{equation*} Moreover, we can infer that the boundedness of λn by the boundedness of ![]() $\left\{u_n\right\}$. Therefore, up to a subsequence,
$\left\{u_n\right\}$. Therefore, up to a subsequence, ![]() $\lambda_n \rightarrow \lambda \in \mathbb{R}$. By (2.18),
$\lambda_n \rightarrow \lambda \in \mathbb{R}$. By (2.18),
 \begin{align}
\int_{\mathbb{R}^N}(\nabla u \nabla \varphi-\mu\frac{u\varphi }{|x|^2}+\lambda u \varphi-f(u) \varphi) \mathrm{d} x=0
\end{align}
\begin{align}
\int_{\mathbb{R}^N}(\nabla u \nabla \varphi-\mu\frac{u\varphi }{|x|^2}+\lambda u \varphi-f(u) \varphi) \mathrm{d} x=0
\end{align} implies that ![]() $(u,\lambda)$ satisfies
$(u,\lambda)$ satisfies
 \begin{align}
-\Delta u-\frac{\mu}{|x|^2}u+\lambda u=f(u).
\end{align}
\begin{align}
-\Delta u-\frac{\mu}{|x|^2}u+\lambda u=f(u).
\end{align}Thus, one has
 \begin{align}
\int_{\mathbb{R}^N}\left(|\nabla u|^2-\frac{\mu}{|x|^2}u^2+\lambda|u|^2-f(u) u\right) \mathrm{d} x=0,
\end{align}
\begin{align}
\int_{\mathbb{R}^N}\left(|\nabla u|^2-\frac{\mu}{|x|^2}u^2+\lambda|u|^2-f(u) u\right) \mathrm{d} x=0,
\end{align}and
 \begin{align}
\frac{N-2}{2 N} \int_{\mathbb{R}^N}|\nabla u|^2 -\frac{\mu}{|x|^2}u^2\mathrm{d} x+\frac{\lambda}{2} \int_{\mathbb{R}^N}|u|^2 \mathrm{d} x-\int_{\mathbb{R}^N} F(u) \mathrm{d} x=0 .
\end{align}
\begin{align}
\frac{N-2}{2 N} \int_{\mathbb{R}^N}|\nabla u|^2 -\frac{\mu}{|x|^2}u^2\mathrm{d} x+\frac{\lambda}{2} \int_{\mathbb{R}^N}|u|^2 \mathrm{d} x-\int_{\mathbb{R}^N} F(u) \mathrm{d} x=0 .
\end{align}Combined with (2.21) and (2.22), we can infer that
 \begin{equation*}
\int_{\mathbb{R}^N}|\nabla u|^2 -\frac{\mu}{|x|^2}u^2\mathrm{d} x-\frac{N}{2} \int_{\mathbb{R}^N}(f(u) u-2 F(u)) \mathrm{d} x=0 .
\end{equation*}
\begin{equation*}
\int_{\mathbb{R}^N}|\nabla u|^2 -\frac{\mu}{|x|^2}u^2\mathrm{d} x-\frac{N}{2} \int_{\mathbb{R}^N}(f(u) u-2 F(u)) \mathrm{d} x=0 .
\end{equation*} i.e. ![]() $P_\mu(u)=0$.
$P_\mu(u)=0$.
Defining ![]() $v_n:=u_n-u \rightharpoonup 0$ in
$v_n:=u_n-u \rightharpoonup 0$ in ![]() $H^1\left(\mathbb{R}^N\right)$, we can utilize the Brézis–Lieb lemma [Reference Brézis and Lieb13] to state that
$H^1\left(\mathbb{R}^N\right)$, we can utilize the Brézis–Lieb lemma [Reference Brézis and Lieb13] to state that
We claim that ![]() $v_n\to 0$ in
$v_n\to 0$ in ![]() $\mathcal{D}^{1,2}\left(\mathbb{R}^N\right)$. Let us proceed by assuming, for the sake of contradiction, that
$\mathcal{D}^{1,2}\left(\mathbb{R}^N\right)$. Let us proceed by assuming, for the sake of contradiction, that
 \begin{align*}
\lim_{n\rightarrow \infty} \int_{\mathbb{R}^N}\left|\nabla v_n\right|^2-\mu\frac{v_n^2}{|x|^2} \mathrm{d} x \gt 0.
\end{align*}
\begin{align*}
\lim_{n\rightarrow \infty} \int_{\mathbb{R}^N}\left|\nabla v_n\right|^2-\mu\frac{v_n^2}{|x|^2} \mathrm{d} x \gt 0.
\end{align*} Since ![]() $P_\mu(u)=0$, we have
$P_\mu(u)=0$, we have ![]() $P_\mu(v_n)= o_n(1)$. This implies
$P_\mu(v_n)= o_n(1)$. This implies
 \begin{align*}
\int_{\mathbb{R}^N}\left|\nabla v_n\right|^2-\mu\frac{v_n^2}{|x|^2} \mathrm{d} x+o_n(1) &=
\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F}(v_n)\mathrm{d} x\\
&= 2^* \eta \int_{\mathbb{R}^N}\left|v_n\right|^{2^*} \mathrm{d} x
+\int_{\mathbb{R}^N}\frac{N}{2}\widetilde{F}(v_n)-2^*\eta|v_n|^{2^*}\mathrm{d} x \\
&\leq 2^* \eta S_\mu^{\frac{N}{2-N}}\left(\int_{\mathbb{R}^N}\left|\nabla v_n\right|^2 -\mu\frac{v_n^2}{|x|^2} \mathrm{d} x
\right)^{\frac{N}{N-2}}+o_n(1).
\end{align*}
\begin{align*}
\int_{\mathbb{R}^N}\left|\nabla v_n\right|^2-\mu\frac{v_n^2}{|x|^2} \mathrm{d} x+o_n(1) &=
\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F}(v_n)\mathrm{d} x\\
&= 2^* \eta \int_{\mathbb{R}^N}\left|v_n\right|^{2^*} \mathrm{d} x
+\int_{\mathbb{R}^N}\frac{N}{2}\widetilde{F}(v_n)-2^*\eta|v_n|^{2^*}\mathrm{d} x \\
&\leq 2^* \eta S_\mu^{\frac{N}{2-N}}\left(\int_{\mathbb{R}^N}\left|\nabla v_n\right|^2 -\mu\frac{v_n^2}{|x|^2} \mathrm{d} x
\right)^{\frac{N}{N-2}}+o_n(1).
\end{align*}Similarly, we can deduce
 \begin{align*}
\lim \limits_{n\rightarrow \infty} \mathcal{I}_\mu(v_n)\geq \frac{1}{N}\left(2^* \eta\right)^{\frac{2-N}{2}}S_\mu^{\frac{N}{2}}.
\end{align*}
\begin{align*}
\lim \limits_{n\rightarrow \infty} \mathcal{I}_\mu(v_n)\geq \frac{1}{N}\left(2^* \eta\right)^{\frac{2-N}{2}}S_\mu^{\frac{N}{2}}.
\end{align*}Furthermore,
 \begin{align*}
\mathcal{I}_\mu(u)&= \mathcal{I}_\mu(u)-\frac{1}{2}P_\mu(u)= \int_{\mathbb{R}^N} \frac{N}{4}\widetilde{F}(u)-F(u)\mathrm{d} x \gt 0.
\end{align*}
\begin{align*}
\mathcal{I}_\mu(u)&= \mathcal{I}_\mu(u)-\frac{1}{2}P_\mu(u)= \int_{\mathbb{R}^N} \frac{N}{4}\widetilde{F}(u)-F(u)\mathrm{d} x \gt 0.
\end{align*}As a consequence, we arrive at
 \begin{equation*}
\begin{aligned}
\sigma_{\mu}(a)&= \mathcal{I}_\mu\left(v_n\right) + \mathcal{I}_\mu\left(u\right)+o_n(1)\geq \frac{1}{N}\left(2^* \eta\right)^{\frac{2-N}{2}}S_\mu^{\frac{N}{2}},
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
\sigma_{\mu}(a)&= \mathcal{I}_\mu\left(v_n\right) + \mathcal{I}_\mu\left(u\right)+o_n(1)\geq \frac{1}{N}\left(2^* \eta\right)^{\frac{2-N}{2}}S_\mu^{\frac{N}{2}},
\end{aligned}
\end{equation*} which contradicts lemma 2.11, so that ![]() $u_n\to u$ in
$u_n\to u$ in ![]() $\mathcal{D}^{1,2}(\mathbb{R}^N)$. Moreover, we have
$\mathcal{D}^{1,2}(\mathbb{R}^N)$. Moreover, we have
 \begin{align}
\lim_{n\rightarrow \infty} \int_{\mathbb{R}^N} |u_n|^{2^*} \mathrm{d} x=\int_{\mathbb{R}^N} |u|^{2^*} \mathrm{d} x,
\end{align}
\begin{align}
\lim_{n\rightarrow \infty} \int_{\mathbb{R}^N} |u_n|^{2^*} \mathrm{d} x=\int_{\mathbb{R}^N} |u|^{2^*} \mathrm{d} x,
\end{align}which leads to
 \begin{equation}
\begin{aligned}
\int_{\mathbb{R}^N} F\left(u_n\right) \mathrm{d} x \rightarrow \int_{\mathbb{R}^N} F(u) \mathrm{d} x, \quad
\int_{\mathbb{R}^N} f\left( u_n\right) u_n \mathrm{d} x \rightarrow \int_{\mathbb{R}^N} f(u) u \mathrm{d} x.
\end{aligned}
\end{equation}
\begin{equation}
\begin{aligned}
\int_{\mathbb{R}^N} F\left(u_n\right) \mathrm{d} x \rightarrow \int_{\mathbb{R}^N} F(u) \mathrm{d} x, \quad
\int_{\mathbb{R}^N} f\left( u_n\right) u_n \mathrm{d} x \rightarrow \int_{\mathbb{R}^N} f(u) u \mathrm{d} x.
\end{aligned}
\end{equation}Furthermore, by (2.20),
 \begin{align*}
-\Delta u -\mu\frac{u}{|x|^2}+\lambda u =f(u).
\end{align*}
\begin{align*}
-\Delta u -\mu\frac{u}{|x|^2}+\lambda u =f(u).
\end{align*} Since (2.21), (2.22), and ![]() $(F4)$, one obtains
$(F4)$, one obtains
 \begin{align*}
\lambda&= \frac{1}{a}\left( \int_{\mathbb{R}^N} f(u) u\mathrm{d} x
-\int_{\mathbb{R}^N}|\nabla u|^2 -\mu\frac{u^2}{|x|^2} \mathrm{d} x\right) \\
&= \frac{1}{a}\left\{\int_{\mathbb{R}^N}\frac{2-N}{2}f(u)u+NF(u) \mathrm{d} x\right\} \gt 0.
\end{align*}
\begin{align*}
\lambda&= \frac{1}{a}\left( \int_{\mathbb{R}^N} f(u) u\mathrm{d} x
-\int_{\mathbb{R}^N}|\nabla u|^2 -\mu\frac{u^2}{|x|^2} \mathrm{d} x\right) \\
&= \frac{1}{a}\left\{\int_{\mathbb{R}^N}\frac{2-N}{2}f(u)u+NF(u) \mathrm{d} x\right\} \gt 0.
\end{align*} Thus, λ > 0 and ![]() $u \in S_a$ by (2.18) and (2.19). According to lemmas 2.6 and 2.8,
$u \in S_a$ by (2.18) and (2.19). According to lemmas 2.6 and 2.8, ![]() $(u, \lambda) \in H_r^1\left(\mathbb{R}^N\right) \times \mathbb{R}^{+}$ is the normalized ground state solution of (1.6). We can further establish that u > 0 through the strong maximum principle. This concludes the proof.
$(u, \lambda) \in H_r^1\left(\mathbb{R}^N\right) \times \mathbb{R}^{+}$ is the normalized ground state solution of (1.6). We can further establish that u > 0 through the strong maximum principle. This concludes the proof.
Here, we provide the proof of proposition 1.2, which has been previously established in [Reference Li, Li and Tang34]. However, for completeness, we will only prove (i) and (ii). It is worth noting that the proof of (iii) has already been established in prior works [Reference Chou and Chu17, Reference Han24, Reference Li, Li and Tang34].
Proof of proposition 1.2
Since  $\frac{1}{|x|^2}\in C^{2}\left(\mathbb{R}^{N}\backslash B_{r_0}(0)\right)$ for any small
$\frac{1}{|x|^2}\in C^{2}\left(\mathbb{R}^{N}\backslash B_{r_0}(0)\right)$ for any small ![]() $r_0 \gt 0$. Then by using a standard elliptic regularity argument, we establish that
$r_0 \gt 0$. Then by using a standard elliptic regularity argument, we establish that ![]() $u \in C^2\left(\mathbb{R}^N \backslash\{0\} \right)$. We now turn our attention to proving the exponential decay of the solution. Since
$u \in C^2\left(\mathbb{R}^N \backslash\{0\} \right)$. We now turn our attention to proving the exponential decay of the solution. Since ![]() $u \in C^2\left(\mathbb{R}^N \backslash\{0\} \right)$ and
$u \in C^2\left(\mathbb{R}^N \backslash\{0\} \right)$ and ![]() $u\in S_{r,a}$, then
$u\in S_{r,a}$, then ![]() $u(x)\rightarrow 0$ as
$u(x)\rightarrow 0$ as ![]() $|x| \rightarrow+\infty$.
$|x| \rightarrow+\infty$.
Consequently, there exists R > 0 such that
 \begin{align}
- \Delta u(x)= \frac{\mu}{|x|^2} u(x)+f(u(x))-\lambda u(x) \leq -\frac{\lambda}{2} u(x) \quad \text {for all }|x| \geq R.
\end{align}
\begin{align}
- \Delta u(x)= \frac{\mu}{|x|^2} u(x)+f(u(x))-\lambda u(x) \leq -\frac{\lambda}{2} u(x) \quad \text {for all }|x| \geq R.
\end{align} Define  $\phi(x)=M \exp \left(-\sqrt{\frac{\lambda}{2}}|x|\right)$, where M is chosen to satisfy
$\phi(x)=M \exp \left(-\sqrt{\frac{\lambda}{2}}|x|\right)$, where M is chosen to satisfy
 \begin{equation*}
M \exp \left(-\sqrt{\frac{\lambda}{2}} R\right) \geq u(x) \,\text {for all }|x|=R.
\end{equation*}
\begin{equation*}
M \exp \left(-\sqrt{\frac{\lambda}{2}} R\right) \geq u(x) \,\text {for all }|x|=R.
\end{equation*}By direct calculation, it follows
 \begin{align*}
\Delta \phi=\left(\frac{\lambda}{2}-\frac{N-1}{r}\sqrt{\frac{\lambda}{2}} \right)\phi, \text {~for all~ } x \neq 0, \text{~where~} r=|x|.
\end{align*}
\begin{align*}
\Delta \phi=\left(\frac{\lambda}{2}-\frac{N-1}{r}\sqrt{\frac{\lambda}{2}} \right)\phi, \text {~for all~ } x \neq 0, \text{~where~} r=|x|.
\end{align*}This leads to the immediate conclusion
 \begin{align}
\Delta \phi \leq \frac{\lambda}{2} \phi \quad \text {for all } x \neq 0 .
\end{align}
\begin{align}
\Delta \phi \leq \frac{\lambda}{2} \phi \quad \text {for all } x \neq 0 .
\end{align} Combining (2.25) with (2.26), it becomes evident that the function ![]() $\varphi=\phi-u$ fulfils
$\varphi=\phi-u$ fulfils
 \begin{equation*}
\begin{cases}
-\Delta \varphi+\frac{\lambda}{2} \varphi \geq 0 & \text {in~ } |x| \geq R, \\
\varphi(x) \geq 0 & \text {in ~}|x|=R, \\
\lim \limits_{|x| \rightarrow \infty} \varphi(x)=0.
\end{cases}
\end{equation*}
\begin{equation*}
\begin{cases}
-\Delta \varphi+\frac{\lambda}{2} \varphi \geq 0 & \text {in~ } |x| \geq R, \\
\varphi(x) \geq 0 & \text {in ~}|x|=R, \\
\lim \limits_{|x| \rightarrow \infty} \varphi(x)=0.
\end{cases}
\end{equation*} In accordance with the maximum principle, it follows that ![]() $\varphi(x) \geq 0$ holds true for all
$\varphi(x) \geq 0$ holds true for all ![]() $|x| \geq R$. Consequently,
$|x| \geq R$. Consequently,
 \begin{align}
u(x) \leq M \exp \left(-\sqrt{\frac{\lambda}{2}}|x|\right), ~|x| \geq R.
\end{align}
\begin{align}
u(x) \leq M \exp \left(-\sqrt{\frac{\lambda}{2}}|x|\right), ~|x| \geq R.
\end{align} Further, based on ![]() $\left(F1\right)$–
$\left(F1\right)$–![]() $(F2)$ in conjunction with the exponential decay of u, it is evident that for sufficiently large
$(F2)$ in conjunction with the exponential decay of u, it is evident that for sufficiently large ![]() $|x|$,
$|x|$,
where ![]() $m_2 \geq m_1 \gt 0$. As u satisfies Eq. (1.6),
$m_2 \geq m_1 \gt 0$. As u satisfies Eq. (1.6),
 \begin{align}
-u_{r r}-\frac{N-1}{r} u_r-\frac{\mu}{r^2} u=f(u)-\lambda u, \quad r \in(r_0,+\infty),~ r_0 \gt 0,
\end{align}
\begin{align}
-u_{r r}-\frac{N-1}{r} u_r-\frac{\mu}{r^2} u=f(u)-\lambda u, \quad r \in(r_0,+\infty),~ r_0 \gt 0,
\end{align} with  $u_r=\frac{\partial u}{\partial r}, u_{r r}=\frac{\partial^2 u}{\partial r^2}, r=|x|$.
$u_r=\frac{\partial u}{\partial r}, u_{r r}=\frac{\partial^2 u}{\partial r^2}, r=|x|$.
It is a known fact that the equation
 \begin{align}
-\left(r^{N-1} u_r\right)_r=\mu r^{N-3} u+r^{N-1} f(u)-\lambda r^{N-1}u , \quad r \in(r_0,+\infty),~ r_0 \gt 0,
\end{align}
\begin{align}
-\left(r^{N-1} u_r\right)_r=\mu r^{N-3} u+r^{N-1} f(u)-\lambda r^{N-1}u , \quad r \in(r_0,+\infty),~ r_0 \gt 0,
\end{align} can be integrated over the interval (r, R), using (2.27), and then letting ![]() $r, R \rightarrow +\infty$. This integration demonstrates that
$r, R \rightarrow +\infty$. This integration demonstrates that ![]() $r^{N-1} u_r$ possesses a limit as
$r^{N-1} u_r$ possesses a limit as ![]() $r \rightarrow \infty$, which, according to (2.27), must be zero. Furthermore, integrating (2.29) over
$r \rightarrow \infty$, which, according to (2.27), must be zero. Furthermore, integrating (2.29) over ![]() $(r,+\infty)$ implies exponential decay of ur (also referenced in [Reference Berestycki and Lions10]). Finally, the exponential decay of urr, and consequently of
$(r,+\infty)$ implies exponential decay of ur (also referenced in [Reference Berestycki and Lions10]). Finally, the exponential decay of urr, and consequently of ![]() $\left|D^\alpha u(x)\right|$ for
$\left|D^\alpha u(x)\right|$ for ![]() $|\alpha| \leq 2$, directly follows from (2.28). This concludes the proof.
$|\alpha| \leq 2$, directly follows from (2.28). This concludes the proof.
3. Proof of theorem 1.3
In this section, we delve into the asymptotic behaviour of the solution to Eq. (1.6) as ![]() $\mu\rightarrow 0^+$.
$\mu\rightarrow 0^+$.
Lemma 3.1. Assume that ![]() $N\geq 3$, a > 0,
$N\geq 3$, a > 0,  $1-\frac{\mu}{\bar{\mu}} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and
$1-\frac{\mu}{\bar{\mu}} \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$, and ![]() $(F 1)$–
$(F 1)$–![]() $(F 5)$ hold. Then, for any sequence
$(F 5)$ hold. Then, for any sequence ![]() $ \mu_n\in (0, \bar{\mu})$ with
$ \mu_n\in (0, \bar{\mu})$ with ![]() $\mu_n \rightarrow 0^{+}$ as
$\mu_n \rightarrow 0^{+}$ as ![]() $n \rightarrow \infty$, we have
$n \rightarrow \infty$, we have  $\lim \limits_{n \rightarrow \infty} m_{\mu_n}(a)=m_{\infty}(a)$.
$\lim \limits_{n \rightarrow \infty} m_{\mu_n}(a)=m_{\infty}(a)$.
Proof. For any ![]() $0 \lt \mu_n \lt \bar{\mu}$,
$0 \lt \mu_n \lt \bar{\mu}$,
 \begin{align*}
\mathcal{I}_{\infty}(u)= \mathcal{I}_{\mu_n}(u)+\mu_n \int_{\mathbb{R}^N} \frac{u^2}{|x|^2} \mathrm{d} x,
\end{align*}
\begin{align*}
\mathcal{I}_{\infty}(u)= \mathcal{I}_{\mu_n}(u)+\mu_n \int_{\mathbb{R}^N} \frac{u^2}{|x|^2} \mathrm{d} x,
\end{align*}and consequently, by lemma 2.3
 \begin{align*}
m_{\infty}(a)=\inf _{u \in S_a} \max _{t \gt 0} \mathcal{I}_{\infty}(t \star u) \geq \inf _{u \in S_a} \max _{t \gt 0} \mathcal{I}_{\mu_n}(t \star u)=m_{\mu_n}(a).
\end{align*}
\begin{align*}
m_{\infty}(a)=\inf _{u \in S_a} \max _{t \gt 0} \mathcal{I}_{\infty}(t \star u) \geq \inf _{u \in S_a} \max _{t \gt 0} \mathcal{I}_{\mu_n}(t \star u)=m_{\mu_n}(a).
\end{align*}We now proceed to assert that
 \begin{equation*}
m_\infty(a)\leq \lim _{n \rightarrow \infty} m_{\mu_n}(a).
\end{equation*}
\begin{equation*}
m_\infty(a)\leq \lim _{n \rightarrow \infty} m_{\mu_n}(a).
\end{equation*} For each ![]() $n \geq 1$, let
$n \geq 1$, let ![]() $u_n \in \mathcal{M}_{\mu_n}(a)$ be such that
$u_n \in \mathcal{M}_{\mu_n}(a)$ be such that
 \begin{equation*} \mathcal{I}_{\mu_n}\left(u_n\right)=m_{\mu_n}(a) \lt m_{\infty}(a)+\frac{1}{n}.\end{equation*}
\begin{equation*} \mathcal{I}_{\mu_n}\left(u_n\right)=m_{\mu_n}(a) \lt m_{\infty}(a)+\frac{1}{n}.\end{equation*} Consequently,  $\left|\nabla u_n\right|_{2}^2 \leq C$ for all
$\left|\nabla u_n\right|_{2}^2 \leq C$ for all ![]() $n \geq 1$, ensuring that un is bounded in
$n \geq 1$, ensuring that un is bounded in ![]() $H^1\left(\mathbb{R}^N\right)$. Let tn be determined according to lemma 2.3 such that
$H^1\left(\mathbb{R}^N\right)$. Let tn be determined according to lemma 2.3 such that ![]() $
t_n \star u_n \in \mathcal{M}_\infty(a).
$ Moreover,
$
t_n \star u_n \in \mathcal{M}_\infty(a).
$ Moreover,
 \begin{align*}
P_{\mu_n}\left(u_n\right)=\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2-\mu_n \frac{u_n^2}{|x|^2} \mathrm{d} x
-\frac{N}{2} \int_{\mathbb{R}^N}\widetilde{F}(u_n) \mathrm{d} x=0,
\end{align*}
\begin{align*}
P_{\mu_n}\left(u_n\right)=\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2-\mu_n \frac{u_n^2}{|x|^2} \mathrm{d} x
-\frac{N}{2} \int_{\mathbb{R}^N}\widetilde{F}(u_n) \mathrm{d} x=0,
\end{align*}and
 \begin{align*}
P_{\infty}\left(t_n \star u_n\right)=t_n^2 \int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 \mathrm{d} x
-\frac{N}{2} t_n^{-N} \int_{\mathbb{R}^N} \widetilde{F}\left(t_n^{\frac{N}{2}} u_n\right) \mathrm{d} x=0 .
\end{align*}
\begin{align*}
P_{\infty}\left(t_n \star u_n\right)=t_n^2 \int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 \mathrm{d} x
-\frac{N}{2} t_n^{-N} \int_{\mathbb{R}^N} \widetilde{F}\left(t_n^{\frac{N}{2}} u_n\right) \mathrm{d} x=0 .
\end{align*} As ![]() $n \rightarrow \infty$, we establish
$n \rightarrow \infty$, we establish
 \begin{equation*}
\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(t_n^{\frac{N}{2}} u_n\right)}{\left|t_n^{\frac{N}{2}} u_n\right|^{2+\frac{4}{N}}}\left|u_n\right|^{2+\frac{4}{N}} \mathrm{d} x
=\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(u_n\right)}{\left|u_n\right|^{2+\frac{4}{N}}}\left|u_n\right|^{2+\frac{4}{N}} \mathrm{d} x+o_n(1).
\end{equation*}
\begin{equation*}
\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(t_n^{\frac{N}{2}} u_n\right)}{\left|t_n^{\frac{N}{2}} u_n\right|^{2+\frac{4}{N}}}\left|u_n\right|^{2+\frac{4}{N}} \mathrm{d} x
=\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(u_n\right)}{\left|u_n\right|^{2+\frac{4}{N}}}\left|u_n\right|^{2+\frac{4}{N}} \mathrm{d} x+o_n(1).
\end{equation*} Furthermore, based on ![]() $(F3)$, it follows that
$(F3)$, it follows that ![]() $t_n \rightarrow 1$ as
$t_n \rightarrow 1$ as ![]() $n \rightarrow \infty$. By [Reference Bartsch and Soave7, lemma 3.5], we have
$n \rightarrow \infty$. By [Reference Bartsch and Soave7, lemma 3.5], we have ![]() $\|t_n \star u_n-u_n\| \rightarrow 0$, and consequently,
$\|t_n \star u_n-u_n\| \rightarrow 0$, and consequently,
This entails
 \begin{equation*}
\begin{aligned}
m_\infty(a) & \leq \mathcal{I}_\infty\left(t_n \star u_n\right)=\mathcal{I}_\infty\left(u_n\right)+o_n(1) \\
& = \mathcal{I}_{\mu_n}\left(u_n\right)+\mu_n \int_{\mathbb{R}^N} \frac{u_n^2}{|x|^2} \mathrm{d} x+o_n(1)
\leq m_{\mu_n}(a)+o_n(1).
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
m_\infty(a) & \leq \mathcal{I}_\infty\left(t_n \star u_n\right)=\mathcal{I}_\infty\left(u_n\right)+o_n(1) \\
& = \mathcal{I}_{\mu_n}\left(u_n\right)+\mu_n \int_{\mathbb{R}^N} \frac{u_n^2}{|x|^2} \mathrm{d} x+o_n(1)
\leq m_{\mu_n}(a)+o_n(1).
\end{aligned}
\end{equation*} Hence, we conclude that  $ m_\infty(a)\leq \lim \limits_{n \rightarrow \infty} m_{\mu_n}(a)$. This concludes the proof.
$ m_\infty(a)\leq \lim \limits_{n \rightarrow \infty} m_{\mu_n}(a)$. This concludes the proof.
Proof of theorem 1.3
Assume that ![]() $(u_{n},\lambda_{n})$ is obtained in theorem 1.1 with
$(u_{n},\lambda_{n})$ is obtained in theorem 1.1 with ![]() $m_{\mu_n}(a)$, where
$m_{\mu_n}(a)$, where ![]() $\bar{\mu} \gt \mu_n$ and
$\bar{\mu} \gt \mu_n$ and ![]() $\mu_n \rightarrow 0^+$. In other words,
$\mu_n \rightarrow 0^+$. In other words, ![]() $(u_n,\lambda_n)$ satisfies
$(u_n,\lambda_n)$ satisfies
 \begin{align}
-\Delta u_n -\frac{\mu_n}{|x|^2} u_n +\lambda_n u_n=f(u_n).
\end{align}
\begin{align}
-\Delta u_n -\frac{\mu_n}{|x|^2} u_n +\lambda_n u_n=f(u_n).
\end{align} Consequently, ![]() $P_{\mu_n}(u_n)=0$.
$P_{\mu_n}(u_n)=0$.
Similarly to the proof of theorem 1.1, ![]() $\{u_n\}$ is bounded in
$\{u_n\}$ is bounded in ![]() $H_r^1\left(\mathbb{R}^N\right)$, so that there exists a nonnegative function
$H_r^1\left(\mathbb{R}^N\right)$, so that there exists a nonnegative function ![]() $u \in H_r^1\left(\mathbb{R}^N\right)$ such that
$u \in H_r^1\left(\mathbb{R}^N\right)$ such that ![]() $u_n \rightharpoonup u$ in
$u_n \rightharpoonup u$ in ![]() $H_r^1\left(\mathbb{R}^N\right)$,
$H_r^1\left(\mathbb{R}^N\right)$, ![]() $u_n \rightarrow u$ in
$u_n \rightarrow u$ in ![]() $L^p\left(\mathbb{R}^N\right)$ for
$L^p\left(\mathbb{R}^N\right)$ for ![]() $p \in\left(2,2^*\right)$, and
$p \in\left(2,2^*\right)$, and ![]() $u_n(x) \rightarrow u(x)$ almost everywhere in
$u_n(x) \rightarrow u(x)$ almost everywhere in ![]() $\mathbb{R}^N$. Consequently, by taking
$\mathbb{R}^N$. Consequently, by taking ![]() $n\to\infty$ in (3.1), we have
$n\to\infty$ in (3.1), we have
which shows that ![]() $P_\infty(u)=0$.
$P_\infty(u)=0$.
Claim 1: ![]() $u\not=0$. If not, u = 0, and
$u\not=0$. If not, u = 0, and ![]() $P_{\mu_n}(u_n)=0$ combined with (2.16) implies that
$P_{\mu_n}(u_n)=0$ combined with (2.16) implies that
 \begin{align}
\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 \mathrm{d} x & =
\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F}(u_n)\mathrm{d} x +
\mu_n \int_{\mathbb{R}^N}\frac{u_n^2}{|x|^2}\mathrm{d} x \nonumber \\
&= 2^* \eta \int_{\mathbb{R}^N}|u_n|^{2^*}\mathrm{d} x+
\int_{\mathbb{R}^N} \frac{N}{2}\widetilde{F}(u_n)-2^*
\eta |u_n|^{2^*}\mathrm{d} x + \mu_n\int_{\mathbb{R}^N}\frac{u_n^2}{|x|^2}\mathrm{d} x \nonumber\\
&= 2^* \eta \int_{\mathbb{R}^N}\left|u_n\right|^{2^*}\mathrm{d} x
+o_n(1) \nonumber\\
& \leq 2^* \eta S^{\frac{N}{2-N}}\left(\int_{\mathbb{R}^N}
\left|\nabla u_n\right|^2 \mathrm{d} x\right)^{\frac{N}{N-2}}+o_n(1).
\end{align}
\begin{align}
\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 \mathrm{d} x & =
\frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F}(u_n)\mathrm{d} x +
\mu_n \int_{\mathbb{R}^N}\frac{u_n^2}{|x|^2}\mathrm{d} x \nonumber \\
&= 2^* \eta \int_{\mathbb{R}^N}|u_n|^{2^*}\mathrm{d} x+
\int_{\mathbb{R}^N} \frac{N}{2}\widetilde{F}(u_n)-2^*
\eta |u_n|^{2^*}\mathrm{d} x + \mu_n\int_{\mathbb{R}^N}\frac{u_n^2}{|x|^2}\mathrm{d} x \nonumber\\
&= 2^* \eta \int_{\mathbb{R}^N}\left|u_n\right|^{2^*}\mathrm{d} x
+o_n(1) \nonumber\\
& \leq 2^* \eta S^{\frac{N}{2-N}}\left(\int_{\mathbb{R}^N}
\left|\nabla u_n\right|^2 \mathrm{d} x\right)^{\frac{N}{N-2}}+o_n(1).
\end{align}Combined with lemma 2.4, we deduce that
 \begin{equation*}
\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 \mathrm{d} x
\geq \left( 2^* \eta\right)^{\frac{2-N}{2}} S^{\frac{N}{2}}+o_n(1) .
\end{equation*}
\begin{equation*}
\int_{\mathbb{R}^N}\left|\nabla u_n\right|^2 \mathrm{d} x
\geq \left( 2^* \eta\right)^{\frac{2-N}{2}} S^{\frac{N}{2}}+o_n(1) .
\end{equation*} Since ![]() $\left\{u_n\right\}$ is bounded in
$\left\{u_n\right\}$ is bounded in ![]() $H^1(\mathbb{R}^N)$ and
$H^1(\mathbb{R}^N)$ and ![]() $\mu_n \rightarrow 0^{+}$, we have
$\mu_n \rightarrow 0^{+}$, we have
 \begin{align}
m_{\mu_n}(a)&= \mathcal{I}_{\mu_n}\left(u_n\right)-\frac{1}{2^*}P_{\mu_n}(u_n) \nonumber \\
& =\frac{1}{N} \int_{\mathbb{R}^N}\left|\nabla u_n\right|^2
-\mu_n \frac{u_n^2}{|x|^2}\mathrm{d} x+\frac{N-2}{4} \int_{\mathbb{R}^N}f(u_n)u_n-2^*F(u_n)
\mathrm{d} x \nonumber \\
&\geq \frac{1}{N}
\left( 2^* \eta \right)^{\frac{2-N}{2}} S^{\frac{N}{2}}+o_n(1).
\end{align}
\begin{align}
m_{\mu_n}(a)&= \mathcal{I}_{\mu_n}\left(u_n\right)-\frac{1}{2^*}P_{\mu_n}(u_n) \nonumber \\
& =\frac{1}{N} \int_{\mathbb{R}^N}\left|\nabla u_n\right|^2
-\mu_n \frac{u_n^2}{|x|^2}\mathrm{d} x+\frac{N-2}{4} \int_{\mathbb{R}^N}f(u_n)u_n-2^*F(u_n)
\mathrm{d} x \nonumber \\
&\geq \frac{1}{N}
\left( 2^* \eta \right)^{\frac{2-N}{2}} S^{\frac{N}{2}}+o_n(1).
\end{align}Thus,
 \begin{equation*}
\lim \limits_{n \rightarrow \infty} m_{\mu_n}(a)=m_{\infty}(a) \geq \frac{1}{N}\left(2^* \eta\right)^{\frac{2-N}{2}} S^{\frac{N}{2}},
\end{equation*}
\begin{equation*}
\lim \limits_{n \rightarrow \infty} m_{\mu_n}(a)=m_{\infty}(a) \geq \frac{1}{N}\left(2^* \eta\right)^{\frac{2-N}{2}} S^{\frac{N}{2}},
\end{equation*} which contradicts lemma 2.11, and then ![]() $u\not=0$. Based on Eq. (3.2), we have
$u\not=0$. Based on Eq. (3.2), we have
 \begin{align*}
\lambda |u|^2_2&=\int_{\mathbb{R}^N}f(u)u\mathrm{d} x-\int_{\mathbb{R}^N}|\nabla u|^2\mathrm{d} x
=\int_{\mathbb{R}^N}f(u)u\mathrm{d} x- \frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F}(u) \mathrm{d} x \gt 0,
\end{align*}
\begin{align*}
\lambda |u|^2_2&=\int_{\mathbb{R}^N}f(u)u\mathrm{d} x-\int_{\mathbb{R}^N}|\nabla u|^2\mathrm{d} x
=\int_{\mathbb{R}^N}f(u)u\mathrm{d} x- \frac{N}{2}\int_{\mathbb{R}^N}\widetilde{F}(u) \mathrm{d} x \gt 0,
\end{align*} which holds due to ![]() $(F4)$.
$(F4)$.
Claim 2: ![]() $u_n \rightarrow u$ in
$u_n \rightarrow u$ in ![]() $\mathcal{D}^{1,2}\left(\mathbb{R}^N\right)$. Let us proceed by contradiction and assume that
$\mathcal{D}^{1,2}\left(\mathbb{R}^N\right)$. Let us proceed by contradiction and assume that
 \begin{equation*}\nu:= \lim \limits_{n\rightarrow \infty}\int_{\mathbb{R}^N}\left|\nabla v_n\right|^2 \mathrm{d} x \gt 0,\end{equation*}
\begin{equation*}\nu:= \lim \limits_{n\rightarrow \infty}\int_{\mathbb{R}^N}\left|\nabla v_n\right|^2 \mathrm{d} x \gt 0,\end{equation*} where ![]() $v_n=u_n-u$. Since
$v_n=u_n-u$. Since ![]() $P_{\mu_n}(u_n)=0$, we can infer that
$P_{\mu_n}(u_n)=0$, we can infer that ![]() $P_{\mu_n}(v_n)= 0$. Similarly, one can see that
$P_{\mu_n}(v_n)= 0$. Similarly, one can see that
 \begin{align*}
\mathcal{I}_{\mu_n}(v_n)\geq \frac{1}{N}
\left( 2^* \eta \right)^{\frac{2-N}{2}} S^{\frac{N}{2}}+o_n(1),
\end{align*}
\begin{align*}
\mathcal{I}_{\mu_n}(v_n)\geq \frac{1}{N}
\left( 2^* \eta \right)^{\frac{2-N}{2}} S^{\frac{N}{2}}+o_n(1),
\end{align*}and by lemma 2.1,
 \begin{align*}
\mathcal{I}_{\infty}(u)=\mathcal{I}_{\infty}(u)-\frac{1}{2}P_{\infty}(u)= \int_{\mathbb{R}^N} \frac{N}{4}\widetilde{F}(u)-F(u)\mathrm{d} x \gt 0.
\end{align*}
\begin{align*}
\mathcal{I}_{\infty}(u)=\mathcal{I}_{\infty}(u)-\frac{1}{2}P_{\infty}(u)= \int_{\mathbb{R}^N} \frac{N}{4}\widetilde{F}(u)-F(u)\mathrm{d} x \gt 0.
\end{align*}Consequently, we arrive at
 \begin{align*}
m_{\mu_n}(a)&= \mathcal{I}_{\mu_n}(u_n)= \mathcal{I}_{\mu_n}(v_n)+ \mathcal{I}_{\infty}(u)+o_n(1) \gt \frac{1}{N}
\left( 2^* \eta \right)^{\frac{2-N}{2}} S^{\frac{N}{2}}+o_n(1) .
\end{align*}
\begin{align*}
m_{\mu_n}(a)&= \mathcal{I}_{\mu_n}(u_n)= \mathcal{I}_{\mu_n}(v_n)+ \mathcal{I}_{\infty}(u)+o_n(1) \gt \frac{1}{N}
\left( 2^* \eta \right)^{\frac{2-N}{2}} S^{\frac{N}{2}}+o_n(1) .
\end{align*}This leads to
 \begin{equation*}\lim \limits_{n \rightarrow \infty} m_{\mu_n}(a)=m_{\infty}(a)\geq
\frac{1}{N}\left(2^* \eta\right)^{\frac{2-N}{2}} S^{\frac{N}{2}},\end{equation*}
\begin{equation*}\lim \limits_{n \rightarrow \infty} m_{\mu_n}(a)=m_{\infty}(a)\geq
\frac{1}{N}\left(2^* \eta\right)^{\frac{2-N}{2}} S^{\frac{N}{2}},\end{equation*} which is a contradiction. Thus, we conclude that ![]() $u_n\rightarrow u$ in
$u_n\rightarrow u$ in ![]() $\mathcal{D}^{1,2}\left(\mathbb{R}^N\right)$.
$\mathcal{D}^{1,2}\left(\mathbb{R}^N\right)$.
Furthermore, we can establish that
 \begin{align*}
\lim_{n\rightarrow \infty} \int_{\mathbb{R}^N} |u_n|^{2^*} \mathrm{d} x=\int_{\mathbb{R}^N} |u|^{2^*} \mathrm{d} x,
\end{align*}
\begin{align*}
\lim_{n\rightarrow \infty} \int_{\mathbb{R}^N} |u_n|^{2^*} \mathrm{d} x=\int_{\mathbb{R}^N} |u|^{2^*} \mathrm{d} x,
\end{align*}and
 \begin{align*}
\int_{\mathbb{R}^N} F\left(u_n\right) \mathrm{d} x \rightarrow \int_{\mathbb{R}^N} F(u) \mathrm{d} x.
\end{align*}
\begin{align*}
\int_{\mathbb{R}^N} F\left(u_n\right) \mathrm{d} x \rightarrow \int_{\mathbb{R}^N} F(u) \mathrm{d} x.
\end{align*}Consequently,
 \begin{equation*}
\lim _{n \rightarrow \infty} \lambda_n\int_{\mathbb{R}^N} |u_n|^2\mathrm{d} x=\lambda
\int_{\mathbb{R}^N}u^2\mathrm{d} x,
\end{equation*}
\begin{equation*}
\lim _{n \rightarrow \infty} \lambda_n\int_{\mathbb{R}^N} |u_n|^2\mathrm{d} x=\lambda
\int_{\mathbb{R}^N}u^2\mathrm{d} x,
\end{equation*} and given that λ > 0, so that ![]() $u_n\to u$ in
$u_n\to u$ in ![]() $H_r^1(\mathbb{R}^N)$. Thus, by lemma 3.1,
$H_r^1(\mathbb{R}^N)$. Thus, by lemma 3.1, ![]() $(u, \lambda) \in H_r^1(\mathbb{R}^N) \times \mathbb{R}^{+}$ is a normalized ground state of Eq. (1.8). Moreover, u > 0 by the strong maximum principle.
$(u, \lambda) \in H_r^1(\mathbb{R}^N) \times \mathbb{R}^{+}$ is a normalized ground state of Eq. (1.8). Moreover, u > 0 by the strong maximum principle.
4. Proof of theorem 1.4
In this section, we focus on the existence of normalized solutions for Eq. (1.6) when µ < 0.
Lemma 4.1. Assume that ![]() $N \geq 3$,
$N \geq 3$, ![]() $0 \gt \mu$, and
$0 \gt \mu$, and ![]() $\left(F1\right)$–
$\left(F1\right)$–![]() $\left(F5\right)$ hold. Then,
$\left(F5\right)$ hold. Then, ![]() $m_\mu(a)=m_{\infty}(a)$. Additionally,
$m_\mu(a)=m_{\infty}(a)$. Additionally, ![]() $m_\mu(a)$ cannot be attained.
$m_\mu(a)$ cannot be attained.
Proof. When µ < 0, it becomes evident that ![]() $m_{\infty}(a) \leq m_\mu(a)$. According to theorem 1.3, Eq. (1.8) possesses a ground state solution
$m_{\infty}(a) \leq m_\mu(a)$. According to theorem 1.3, Eq. (1.8) possesses a ground state solution ![]() $v \in \mathcal{M}_{\infty}(a)$, achieving
$v \in \mathcal{M}_{\infty}(a)$, achieving ![]() $m_{\infty}(a)$, i.e.
$m_{\infty}(a)$, i.e. ![]() $\mathcal{I}_{\infty}(v)=m_{\infty}(a)$ and
$\mathcal{I}_{\infty}(v)=m_{\infty}(a)$ and ![]() $P_{\infty}(v)=0$. Moreover, due to the exponential decay of v, we have
$P_{\infty}(v)=0$. Moreover, due to the exponential decay of v, we have
 \begin{equation*}
v(x) \leq M \exp \left(-\sqrt{\frac{\lambda}{2}}|x|\right), |x| \gt R, ~\text{for some } R \gt 0.
\end{equation*}
\begin{equation*}
v(x) \leq M \exp \left(-\sqrt{\frac{\lambda}{2}}|x|\right), |x| \gt R, ~\text{for some } R \gt 0.
\end{equation*} Consequently, we can introduce ![]() $v_n(x) = v(x - y_ne_1)$, where
$v_n(x) = v(x - y_ne_1)$, where ![]() $e_1=(1,0,\ldots,0)$,
$e_1=(1,0,\ldots,0)$, ![]() $y_n\in \mathbb{R}^+$ and
$y_n\in \mathbb{R}^+$ and ![]() $y_n \rightarrow+\infty$ as
$y_n \rightarrow+\infty$ as ![]() $n \rightarrow \infty$. Furthermore, given any ϵ > 0, there exists
$n \rightarrow \infty$. Furthermore, given any ϵ > 0, there exists ![]() $R_{\epsilon} \gt 0$ such that,
$R_{\epsilon} \gt 0$ such that,
 \begin{equation*}
\frac{1}{|x|^2} \leq \epsilon,~ \text{for all}~ |x| \geq R_{\epsilon}.
\end{equation*}
\begin{equation*}
\frac{1}{|x|^2} \leq \epsilon,~ \text{for all}~ |x| \geq R_{\epsilon}.
\end{equation*} Since ![]() $y_n \rightarrow+\infty$ as
$y_n \rightarrow+\infty$ as ![]() $n \rightarrow \infty$, there exists
$n \rightarrow \infty$, there exists ![]() $R_{\epsilon} \gt 0$, such that as
$R_{\epsilon} \gt 0$, such that as ![]() $n\rightarrow \infty$,
$n\rightarrow \infty$,
 \begin{equation*}
v_n(x) \leq M \exp \left(-\sqrt{\frac{\lambda}{2}}\left|x-y_n\right|\right)
\leq \frac{C}{\left|x-y_n\right|},~ x\in B_{R_{\epsilon}}(0).
\end{equation*}
\begin{equation*}
v_n(x) \leq M \exp \left(-\sqrt{\frac{\lambda}{2}}\left|x-y_n\right|\right)
\leq \frac{C}{\left|x-y_n\right|},~ x\in B_{R_{\epsilon}}(0).
\end{equation*}This results in
 \begin{equation*}
\begin{aligned}
\int_{\mathbb{R}^N} \frac{v_n^2}{|x|^2} \mathrm{d} x& =\int_{B_{R_{\epsilon}}(0)} \frac{v_n^2}{|x|^2} \mathrm{d} x+\int_{\mathbb{R}^N \backslash B_{R_{\epsilon}}(0)} \frac{v_n^2 }{|x|^2} \mathrm{d} x\\
& \leq \frac{C}{\left|y_n-R_{\epsilon}\right|^2} \int_{B_{R_{\epsilon}}(0)} \frac{1}{|x|^2} \mathrm{d} x+\epsilon \int_{\mathbb{R}^N \backslash B_{R_{\epsilon}}(0)} v_n^2 \mathrm{d} x\\
& \leq \frac{C_1}{\left|y_n-R_{\epsilon}\right|^2}+\epsilon a \rightarrow 0, ~\text{as}~ n\rightarrow \infty.
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
\int_{\mathbb{R}^N} \frac{v_n^2}{|x|^2} \mathrm{d} x& =\int_{B_{R_{\epsilon}}(0)} \frac{v_n^2}{|x|^2} \mathrm{d} x+\int_{\mathbb{R}^N \backslash B_{R_{\epsilon}}(0)} \frac{v_n^2 }{|x|^2} \mathrm{d} x\\
& \leq \frac{C}{\left|y_n-R_{\epsilon}\right|^2} \int_{B_{R_{\epsilon}}(0)} \frac{1}{|x|^2} \mathrm{d} x+\epsilon \int_{\mathbb{R}^N \backslash B_{R_{\epsilon}}(0)} v_n^2 \mathrm{d} x\\
& \leq \frac{C_1}{\left|y_n-R_{\epsilon}\right|^2}+\epsilon a \rightarrow 0, ~\text{as}~ n\rightarrow \infty.
\end{aligned}
\end{equation*} Since ![]() $v_n\in \mathcal{M}_{\infty}(a)$,
$v_n\in \mathcal{M}_{\infty}(a)$,
 \begin{align*}
P_{\infty}\left(v_n\right)=\int_{\mathbb{R}^N}\left|\nabla v_n\right|^2 \mathrm{d} x-\frac{N}{2} \int_{\mathbb{R}^N}\left[f\left(v_n\right) v_n-2F\left(v_n\right)\right] \mathrm{d} x=0,
\end{align*}
\begin{align*}
P_{\infty}\left(v_n\right)=\int_{\mathbb{R}^N}\left|\nabla v_n\right|^2 \mathrm{d} x-\frac{N}{2} \int_{\mathbb{R}^N}\left[f\left(v_n\right) v_n-2F\left(v_n\right)\right] \mathrm{d} x=0,
\end{align*} as deduced from lemma 2.3, there exists a unique ![]() $t_n \gt 0$ satisfying
$t_n \gt 0$ satisfying
 \begin{align*}
P\left(t_n \star v_n\right)=t_n^2 \int_{\mathbb{R}^N}\left(\left|\nabla v_n\right|^2-\mu\frac{v_n^2}{|x|^2} \right) \mathrm{d} x-\frac{N}{2} t_n^{-N} \int_{\mathbb{R}^N} \widetilde{F}\left(t_n^{\frac{N}{2}} v_n\right) \mathrm{d} x=0 .
\end{align*}
\begin{align*}
P\left(t_n \star v_n\right)=t_n^2 \int_{\mathbb{R}^N}\left(\left|\nabla v_n\right|^2-\mu\frac{v_n^2}{|x|^2} \right) \mathrm{d} x-\frac{N}{2} t_n^{-N} \int_{\mathbb{R}^N} \widetilde{F}\left(t_n^{\frac{N}{2}} v_n\right) \mathrm{d} x=0 .
\end{align*} Therefore, as ![]() $y_n \rightarrow \infty$, we establish
$y_n \rightarrow \infty$, we establish
 \begin{equation*}
\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(t_n^{\frac{N}{2}} v_n\right)}{\left|t_n^{\frac{N}{2}} v_n\right|^{2+\frac{4}{N}}}\left|v_n\right|^{2+\frac{4}{N}} \mathrm{d} x=\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(v_n\right)}{\left|v_n\right|^{2+\frac{4}{N}}}\left|v_n\right|^{2+\frac{4}{N}} \mathrm{d} x+o_n(1).
\end{equation*}
\begin{equation*}
\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(t_n^{\frac{N}{2}} v_n\right)}{\left|t_n^{\frac{N}{2}} v_n\right|^{2+\frac{4}{N}}}\left|v_n\right|^{2+\frac{4}{N}} \mathrm{d} x=\int_{\mathbb{R}^N} \frac{\widetilde{F}\left(v_n\right)}{\left|v_n\right|^{2+\frac{4}{N}}}\left|v_n\right|^{2+\frac{4}{N}} \mathrm{d} x+o_n(1).
\end{equation*} Furthermore, based on ![]() $(F3)$, it follows that
$(F3)$, it follows that ![]() $t_n \rightarrow 1$ as
$t_n \rightarrow 1$ as ![]() $n \rightarrow \infty$. We now proceed to demonstrate that
$n \rightarrow \infty$. We now proceed to demonstrate that ![]() $m_\mu(a) \leq m_{\infty}(a)$. As
$m_\mu(a) \leq m_{\infty}(a)$. As ![]() $\left\{t_n \star v_n\right\} \subset \mathcal{M}_\mu(a)$ and
$\left\{t_n \star v_n\right\} \subset \mathcal{M}_\mu(a)$ and ![]() $t_n \rightarrow 1$ as
$t_n \rightarrow 1$ as ![]() $n \rightarrow \infty$, it follows that
$n \rightarrow \infty$, it follows that
 \begin{equation*}
\begin{aligned}
m_\mu(a) & \leq \mathcal{I}_\mu\left(t_n \star v_n\right)= \mathcal{I}_\mu\left(t_n \star v_n\right)-\frac{1}{2^*} P_\mu\left(t_n \star v_n\right) \\
& = \frac{t_n^2}{N}\int_{\mathbb{R}^N} |\nabla v_n|^2 -\frac{\mu}{|x|^2} v_n^2\mathrm{d} x+
\int_{\mathbb{R}^N} \frac{N-2}{4} f\left(t_n \star v_n\right) t_n \star v_n \\ &\quad -\frac{N}{2} F\left(t_n \star v_n\right) \mathrm{d} x\\
&= \frac{1}{N}\int_{\mathbb{R}^N} |\nabla v_n|^2\mathrm{d} x+
\int_{\mathbb{R}^N} \frac{N-2}{4} f\left(v_n\right) v_n -\frac{N}{2} F\left(v_n\right) \mathrm{d} x+o_n(1) \\
& =\mathcal{I}_{\infty}\left(v_n\right)-\frac{1}{2^*} P_{\infty}\left(v_n\right) +o_n(1) \\
& =\mathcal{I}_{\infty}\left(v_n\right)+o_n(1)=\mathcal{I}_{\infty}\left(v\right)+o_n(1)=m_{\infty}(a)+o_n(1).
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
m_\mu(a) & \leq \mathcal{I}_\mu\left(t_n \star v_n\right)= \mathcal{I}_\mu\left(t_n \star v_n\right)-\frac{1}{2^*} P_\mu\left(t_n \star v_n\right) \\
& = \frac{t_n^2}{N}\int_{\mathbb{R}^N} |\nabla v_n|^2 -\frac{\mu}{|x|^2} v_n^2\mathrm{d} x+
\int_{\mathbb{R}^N} \frac{N-2}{4} f\left(t_n \star v_n\right) t_n \star v_n \\ &\quad -\frac{N}{2} F\left(t_n \star v_n\right) \mathrm{d} x\\
&= \frac{1}{N}\int_{\mathbb{R}^N} |\nabla v_n|^2\mathrm{d} x+
\int_{\mathbb{R}^N} \frac{N-2}{4} f\left(v_n\right) v_n -\frac{N}{2} F\left(v_n\right) \mathrm{d} x+o_n(1) \\
& =\mathcal{I}_{\infty}\left(v_n\right)-\frac{1}{2^*} P_{\infty}\left(v_n\right) +o_n(1) \\
& =\mathcal{I}_{\infty}\left(v_n\right)+o_n(1)=\mathcal{I}_{\infty}\left(v\right)+o_n(1)=m_{\infty}(a)+o_n(1).
\end{aligned}
\end{equation*} This concludes the establishment that ![]() $m_\mu(a)=m_{\infty}(a)$.
$m_\mu(a)=m_{\infty}(a)$.
Now, we proceed to prove that ![]() $m_\mu(a)$ cannot be achievement. By proof by contradiction, we assume that
$m_\mu(a)$ cannot be achievement. By proof by contradiction, we assume that ![]() $u_a \in \mathcal{M}_\mu(a)$ attains
$u_a \in \mathcal{M}_\mu(a)$ attains ![]() $m_\mu(a)$. By lemma 2.3, there exists a unique
$m_\mu(a)$. By lemma 2.3, there exists a unique ![]() $t_{u_a} \gt 0$ such that
$t_{u_a} \gt 0$ such that ![]() $t_{u_a} \star u_a \in \mathcal{M}_{\infty}(a)$. It can be seen that
$t_{u_a} \star u_a \in \mathcal{M}_{\infty}(a)$. It can be seen that
 \begin{equation*}
\begin{aligned}
m_{\infty}(a) & \leq \mathcal{I}_{\infty}\left(t_{u_a} \star u_a\right)= \mathcal{I}_\mu\left(t_{u_a} \star u_a\right)+\frac{t_{u_a}^2}{2} \int_{\mathbb{R}^N} \frac{\mu}{|x|^2} u_a^2 \mathrm{d} x\\
& \lt \mathcal{I}_\mu\left(t_{u_a} \star u_a\right) \leq \mathcal{I}_\mu\left(u_a\right)=m_\mu(a)=m_{\infty}(a),
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
m_{\infty}(a) & \leq \mathcal{I}_{\infty}\left(t_{u_a} \star u_a\right)= \mathcal{I}_\mu\left(t_{u_a} \star u_a\right)+\frac{t_{u_a}^2}{2} \int_{\mathbb{R}^N} \frac{\mu}{|x|^2} u_a^2 \mathrm{d} x\\
& \lt \mathcal{I}_\mu\left(t_{u_a} \star u_a\right) \leq \mathcal{I}_\mu\left(u_a\right)=m_\mu(a)=m_{\infty}(a),
\end{aligned}
\end{equation*}which creates a contradiction.
Proof of theorem 1.4
The first part of theorem 1.4 has already been established in lemma 4.1. Next, we will prove that Eq. (1.6) has normalized solutions.
By lemma 2.10, there exists a Pohožaev–Palais–Smale sequence ![]() $\left\{u_n\right\} \subset S_{r, a}$ for
$\left\{u_n\right\} \subset S_{r, a}$ for ![]() $\mathcal{I}_\mu$ at level of
$\mathcal{I}_\mu$ at level of ![]() $\sigma_\mu(a)$. That is,
$\sigma_\mu(a)$. That is,
 \begin{equation*}
\mathcal{I}_\mu\left(u_n\right) \rightarrow \sigma_\mu(a), \quad \left( \left. \mathcal{I}_{\mu}\right|_{S_{r, a}} \right)
^{\prime}\left(u_n\right) \rightarrow 0, \quad P_{\mu}\left(u_n\right) \rightarrow 0,
\quad \text {as } n \rightarrow \infty \text {. }
\end{equation*}
\begin{equation*}
\mathcal{I}_\mu\left(u_n\right) \rightarrow \sigma_\mu(a), \quad \left( \left. \mathcal{I}_{\mu}\right|_{S_{r, a}} \right)
^{\prime}\left(u_n\right) \rightarrow 0, \quad P_{\mu}\left(u_n\right) \rightarrow 0,
\quad \text {as } n \rightarrow \infty \text {. }
\end{equation*} Similarly as the proof of theorem 1.1, ![]() $\{u_n\}$ is bounded in
$\{u_n\}$ is bounded in ![]() $H_{r}^1\left(\mathbb{R}^N\right)$, and there exists
$H_{r}^1\left(\mathbb{R}^N\right)$, and there exists ![]() $u\in H^1\left(\mathbb{R}^N\right)\backslash\{0\}$, such that
$u\in H^1\left(\mathbb{R}^N\right)\backslash\{0\}$, such that ![]() $u_n\rightarrow u $ in
$u_n\rightarrow u $ in ![]() $H^1\left(\mathbb{R}^N\right)$, and there exists a λ > 0, such that
$H^1\left(\mathbb{R}^N\right)$, and there exists a λ > 0, such that ![]() $( u, \lambda) \in H_r^1\left(\mathbb{R}^N\right) \times \mathbb{R}^{+}$ is a normalized solution of Eq. (1.6). Furthermore, u > 0 by the strong maximum principle. By lemma 4.1, we can deduce that
$( u, \lambda) \in H_r^1\left(\mathbb{R}^N\right) \times \mathbb{R}^{+}$ is a normalized solution of Eq. (1.6). Furthermore, u > 0 by the strong maximum principle. By lemma 4.1, we can deduce that ![]() $\sigma_\mu(a) \gt m_\mu(a)$.
$\sigma_\mu(a) \gt m_\mu(a)$.
5. Proof of theorem 1.6
Lemma 5.1. Assume that ![]() $N\geq 3$, a > 0, and
$N\geq 3$, a > 0, and  $1 \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$. Then, for any sequence
$1 \gt 2^* C_{N,\bar{p}}^{\bar{p}}\beta
a^{\frac{2}{N}}$. Then, for any sequence ![]() $ \mu_n\leq 0$ with
$ \mu_n\leq 0$ with ![]() $\mu_n \rightarrow 0^{-}$ as
$\mu_n \rightarrow 0^{-}$ as ![]() $n \rightarrow \infty$, we have
$n \rightarrow \infty$, we have  $\lim \limits_{n \rightarrow \infty} \sigma_{\mu_n}(a)=m_{\infty}(a)$.
$\lim \limits_{n \rightarrow \infty} \sigma_{\mu_n}(a)=m_{\infty}(a)$.
Proof. For the sake of clarity in our presentation, let’s define:
 \begin{equation*}
m_{r,\infty}(a):=\inf _{v \in \mathcal{M}^r_{\infty}(a)} \mathcal{I}_{\infty}(v),
\end{equation*}
\begin{equation*}
m_{r,\infty}(a):=\inf _{v \in \mathcal{M}^r_{\infty}(a)} \mathcal{I}_{\infty}(v),
\end{equation*}where
It’s clear that ![]() $m_{r,\infty}(a)=m_{\infty}(a)$ by lemma 2.8. Hence, we just need to prove
$m_{r,\infty}(a)=m_{\infty}(a)$ by lemma 2.8. Hence, we just need to prove  $\lim \limits_{n \rightarrow \infty} \sigma_{\mu_n}(a)=m_{r,\infty}(a)$. For
$\lim \limits_{n \rightarrow \infty} \sigma_{\mu_n}(a)=m_{r,\infty}(a)$. For ![]() $\mu \leq 0$, it’s evident that
$\mu \leq 0$, it’s evident that ![]() $\sigma_{\mu}(a) \geq m_{\infty}(a)$ and
$\sigma_{\mu}(a) \geq m_{\infty}(a)$ and ![]() $ \sigma_\mu(a)$ is non-increasing with respect to µ. Therefore, we just need to prove that
$ \sigma_\mu(a)$ is non-increasing with respect to µ. Therefore, we just need to prove that ![]() $m_{\infty}(a)$ is the greatest lower bound of
$m_{\infty}(a)$ is the greatest lower bound of ![]() $\left\{\sigma_{\mu_n}(a)\right\}$.
$\left\{\sigma_{\mu_n}(a)\right\}$.
Assume that ![]() $\omega \in \mathcal{M}^r_{\infty}(a)$ is the function that achieves
$\omega \in \mathcal{M}^r_{\infty}(a)$ is the function that achieves ![]() $m_{r,\infty}(a)$, implying
$m_{r,\infty}(a)$, implying ![]() $\mathcal{I}_{\infty}(\omega)=m_{r,\infty}(a)$. By lemma 2.3, we can find
$\mathcal{I}_{\infty}(\omega)=m_{r,\infty}(a)$. By lemma 2.3, we can find ![]() $0 \lt s_0 \lt 1 \lt s_1$ such that
$0 \lt s_0 \lt 1 \lt s_1$ such that
Furthermore, for any given ϵ > 0, there exists a positive integer Nϵ such that, for every ![]() $n \gt N_{\epsilon}$,
$n \gt N_{\epsilon}$,
 \begin{equation*}
-\frac{1}{2}s_1^2 \int_{\mathbb{R}^N} \frac{\mu_n}{|x|^2} \omega^2
\mathrm{d} x\leq \epsilon.
\end{equation*}
\begin{equation*}
-\frac{1}{2}s_1^2 \int_{\mathbb{R}^N} \frac{\mu_n}{|x|^2} \omega^2
\mathrm{d} x\leq \epsilon.
\end{equation*}We can deduce that
 \begin{equation*}
\begin{aligned}
\sigma_{\mu_n}(a) &\leq \max _{t\in [0,1]} \mathcal{I}_{\mu_n}(\gamma_o(t))
=\max _{t\in[0,1]} \mathcal{I}_{\infty}(\gamma_o(t))-\frac{s_1^2}{2} \int_{\mathbb{R}^N}
\frac{\mu_n}{|x|^2} \omega^2 \mathrm{d} x\\
& =\mathcal{I}_{\infty}(\omega)+\epsilon=m_{r,\infty}(a)+\epsilon.
\end{aligned}
\end{equation*}
\begin{equation*}
\begin{aligned}
\sigma_{\mu_n}(a) &\leq \max _{t\in [0,1]} \mathcal{I}_{\mu_n}(\gamma_o(t))
=\max _{t\in[0,1]} \mathcal{I}_{\infty}(\gamma_o(t))-\frac{s_1^2}{2} \int_{\mathbb{R}^N}
\frac{\mu_n}{|x|^2} \omega^2 \mathrm{d} x\\
& =\mathcal{I}_{\infty}(\omega)+\epsilon=m_{r,\infty}(a)+\epsilon.
\end{aligned}
\end{equation*} This clearly indicates that ![]() $m_{r,\infty}(a)$ is the infimum of
$m_{r,\infty}(a)$ is the infimum of ![]() $\left\{\sigma_{\mu_n}(a)\right\}$, and by lemma 2.8,
$\left\{\sigma_{\mu_n}(a)\right\}$, and by lemma 2.8,  $\lim \limits_{n \rightarrow \infty} \sigma_{\mu_n}(a) =m_{r,\infty}(a)=m_{\infty}(a)$.
$\lim \limits_{n \rightarrow \infty} \sigma_{\mu_n}(a) =m_{r,\infty}(a)=m_{\infty}(a)$.
Proof of theorem 1.6
Let ![]() $(u_n,\lambda_n) $ be the solution in theorem 1.4, which satisfies
$(u_n,\lambda_n) $ be the solution in theorem 1.4, which satisfies
 \begin{align}
-\Delta u_n -\frac{\mu_n}{|x|^2} u_n +\lambda_n u_n=f(u_n),
\end{align}
\begin{align}
-\Delta u_n -\frac{\mu_n}{|x|^2} u_n +\lambda_n u_n=f(u_n),
\end{align} where ![]() $\mu_n\to 0^{-}$ as
$\mu_n\to 0^{-}$ as ![]() $n\to \infty,$ and then
$n\to \infty,$ and then ![]() $P_{\mu_n}(u_n)=0$. As in the proof of theorem 1.4, we can establish that
$P_{\mu_n}(u_n)=0$. As in the proof of theorem 1.4, we can establish that ![]() $u_n\to u $ in
$u_n\to u $ in ![]() $H^1\left(\mathbb{R}^N\right)$ and
$H^1\left(\mathbb{R}^N\right)$ and ![]() $\lambda_n\to\lambda \gt 0$, and
$\lambda_n\to\lambda \gt 0$, and ![]() $(u, \lambda) \in H_r^1\left(\mathbb{R}^N\right) \times \mathbb{R}^{+}$ is the normalized ground state solution of Eq. (1.8). Additionally, by the strong maximum principle, u > 0.
$(u, \lambda) \in H_r^1\left(\mathbb{R}^N\right) \times \mathbb{R}^{+}$ is the normalized ground state solution of Eq. (1.8). Additionally, by the strong maximum principle, u > 0.
Proof of proposition 1.7
The proof of proposition 1.7 can be derived from the proof of proposition 1.2 by applying the same method. To avoid unnecessary repetition, we do not provide the proof here.
Acknowledgements
The authors express their gratitude to the referee for his/her invaluable suggestions and comments on the manuscript.