On Diophantine approximations of the solutions of q-functional equations

Abstract

Given a sequence of linear forms 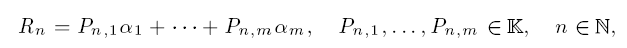in m ≥ 2 complex or p-adic numbers α₁, …,α_m ∈ K_v with appropriate growth conditions, Nesterenko proved a lower bound for the dimension d of the vector space Kα₁ + ··· + Kα_m over K, when K = Q and v is the infinite place. We shall generalize Nesterenko's dimension estimate over number fields K with appropriate places v, if the lower bound condition for |R_n| is replaced by the determinant condition. For the q-series approximations also a linear independence measure is given for the d linearly independent numbers. As an application we prove that the initial values F(t), F(qt), …, F(q^m−1t) of the linear homogeneous q-functional equation 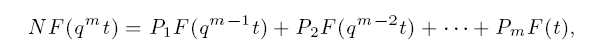where N = N(q, t), P_i = P_i(q, t) ∈ K[q, t] (i = 1, …, m), generate a vector space of dimension d ≥ 2 over K under some conditions for the coefficient polynomials, the solution F(t) and t, q ∈ K*.