Sufficiency theorems for local minimizers of the multiple integrals of the calculus of variations

Abstract

Let Ω ⊂ Rⁿ be a bounded domain and let f : Ω × R^N × R^N×n → R. Consider the functional 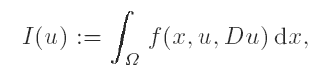over the class of Sobolev functions W^1,q(Ω;R^N) (1 ≤ q ≤ ∞) for which the integral on the right is well defined. In this paper we establish sufficient conditions on a given function u₀ and f to ensure that u₀ provides an L^r local minimizer for I where 1 ≤ r ≤ ∞. The case r = ∞ is somewhat known and there is a considerable literature on the subject treating the case min(n, N) = 1, mostly based on the field theory of the calculus of variations. The main contribution here is to present a set of sufficient conditions for the case 1 ≤ r < ∞. Our proof is based on an indirect approach and is largely motivated by an argument of Hestenes relying on the concept of ‘directional convergence’.