1. Introduction
We investigate the spatial Whitham (sWhitham) equation, proposed by Trillo et al. (Reference Trillo, Klein, Clauss and Onorato2016), for gravity waves propagating on the surface of water. This equation was motivated by the success of the temporal Korteweg–de Vries (tKdV) and temporal Whitham (tWhitham) equations in modelling the evolution of unidirectional waves in shallow water. The tWhitham equation uses a more accurate linear dispersive term than the tKdV equation and therefore may be useful for depths ranging from shallow to deep. The tWhitham equation can also approximate some singularity formation effects, see Whitham (Reference Whitham1967) and Ehrnström & Wahlén (Reference Ehrnström and Wahlén2019). Comparisons with experimental data suggest the tWhitham equation improves on the tKdV equation in the small-amplitude long-wave regime, see Trillo et al. (Reference Trillo, Klein, Clauss and Onorato2016) and Carter (Reference Carter2018).
A limitation of temporal models is that they describe how a given surface displacement profile evolves in time. However, many field and laboratory experiments provide temporal profiles of the surface displacement at fixed spatial locations and ask how these temporal profiles evolve in space. This problem arises in many wave phenomena, e.g. in optics, and there is a long use of ‘spatial evolution’ equations that describe how a signal, e.g. an optical image, changes along some direction in space. Despite their motivation, spatial equations have not been used systematically for nonlinear dispersive water-wave models. Herein, we examine a recently proposed model, the sWhitham equation of Trillo et al. (Reference Trillo, Klein, Clauss and Onorato2016), and show evidence that this equation can improve on predictions of the tWhitham, tKdV and spatial KdV (sKdV) equations. We consider this equation mathematically, numerically and experimentally and compare its properties and predictions with those of the tKdV, tWhitham and sKdV equations. Our results yield new information on the properties of the sWhitham equation and its strengths and limitations as a model of unidirectional water waves.
The motion of a two-dimensional, irrotational, incompressible, inviscid fluid on a horizontal, impermeable bed can be modelled by
where ![]() $\phi (x, z, t)$ represents the velocity potential of the fluid,
$\phi (x, z, t)$ represents the velocity potential of the fluid, ![]() $\eta (x, t)$ represents the surface displacement of the fluid,
$\eta (x, t)$ represents the surface displacement of the fluid, ![]() $g$ represents the acceleration due to gravity,
$g$ represents the acceleration due to gravity, ![]() $h_0$ represents the uniform depth of the fluid at rest and
$h_0$ represents the uniform depth of the fluid at rest and ![]() $x$,
$x$, ![]() $z$,
$z$, ![]() $t$ represent the horizontal, vertical and temporal coordinates, respectively. See Johnson (Reference Johnson2001) for details of this system. Since this system is a nonlinear free-boundary problem, approximate models are often used. Linear theory gives the dispersion relation
$t$ represent the horizontal, vertical and temporal coordinates, respectively. See Johnson (Reference Johnson2001) for details of this system. Since this system is a nonlinear free-boundary problem, approximate models are often used. Linear theory gives the dispersion relation
where ![]() $\kappa$ and
$\kappa$ and ![]() $\omega$ are the wavenumber and angular frequency of the linear wave, respectively.
$\omega$ are the wavenumber and angular frequency of the linear wave, respectively.
The dimensional tKdV equation (Korteweg & de Vries Reference Korteweg and de Vries1895),
is a small-amplitude, long-wave approximation of (1.1). The tKdV equation has been well studied mathematically (e.g. Ablowitz & Segur Reference Ablowitz and Segur1981; Miles Reference Miles1981; Lannes Reference Lannes2013) and experimentally (e.g. Russell Reference Russell1844; Zabusky & Galvin Reference Zabusky and Galvin1971; Hammack Reference Hammack1973; Hammack & Segur Reference Hammack and Segur1978). It has dispersion relation
Equation (1.4) is a unidirectional approximation of (1.2) that is valid in the long-wave (i.e. ![]() $\kappa h_0\rightarrow 0$) limit. In order to obtain a weakly nonlinear approximation to (1.1) that is valid for a wider range of
$\kappa h_0\rightarrow 0$) limit. In order to obtain a weakly nonlinear approximation to (1.1) that is valid for a wider range of ![]() $\kappa h_0$ values, Whitham (Reference Whitham1967, Reference Whitham1974) proposed the following dimensional equation:
$\kappa h_0$ values, Whitham (Reference Whitham1967, Reference Whitham1974) proposed the following dimensional equation:
as a model for the evolution of small-amplitude waves on shallow water. We refer to (1.5) as the dimensional tWhitham equation. The convolution term is defined by
where ![]() $\mathcal {K}(\kappa )$ is the non-dimensional Fourier multiplier given by
$\mathcal {K}(\kappa )$ is the non-dimensional Fourier multiplier given by
and ![]() $\tilde {\mathcal {F}}$ and
$\tilde {\mathcal {F}}$ and ![]() $\tilde {\mathcal {F}}^{-1}$ represent the Fourier and inverse Fourier transforms in
$\tilde {\mathcal {F}}^{-1}$ represent the Fourier and inverse Fourier transforms in ![]() $x$, respectively. We write the tWhitham equation and
$x$, respectively. We write the tWhitham equation and ![]() $\mathcal {K}$ in forms different than those used by Whitham in order to simplify the work below. The linear dispersion relation for the tWhitham equation is
$\mathcal {K}$ in forms different than those used by Whitham in order to simplify the work below. The linear dispersion relation for the tWhitham equation is
Equation (1.8) is one root of (1.2), which means that the tWhitham equation exactly models the wave speed of unidirectional linear waves for any ![]() $\kappa h_0$.
$\kappa h_0$.
Given an initial spatial profile of the surface displacement (i.e. given ![]() $\eta$ for all values of
$\eta$ for all values of ![]() $x$ at
$x$ at ![]() $t=0$), (1.3) and (1.5) provide predictions for the spatial profiles of
$t=0$), (1.3) and (1.5) provide predictions for the spatial profiles of ![]() $\eta$ for
$\eta$ for ![]() $t>0$. Because of this requirement for initial spatial data, these equations are known as evolution-in-time or ‘temporal’ equations. Many laboratory and field water-wave measurements are made by recording time series at fixed spatial locations (i.e. using stationary gauges or buoys to collect time series). Evolution-in-space or ‘spatial’ equations are required to directly compare with measurements of this type. In these situations, time series at the first measurement location are used as initial conditions in spatial equations that are solved to obtain predictions for the time series at the downstream measurement locations. Spatial equations are commonly used in nonlinear optics, e.g. in the study of pulse propagation in optical fibres, see for e.g. Manakov (Reference Manakov1974) and Agrawal (Reference Agrawal2019).
$t>0$. Because of this requirement for initial spatial data, these equations are known as evolution-in-time or ‘temporal’ equations. Many laboratory and field water-wave measurements are made by recording time series at fixed spatial locations (i.e. using stationary gauges or buoys to collect time series). Evolution-in-space or ‘spatial’ equations are required to directly compare with measurements of this type. In these situations, time series at the first measurement location are used as initial conditions in spatial equations that are solved to obtain predictions for the time series at the downstream measurement locations. Spatial equations are commonly used in nonlinear optics, e.g. in the study of pulse propagation in optical fibres, see for e.g. Manakov (Reference Manakov1974) and Agrawal (Reference Agrawal2019).
Three common methods used for deriving approximate spatial equations from (1.1) include the following: (i) interchanging the roles of ![]() $x$ and
$x$ and ![]() $t$ in the derivation of the temporal equations; (ii) starting with an approximate temporal equation and then applying the change of variables
$t$ in the derivation of the temporal equations; (ii) starting with an approximate temporal equation and then applying the change of variables ![]() $\eta _t\sim -\sqrt {gh_0}\,\eta _x$ for waves in shallow water; (iii) inverting the dispersion relation. Using either (i) or (ii) gives the dimensional sKdV equation
$\eta _t\sim -\sqrt {gh_0}\,\eta _x$ for waves in shallow water; (iii) inverting the dispersion relation. Using either (i) or (ii) gives the dimensional sKdV equation
Using method (i) for the nonlinear part and method (iii) for the linear part, Trillo et al. (Reference Trillo, Klein, Clauss and Onorato2016) proposed the following dimensional sWhitham equation:
 \begin{equation} \eta_x+\sqrt{\frac{h_0}{g}}\,\mathcal{K}^{{-}1}(\omega)*\eta-\frac{3}{2h_0\sqrt{gh_0}}\,\eta\eta_t=0, \end{equation}
\begin{equation} \eta_x+\sqrt{\frac{h_0}{g}}\,\mathcal{K}^{{-}1}(\omega)*\eta-\frac{3}{2h_0\sqrt{gh_0}}\,\eta\eta_t=0, \end{equation}where the convolution term is defined by
using ![]() $\mathcal {F}$ and
$\mathcal {F}$ and ![]() $\mathcal {F}^{-1}$ to represent the Fourier and inverse Fourier transforms in
$\mathcal {F}^{-1}$ to represent the Fourier and inverse Fourier transforms in ![]() $t$, respectively, and
$t$, respectively, and ![]() $\mathcal {K}^{-1}(\omega )$ as the inverse of
$\mathcal {K}^{-1}(\omega )$ as the inverse of ![]() $\mathcal {K}(\kappa )$. Although a closed-form expression for
$\mathcal {K}(\kappa )$. Although a closed-form expression for ![]() $\mathcal {K}^{-1}(\omega )$ is not known, the inverse is guaranteed to exist since
$\mathcal {K}^{-1}(\omega )$ is not known, the inverse is guaranteed to exist since ![]() $\mathcal {K}(\kappa )$ is one-to-one and onto for
$\mathcal {K}(\kappa )$ is one-to-one and onto for ![]() $\kappa \in \mathbb {R}$. In general, if the dispersion is monotonic and onto, then the linear initial-value problem can be written in spatial form. In other words, one can recover the spatial initial condition from the time series at
$\kappa \in \mathbb {R}$. In general, if the dispersion is monotonic and onto, then the linear initial-value problem can be written in spatial form. In other words, one can recover the spatial initial condition from the time series at ![]() $x=0$ and vice versa. Note that if weak surface tension were included, then
$x=0$ and vice versa. Note that if weak surface tension were included, then ![]() $\mathcal {K}(\kappa )$ would not be one-to-one and a unique inverse would not exist.
$\mathcal {K}(\kappa )$ would not be one-to-one and a unique inverse would not exist.
In the following, we consider both the mathematical properties of the sWhitham equation and its applications. Section 2 includes a summary of the mathematical properties including its conserved quantities, travelling-wave solutions and their stability. Section 3 contains comparisons between measurements from laboratory experiments and predictions obtained from the tKdV, sKdV, tWhitham and sWhitham equations, and their dissipative generalizations. Section 4 contains a summary of the results.
2. Properties of the spatial equations
In order to gain an understanding of the sWhitham equation, we present its properties along with the properties of the sKdV equation for comparative purposes. We define non-dimensional variables ![]() $u$,
$u$, ![]() $\chi$ and
$\chi$ and ![]() $\tau$ by
$\tau$ by
The corresponding non-dimensional sKdV equation is
and the non-dimensional sWhitham equation is
where ![]() $w=\sqrt {h_0/g}\,\omega$ is the non-dimensional circular frequency and
$w=\sqrt {h_0/g}\,\omega$ is the non-dimensional circular frequency and ![]() $K$ is the non-dimensional Fourier multiplier defined by
$K$ is the non-dimensional Fourier multiplier defined by
where ![]() $k=h_0\kappa$ is the non-dimensional wavenumber.
$k=h_0\kappa$ is the non-dimensional wavenumber.
2.1. Conserved quantities
The sKdV equation is known to have an infinite number of conservation laws, see for example Ablowitz & Segur (Reference Ablowitz and Segur1981). The first three conserved quantities are
where ![]() $\mathcal {Q}_3$ is the Hamiltonian. The sWhitham equation conserves
$\mathcal {Q}_3$ is the Hamiltonian. The sWhitham equation conserves ![]() $\tilde {\mathcal {Q}}_1$,
$\tilde {\mathcal {Q}}_1$,![]() $\tilde {\mathcal {Q}}_2$ and its Hamiltonian
$\tilde {\mathcal {Q}}_2$ and its Hamiltonian
The quantities ![]() $\mathcal {Q}_1$ and
$\mathcal {Q}_1$ and ![]() $\mathcal {Q}_2$ are sometimes referred to as the mass and momentum integrals, respectively. These interpretations are discussed in depth in Ali & Kalisch (Reference Ali and Kalisch2014). We emphasize that the quantities
$\mathcal {Q}_2$ are sometimes referred to as the mass and momentum integrals, respectively. These interpretations are discussed in depth in Ali & Kalisch (Reference Ali and Kalisch2014). We emphasize that the quantities ![]() $\tilde {\mathcal {Q}}_1$ to
$\tilde {\mathcal {Q}}_1$ to ![]() $\tilde {\mathcal {Q}}_4$ are constant in
$\tilde {\mathcal {Q}}_4$ are constant in ![]() $\chi$, the non-dimensional spatial variable. The periodic generalizations of these conserved quantities,
$\chi$, the non-dimensional spatial variable. The periodic generalizations of these conserved quantities, ![]() $\tilde {\mathcal {Q}}_1$,
$\tilde {\mathcal {Q}}_1$, ![]() $\tilde {\mathcal {Q}}_2$,
$\tilde {\mathcal {Q}}_2$, ![]() $\tilde {\mathcal {Q}}_3$,
$\tilde {\mathcal {Q}}_3$, ![]() $\tilde {\mathcal {Q}}_4$, for the tKdV, sKdV, tWhitham and sWhitham equations are included in the Appendix A.
$\tilde {\mathcal {Q}}_4$, for the tKdV, sKdV, tWhitham and sWhitham equations are included in the Appendix A.
2.2. Travelling-wave solutions
The sKdV equation admits a two-parameter family of travelling-wave solutions given by
where
Here ![]() $T$ is the (temporal) period of the solution,
$T$ is the (temporal) period of the solution, ![]() $U_2$ is the wave height of the solution,
$U_2$ is the wave height of the solution, ![]() $m\in [0,1)$ is known as the elliptic parameter of the Jacobi elliptic function
$m\in [0,1)$ is known as the elliptic parameter of the Jacobi elliptic function ![]() $\mbox {cn}({\cdot },m)$, and
$\mbox {cn}({\cdot },m)$, and ![]() $E_1(m)$ and
$E_1(m)$ and ![]() $E_2(m)$ are the complete elliptic integrals of the first and second kinds, respectively. See Byrd & Friedman (Reference Byrd and Friedman1971) for details of elliptic functions. Without loss of generality, the integration constant,
$E_2(m)$ are the complete elliptic integrals of the first and second kinds, respectively. See Byrd & Friedman (Reference Byrd and Friedman1971) for details of elliptic functions. Without loss of generality, the integration constant, ![]() $U_0$, was chosen so that the solutions have zero mean (i.e.
$U_0$, was chosen so that the solutions have zero mean (i.e. ![]() $\mathcal {Q}_1=0$) because (2.7) is invariant under the transformation
$\mathcal {Q}_1=0$) because (2.7) is invariant under the transformation
where ![]() $\nu$ is any real constant. The two-parameter family (the free parameters are
$\nu$ is any real constant. The two-parameter family (the free parameters are ![]() $T$ and
$T$ and ![]() $m$) of solutions given in (2.7) comprises all zero-mean, travelling-wave solutions to the sKdV equation. A profile of the form
$m$) of solutions given in (2.7) comprises all zero-mean, travelling-wave solutions to the sKdV equation. A profile of the form ![]() $u(\tau -\gamma \chi )$ of the spatial equation is interpreted as the
$u(\tau -\gamma \chi )$ of the spatial equation is interpreted as the ![]() $u(-\gamma (x-{\gamma }^{-1}t))$ travelling-wave profile of the temporal evolution. This means that the non-dimensional real parameter
$u(-\gamma (x-{\gamma }^{-1}t))$ travelling-wave profile of the temporal evolution. This means that the non-dimensional real parameter ![]() $\gamma$ corresponds to the inverse of the non-dimensional wave speed.
$\gamma$ corresponds to the inverse of the non-dimensional wave speed.
Figure 1(a) contains plots of four ![]() $2{\rm \pi}$-periodic solutions of the sKdV equation. The wave heights,
$2{\rm \pi}$-periodic solutions of the sKdV equation. The wave heights, ![]() $H$, and
$H$, and ![]() $\gamma$ values for these solutions are included in the legend. The sKdV equation does not admit a solution with maximal height, nor does it admit a solution with minimal
$\gamma$ values for these solutions are included in the legend. The sKdV equation does not admit a solution with maximal height, nor does it admit a solution with minimal ![]() $\gamma$ value. As
$\gamma$ value. As ![]() $m\rightarrow 1$,
$m\rightarrow 1$, ![]() $H$ increases without bound, and
$H$ increases without bound, and ![]() $\gamma$ decreases without bound. Once the height of the solution becomes large enough, the
$\gamma$ decreases without bound. Once the height of the solution becomes large enough, the ![]() $\gamma$ value becomes negative. For solutions with period
$\gamma$ value becomes negative. For solutions with period ![]() $T=2{\rm \pi}$, this sign transition occurs for solutions with height
$T=2{\rm \pi}$, this sign transition occurs for solutions with height ![]() $H\approx 4.28$. Since
$H\approx 4.28$. Since ![]() $\gamma$ is the inverse of wave speed,
$\gamma$ is the inverse of wave speed, ![]() $\gamma$ going through zero corresponds to the wave speed going to infinity. This non-physical result may be due to the fact that the sWhitham equation is a generalization of the sKdV equation, which is a model of small-amplitude waves, and these waves are well outside the small-amplitude regime.
$\gamma$ going through zero corresponds to the wave speed going to infinity. This non-physical result may be due to the fact that the sWhitham equation is a generalization of the sKdV equation, which is a model of small-amplitude waves, and these waves are well outside the small-amplitude regime.

Figure 1. Plots of four ![]() $2{\rm \pi}$-periodic, zero-mean, travelling-wave solutions to (a) the sKdV equation and (b) the sWhitham equation. The
$2{\rm \pi}$-periodic, zero-mean, travelling-wave solutions to (a) the sKdV equation and (b) the sWhitham equation. The ![]() $\gamma$ values and wave heights,
$\gamma$ values and wave heights, ![]() $H$, are included in the legends.
$H$, are included in the legends.
Ehrnström & Kalisch (Reference Ehrnström and Kalisch2009) proved that the tWhitham equation admits small-amplitude, periodic travelling-wave solutions and computed some of these solutions. Ehrnström & Wahlén (Reference Ehrnström and Wahlén2019) proved Whitham's conjecture that the tWhitham equation admits a travelling-wave solution with maximal wave height and that this solution is cusped. Carter (Reference Carter2024) numerically examined the properties of solutions to the tWhitham equation close to the maximal height.
We consider periodic travelling-wave solutions of the sWhitham equation of the form
where ![]() $\gamma$ is a real constant and
$\gamma$ is a real constant and ![]() $f$ is a smooth, real-valued function of
$f$ is a smooth, real-valued function of ![]() $\xi =\tau -\gamma \chi$ with non-dimensional temporal period
$\xi =\tau -\gamma \chi$ with non-dimensional temporal period ![]() $T$. Substituting (2.10) into (2.3) and integrating with respect to
$T$. Substituting (2.10) into (2.3) and integrating with respect to ![]() $\xi$ once gives
$\xi$ once gives
where ![]() $B$ is the constant of integration. This equation is invariant under the transformation
$B$ is the constant of integration. This equation is invariant under the transformation
where ![]() $\nu$ is any real constant. Therefore, without loss of generality, we only consider travelling-wave solutions of the sWhitham equation that have zero mean.
$\nu$ is any real constant. Therefore, without loss of generality, we only consider travelling-wave solutions of the sWhitham equation that have zero mean.
Equation (2.11) can be solved approximately by assuming ![]() $f$ has a Fourier expansion of the form
$f$ has a Fourier expansion of the form
 \begin{equation} f(\xi)=\sum_{j={-}N}^{N} \hat{f}(j)\exp\left(\frac{2{\rm \pi} {\rm i}j\xi}{T}\right), \end{equation}
\begin{equation} f(\xi)=\sum_{j={-}N}^{N} \hat{f}(j)\exp\left(\frac{2{\rm \pi} {\rm i}j\xi}{T}\right), \end{equation}
where ![]() $N$ is a large positive integer and the
$N$ is a large positive integer and the ![]() $\hat {f}$ are complex constants. Since
$\hat {f}$ are complex constants. Since ![]() $f$ has zero mean,
$f$ has zero mean, ![]() $\hat {f}(0)=0$. For simplicity, we assume that the solutions are real and even. Thus,
$\hat {f}(0)=0$. For simplicity, we assume that the solutions are real and even. Thus, ![]() $\hat {f}(-j)=\hat {f}(j)$ for
$\hat {f}(-j)=\hat {f}(j)$ for ![]() $j=1,\dots,N$. Substituting (2.13) into (2.11) gives
$j=1,\dots,N$. Substituting (2.13) into (2.11) gives
 \begin{align} {-}\gamma\hat{f}(j)+\frac{TK^{{-}1}\left(\dfrac{2{\rm \pi} w}{T}\right)}{2{\rm \pi} iw}\hat{f}(j)-\frac{3}{4}\sum_{l={-}N+j}^{N}\hat{f}(j-l)\hat{f}(l)=0,\quad\text{for}\ j=1,2,\dots,N. \end{align}
\begin{align} {-}\gamma\hat{f}(j)+\frac{TK^{{-}1}\left(\dfrac{2{\rm \pi} w}{T}\right)}{2{\rm \pi} iw}\hat{f}(j)-\frac{3}{4}\sum_{l={-}N+j}^{N}\hat{f}(j-l)\hat{f}(l)=0,\quad\text{for}\ j=1,2,\dots,N. \end{align}
We solved this system of nonlinear algebraic equations for the ![]() $\hat {f}$ using Newton's method (see Ehrnström & Kalisch (Reference Ehrnström and Kalisch2009) and Carter et al. (Reference Carter, Kalisch, Kharif and Abid2022) for the details in closely related problems).
$\hat {f}$ using Newton's method (see Ehrnström & Kalisch (Reference Ehrnström and Kalisch2009) and Carter et al. (Reference Carter, Kalisch, Kharif and Abid2022) for the details in closely related problems).
Figure 1(b) includes plots of four ![]() $2{\rm \pi}$-periodic, zero-mean, travelling-wave solutions to the sWhitham equation. As the value of
$2{\rm \pi}$-periodic, zero-mean, travelling-wave solutions to the sWhitham equation. As the value of ![]() $\gamma$ decreases, the height of the solution increases. For the sWhitham equation, the
$\gamma$ decreases, the height of the solution increases. For the sWhitham equation, the ![]() $\gamma$ value for
$\gamma$ value for ![]() $2{\rm \pi}$-periodic solutions becomes negative when
$2{\rm \pi}$-periodic solutions becomes negative when ![]() $H\approx 3.70$. We do not see evidence of a wave of maximum height that is analogous to the cusped wave seen in the tWhitham equation, see Ehrnström & Wahlén (Reference Ehrnström and Wahlén2019). This result is consistent with the fact that stronger dispersion typically has a regularizing effect.
$H\approx 3.70$. We do not see evidence of a wave of maximum height that is analogous to the cusped wave seen in the tWhitham equation, see Ehrnström & Wahlén (Reference Ehrnström and Wahlén2019). This result is consistent with the fact that stronger dispersion typically has a regularizing effect.
Figure 2 includes plots of four travelling-wave solutions to the sWhitham equation with period ![]() $T=10{\rm \pi}$. Just as in the
$T=10{\rm \pi}$. Just as in the ![]() $T=2{\rm \pi}$ case, there does not appear to be a solution with maximal height, nor a solution with minimal
$T=2{\rm \pi}$ case, there does not appear to be a solution with maximal height, nor a solution with minimal ![]() $\gamma$ value. However, once the height becomes large enough, the solutions no longer increase monotonically on
$\gamma$ value. However, once the height becomes large enough, the solutions no longer increase monotonically on ![]() $\xi \in (-5{\rm \pi},0)$. This is demonstrated in the inset plot in figure 2. This non-monotonic behaviour is not a numerical artefact. We checked that the purple solution is in fact a travelling-wave solution by evolving it for multiple periods in evolution code for the sWhitham equation. This non-monotonic behaviour is not exhibited by solutions to the sKdV, tKdV or tWhitham equations. Finally, the sWhitham equation does not meet the criteria for monotonic growth presented in Bruell & Pei (Reference Bruell and Pei2023). For clarity, we define height by
$\xi \in (-5{\rm \pi},0)$. This is demonstrated in the inset plot in figure 2. This non-monotonic behaviour is not a numerical artefact. We checked that the purple solution is in fact a travelling-wave solution by evolving it for multiple periods in evolution code for the sWhitham equation. This non-monotonic behaviour is not exhibited by solutions to the sKdV, tKdV or tWhitham equations. Finally, the sWhitham equation does not meet the criteria for monotonic growth presented in Bruell & Pei (Reference Bruell and Pei2023). For clarity, we define height by ![]() $H=\max (u)-\min (u)$ regardless if the solution is monotonic on
$H=\max (u)-\min (u)$ regardless if the solution is monotonic on ![]() $\xi \in (-{T}/{2},0)$ or not.
$\xi \in (-{T}/{2},0)$ or not.

Figure 2. Plots of four travelling-wave solutions to the sWhitham equation with period ![]() $T=10{\rm \pi}$. The inset plot shows that the solutions are not monotonic on
$T=10{\rm \pi}$. The inset plot shows that the solutions are not monotonic on ![]() $\xi \in (-5{\rm \pi},0)$ when the solution height is sufficiently large.
$\xi \in (-5{\rm \pi},0)$ when the solution height is sufficiently large.
2.3. Stability of travelling-wave solutions
Benjamin & Feir (Reference Benjamin and Feir1967) showed that small-amplitude periodic travelling-wave solutions to (1.1) are stable with respect to the modulational instability (long-wavelength perturbations) when ![]() ${2{\rm \pi} }/{L}<1.363$ and are unstable with respect to the modulational instability when
${2{\rm \pi} }/{L}<1.363$ and are unstable with respect to the modulational instability when ![]() ${2{\rm \pi} }/{L}>1.363$, where
${2{\rm \pi} }/{L}>1.363$, where ![]() $L$ is the spatial wavelength. Bottman & Deconinck (Reference Bottman and Deconinck2009) and Deconinck & Nivala (Reference Deconinck and Nivala2010) proved that all travelling-wave solutions of the tKdV equation are stable regardless of their height or (spatial) period. Due to the similarities between the tKdV and sKdV equations, these results also establish that all travelling-wave solutions of sKdV are stable regardless of their height or period. Hur & Johnson (Reference Hur and Johnson2015) proved that travelling-wave solutions to the tWhitham equation with sufficiently small amplitude are stable with respect to the modulational instability if
$L$ is the spatial wavelength. Bottman & Deconinck (Reference Bottman and Deconinck2009) and Deconinck & Nivala (Reference Deconinck and Nivala2010) proved that all travelling-wave solutions of the tKdV equation are stable regardless of their height or (spatial) period. Due to the similarities between the tKdV and sKdV equations, these results also establish that all travelling-wave solutions of sKdV are stable regardless of their height or period. Hur & Johnson (Reference Hur and Johnson2015) proved that travelling-wave solutions to the tWhitham equation with sufficiently small amplitude are stable with respect to the modulational instability if ![]() $2{\rm \pi} /L<1.146$ and are unstable with respect to the modulational instability otherwise. The more general work of Binswanger et al. (Reference Binswanger, Hoefer, Ilan and Sprenger2021) also shows that the small-amplitude modulational instability cutoff occurs at
$2{\rm \pi} /L<1.146$ and are unstable with respect to the modulational instability otherwise. The more general work of Binswanger et al. (Reference Binswanger, Hoefer, Ilan and Sprenger2021) also shows that the small-amplitude modulational instability cutoff occurs at ![]() $2{\rm \pi} /L=1.146$ in the tWhitham equation. Sanford et al. (Reference Sanford, Kodama, Carter and Kalisch2014) numerically corroborated these results. They numerically showed that all periodic travelling-wave solutions of the tWhitham equation with large enough amplitude, regardless of wavelength, are unstable. Additionally, they showed that these large-amplitude solutions are unstable with respect to perturbations of all wavelengths greater than or equal to that of the unperturbed solution. Finally, they showed that the instability growth rate increases monotonically with the solution height.
$2{\rm \pi} /L=1.146$ in the tWhitham equation. Sanford et al. (Reference Sanford, Kodama, Carter and Kalisch2014) numerically corroborated these results. They numerically showed that all periodic travelling-wave solutions of the tWhitham equation with large enough amplitude, regardless of wavelength, are unstable. Additionally, they showed that these large-amplitude solutions are unstable with respect to perturbations of all wavelengths greater than or equal to that of the unperturbed solution. Finally, they showed that the instability growth rate increases monotonically with the solution height.
Applying equation (23) of Binswanger et al. (Reference Binswanger, Hoefer, Ilan and Sprenger2021) to the sWhitham equation establishes that all small-amplitude periodic travelling-wave solutions are stable with respect to the modulational instability regardless of their period. This result is qualitatively similar to the tKdV and sKdV results, but is qualitatively different than the tWhitham result.
In order to numerically study the stability of travelling-wave solutions to the sWhitham equation, we employ the Fourier–Floquet–Hill method of Deconinck & Kutz (Reference Deconinck and Kutz2006). First, enter a coordinate frame moving with the solution via the change of variables
This converts (2.3) to
and converts travelling-wave solutions of (2.3) into stationary (i.e. ![]() $\partial _\chi u=0$) solutions of (2.16). Next, consider perturbed solutions of the form
$\partial _\chi u=0$) solutions of (2.16). Next, consider perturbed solutions of the form
where ![]() $u$ is a periodic travelling-wave solution of the sWhitham equation,
$u$ is a periodic travelling-wave solution of the sWhitham equation, ![]() $\epsilon$ is a small real constant and
$\epsilon$ is a small real constant and ![]() $\epsilon v$ is the leading-order part of the perturbation. Substituting (2.17) into (2.16) and linearizing gives
$\epsilon v$ is the leading-order part of the perturbation. Substituting (2.17) into (2.16) and linearizing gives
where ‘prime’ means derivative with respect to ![]() $\xi$. Without loss of generality assume
$\xi$. Without loss of generality assume
where ![]() $V$ is a complex-valued function,
$V$ is a complex-valued function, ![]() $\lambda$ is a complex constant whose real part corresponds to the growth rate of the instability and c.c. stands for complex conjugate. Substituting (2.19) into (2.18) and rearranging gives
$\lambda$ is a complex constant whose real part corresponds to the growth rate of the instability and c.c. stands for complex conjugate. Substituting (2.19) into (2.18) and rearranging gives
All bounded solutions to this equation (i.e. solutions with any period) have the form ![]() $V(\xi ) = {\rm e}^{{\rm i} \mu \xi } W(\xi )$, where
$V(\xi ) = {\rm e}^{{\rm i} \mu \xi } W(\xi )$, where ![]() $W$ is a
$W$ is a ![]() $T$-periodic function and
$T$-periodic function and ![]() $\mu \in [-{{\rm \pi} }/{T},{{\rm \pi} }/{T}]$ is a constant known as the Floquet parameter, see Deconinck & Kutz (Reference Deconinck and Kutz2006). This gives
$\mu \in [-{{\rm \pi} }/{T},{{\rm \pi} }/{T}]$ is a constant known as the Floquet parameter, see Deconinck & Kutz (Reference Deconinck and Kutz2006). This gives
 \begin{equation} V(\xi) = \mbox{e}^{{\rm i} \mu \xi} \sum_{j={-}\infty}^{\infty} {\hat W}(j)\mbox{e}^{2 {\rm \pi}{\rm i} j \xi /T}, \end{equation}
\begin{equation} V(\xi) = \mbox{e}^{{\rm i} \mu \xi} \sum_{j={-}\infty}^{\infty} {\hat W}(j)\mbox{e}^{2 {\rm \pi}{\rm i} j \xi /T}, \end{equation}
where the ![]() $\hat {W}(j)$ are complex numbers. In our numerical computations, we use the truncation
$\hat {W}(j)$ are complex numbers. In our numerical computations, we use the truncation
 \begin{equation} V(\xi) = \mbox{e}^{{\rm i} \mu \xi} \sum_{j={-}N}^{N}{\hat W}(j)\mbox{e}^{2 {\rm \pi}{\rm i} j \xi /T}, \end{equation}
\begin{equation} V(\xi) = \mbox{e}^{{\rm i} \mu \xi} \sum_{j={-}N}^{N}{\hat W}(j)\mbox{e}^{2 {\rm \pi}{\rm i} j \xi /T}, \end{equation}
where ![]() $N$ is a large positive integer. If there exists a bounded solution to (2.20) with
$N$ is a large positive integer. If there exists a bounded solution to (2.20) with ![]() $\lambda$ that has a positive real part, then the perturbation grows exponentially in
$\lambda$ that has a positive real part, then the perturbation grows exponentially in ![]() $\chi$ and the corresponding solution to the sWhitham equation is said to be unstable. If all solutions of (2.20) have
$\chi$ and the corresponding solution to the sWhitham equation is said to be unstable. If all solutions of (2.20) have ![]() $\lambda$ values that are purely imaginary, then the corresponding solution is said to be spectrally stable.
$\lambda$ values that are purely imaginary, then the corresponding solution is said to be spectrally stable.
Travelling-wave solutions to the sWhitham equation with period ![]() $T=2{\rm \pi}$, height
$T=2{\rm \pi}$, height ![]() $H<0.842$ and
$H<0.842$ and ![]() $\gamma >1.14$ are spectrally stable. As the wave height surpasses
$\gamma >1.14$ are spectrally stable. As the wave height surpasses ![]() $H=0.842$ (and
$H=0.842$ (and ![]() $\gamma$ falls below
$\gamma$ falls below ![]() $1.14$), the solutions become unstable. The spectra corresponding to solutions with heights just above the critical value are oval-like shapes centred at the origin. The oval-like shapes correspond to perturbations with
$1.14$), the solutions become unstable. The spectra corresponding to solutions with heights just above the critical value are oval-like shapes centred at the origin. The oval-like shapes correspond to perturbations with ![]() $\mu$ values near
$\mu$ values near ![]() $\pm 0.5$. (A perturbation with
$\pm 0.5$. (A perturbation with ![]() $\mu =0.5$ has a period that is twice that of the unperturbed solution.) As the height increases further, more
$\mu =0.5$ has a period that is twice that of the unperturbed solution.) As the height increases further, more ![]() $\mu$ values lead to instability and the ovals transition into lemniscates centred at the origin. All non-zero
$\mu$ values lead to instability and the ovals transition into lemniscates centred at the origin. All non-zero ![]() $\mu$ values lead to instability when the complete lemniscate is formed. For solutions with period
$\mu$ values lead to instability when the complete lemniscate is formed. For solutions with period ![]() $2{\rm \pi}$, the complete lemniscate is first observed near
$2{\rm \pi}$, the complete lemniscate is first observed near ![]() $H=0.845$. These sWhitham stability results are qualitatively different than the tWhitham results where the first unstable solutions have spectra with lemniscates centred at the origin. Such spectra are created by perturbations with
$H=0.845$. These sWhitham stability results are qualitatively different than the tWhitham results where the first unstable solutions have spectra with lemniscates centred at the origin. Such spectra are created by perturbations with ![]() $\mu$ values near zero and are representative of the modulational instability.
$\mu$ values near zero and are representative of the modulational instability.
Figure 3(a) includes plots of the stability spectra for the sWhitham solutions shown in figure 1(b). Figure 3(b) includes plots of ![]() $\max ({\rm Re}(\lambda ))$, i.e. the maximal instability growth rate, versus
$\max ({\rm Re}(\lambda ))$, i.e. the maximal instability growth rate, versus ![]() $\mu$ for the same solutions. The solution with smallest height (coloured blue in the plots) is spectrally stable since it has a purely imaginary spectrum. The fact that this solution is stable is consistent with the Binswanger et al. (Reference Binswanger, Hoefer, Ilan and Sprenger2021) asymptotic result that all travelling-wave solutions of the sWhitham equation with sufficiently small amplitudes are stable with respect to the modulational instability. Additionally, this solution does not exhibit the ‘bubble’ instabilities seen in the Euler equations, see Deconinck & Oliveras (Reference Deconinck and Oliveras2011), or in bidirectional generalizations of the tWhitham equation, see Deconinck & Trichtchenko (Reference Deconinck and Trichtchenko2015). The other three solutions plotted in figure 1 are unstable.
$\mu$ for the same solutions. The solution with smallest height (coloured blue in the plots) is spectrally stable since it has a purely imaginary spectrum. The fact that this solution is stable is consistent with the Binswanger et al. (Reference Binswanger, Hoefer, Ilan and Sprenger2021) asymptotic result that all travelling-wave solutions of the sWhitham equation with sufficiently small amplitudes are stable with respect to the modulational instability. Additionally, this solution does not exhibit the ‘bubble’ instabilities seen in the Euler equations, see Deconinck & Oliveras (Reference Deconinck and Oliveras2011), or in bidirectional generalizations of the tWhitham equation, see Deconinck & Trichtchenko (Reference Deconinck and Trichtchenko2015). The other three solutions plotted in figure 1 are unstable.

Figure 3. Plots of the stability results for the sWhitham solutions with ![]() $T=2{\rm \pi}$ shown in figure 1(b). The coloured curves in this figure correspond to the solutions of the same colours in figure 1(b). Panel (a) shows the stability spectra and (b) shows the maximum growth rate versus the Floquet parameter.
$T=2{\rm \pi}$ shown in figure 1(b). The coloured curves in this figure correspond to the solutions of the same colours in figure 1(b). Panel (a) shows the stability spectra and (b) shows the maximum growth rate versus the Floquet parameter.
The solution with second smallest height (coloured orange) is unstable. Its stability spectrum is an oval-like shape centred at the origin that is transitioning into a lemniscate, see figure 3(a). This sWhitham solution is only unstable with respect to perturbations with approximately ![]() $|\mu |\in [0.1,0.5]$. Since
$|\mu |\in [0.1,0.5]$. Since ![]() $\mu$ near zero does not lead to instability, this solution is stable with respect to the modulational instability. The solution is most unstable with respect to perturbations with
$\mu$ near zero does not lead to instability, this solution is stable with respect to the modulational instability. The solution is most unstable with respect to perturbations with ![]() $\mu =0.5$. This means that perturbations with period
$\mu =0.5$. This means that perturbations with period ![]() $T=4{\rm \pi}$, i.e. twice the period of the underlying solution, grow fastest. These results are qualitatively different than those obtained for moderate-amplitude
$T=4{\rm \pi}$, i.e. twice the period of the underlying solution, grow fastest. These results are qualitatively different than those obtained for moderate-amplitude ![]() $2{\rm \pi}$-periodic travelling-wave solutions of the tWhitham equation, see Sanford et al. (Reference Sanford, Kodama, Carter and Kalisch2014).
$2{\rm \pi}$-periodic travelling-wave solutions of the tWhitham equation, see Sanford et al. (Reference Sanford, Kodama, Carter and Kalisch2014).
The solution with the third smallest height (coloured green in the plots) is unstable with respect to perturbations with any non-zero value of ![]() $\mu$. The stability spectrum is a lemniscate centred at the origin. The instability growth rates for this solution are larger than those for the solution with the second smallest height. It is most unstable with respect to the perturbation with
$\mu$. The stability spectrum is a lemniscate centred at the origin. The instability growth rates for this solution are larger than those for the solution with the second smallest height. It is most unstable with respect to the perturbation with ![]() $\mu =0.5$, a perturbation with
$\mu =0.5$, a perturbation with ![]() $T=4{\rm \pi}$. Finally, the solution with the largest height (coloured magenta) is also unstable with respect to perturbations with any non-zero
$T=4{\rm \pi}$. Finally, the solution with the largest height (coloured magenta) is also unstable with respect to perturbations with any non-zero ![]() $\mu$ and its stability spectrum is a lemniscate centred at the origin. However, the growth rates of the instabilities of this solution are smaller than the growth rates of the solution with third smallest wave height, see figure 3(b).
$\mu$ and its stability spectrum is a lemniscate centred at the origin. However, the growth rates of the instabilities of this solution are smaller than the growth rates of the solution with third smallest wave height, see figure 3(b).
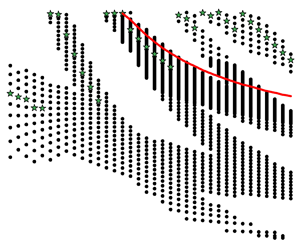
As the wave height continues to increase, there are alternating bands of stability and instability. Figure 4 shows regions of ![]() $(T,H)$-space for which periodic travelling-wave solutions to the sWhitham equation are unstable with respect to
$(T,H)$-space for which periodic travelling-wave solutions to the sWhitham equation are unstable with respect to ![]() $\mu =0.5$ perturbations (black dots). For this plot, we only examined
$\mu =0.5$ perturbations (black dots). For this plot, we only examined ![]() $\mu =0.5$ perturbations because for all
$\mu =0.5$ perturbations because for all ![]() $(T,H)$ pairs we examined, the
$(T,H)$ pairs we examined, the ![]() $\mu =0.5$ perturbation had the largest instability growth rate. There does not appear to be a simple relationship between
$\mu =0.5$ perturbation had the largest instability growth rate. There does not appear to be a simple relationship between ![]() $(T,H)$ and stability. For example, all four of the
$(T,H)$ and stability. For example, all four of the ![]() $10{\rm \pi}$-periodic solutions shown in figure 2 are stable, but not all
$10{\rm \pi}$-periodic solutions shown in figure 2 are stable, but not all ![]() $10{\rm \pi}$-periodic solutions are stable. The approximate values of
$10{\rm \pi}$-periodic solutions are stable. The approximate values of ![]() $(T,H)$ where
$(T,H)$ where ![]() $\gamma$ turns negative are shown by the red curve. This curve does not appear to have a simple relationship with stability. Figure 4 shows that there are bands of instability and stability. This is qualitatively different than what happens in the tWhitham case where all large-amplitude solutions are unstable and the growth rate increases monotonically with wave height. It is unintuitive that some large-amplitude solutions are stable. This unintuitive result may be attributed to the fact that the sWhitham equation is a model for small-amplitude waves and these solutions are outside of that range of validity.
$\gamma$ turns negative are shown by the red curve. This curve does not appear to have a simple relationship with stability. Figure 4 shows that there are bands of instability and stability. This is qualitatively different than what happens in the tWhitham case where all large-amplitude solutions are unstable and the growth rate increases monotonically with wave height. It is unintuitive that some large-amplitude solutions are stable. This unintuitive result may be attributed to the fact that the sWhitham equation is a model for small-amplitude waves and these solutions are outside of that range of validity.

Figure 4. Plot of the regions of ![]() $(T,H)$-space for which periodic travelling-wave solutions of the sWhitham equation are unstable (black dots) with respect to the
$(T,H)$-space for which periodic travelling-wave solutions of the sWhitham equation are unstable (black dots) with respect to the ![]() $\mu =0.5$ instability. Solutions corresponding to
$\mu =0.5$ instability. Solutions corresponding to ![]() $(T,H)$ in the white bands are stable with respect to
$(T,H)$ in the white bands are stable with respect to ![]() $\mu =0.5$ perturbations. The green stars represent the approximate location of the most unstable solutions for that period. The red curve shows the approximate values of
$\mu =0.5$ perturbations. The green stars represent the approximate location of the most unstable solutions for that period. The red curve shows the approximate values of ![]() $(T,H)$ where
$(T,H)$ where ![]() $\gamma$ becomes negative.
$\gamma$ becomes negative.
Finally, we did not find any solutions to the sWhitham equation that are unstable with respect to the superharmonic instability, a perturbation that has the same period as the solution (i.e. ![]() $\mu =0$). Additionally, neither
$\mu =0$). Additionally, neither ![]() $\mathcal {Q}_2$ nor
$\mathcal {Q}_2$ nor ![]() $\mathcal {Q}_3$ oscillate for the sWhitham equation as solution height increases. These results are qualitatively different than the tWhitham case where travelling-wave solutions with large-enough amplitude are unstable with respect to the superharmonic instability, see Carter et al. (Reference Carter, Francius, Kharif, Kalisch and Abid2023).
$\mathcal {Q}_3$ oscillate for the sWhitham equation as solution height increases. These results are qualitatively different than the tWhitham case where travelling-wave solutions with large-enough amplitude are unstable with respect to the superharmonic instability, see Carter et al. (Reference Carter, Francius, Kharif, Kalisch and Abid2023).
3. Comparisons with experiments
The tKdV equation has been shown to compare favourably with experiments, see for example Russell (Reference Russell1844), Zabusky & Galvin (Reference Zabusky and Galvin1971), Hammack (Reference Hammack1973), Hammack & Segur (Reference Hammack and Segur1974) and Hammack & Segur (Reference Hammack and Segur1978). Trillo et al. (Reference Trillo, Klein, Clauss and Onorato2016) showed that both the sKdV and sWhitham equations accurately model the evolution of experimental waves of depression on shallow water. Carter (Reference Carter2018) showed that the tWhitham equation more accurately predicts the evolution of experimental waves of depression than do the tKdV and Serre equations.
In this section, we present comparisons between predictions obtained from numerical simulations of the model equations and measurements from four different series of laboratory experiments including waves of depression and elevation on shallow water (§§ 3.2 and 3.3), solitons on shallow water (§ 3.4), waves of depression and elevation on deep water (§ 3.5) and wave packets on deep water (§ 3.6).
3.1. Numerical methods
In order to make comparisons with measurements from laboratory experiments, the model equations need to be solved numerically. The tKdV and tWhitham equations are solved numerically using fourth-order operator-splitting in time (see Yoshida Reference Yoshida1990) and periodic boundary conditions in space. The nonlinear parts of the equations are solved using fourth-order Runge–Kutta in time and a Fourier pseudospectral evaluation of the nonlinearity on a uniform grid (pointwise multiplication in space and spectral computation of the derivative). The linear part of the equations is solved exactly in Fourier space. We use the fast Fourier transform to move between the spatial and spectral variables. The length of the numerical tank must be large enough that waves do not leave one end of the domain, wrap around and impact waves on the other end of the domain. Additionally, the spatial gridpoints must be selected to line up with the experimental gauge locations. As a check on the results, the numerical preservation of the conserved quantities of the equations, see the Appendix A, was monitored.
The sKdV and sWhitham equations are solved using the same methods except that space and time are interchanged. The inverse ![]() $K^{-1}(w)$ is evaluated numerically using Newton's method. As a check on the results, the preservation of the conserved quantities, see the Appendix A, was monitored.
$K^{-1}(w)$ is evaluated numerically using Newton's method. As a check on the results, the preservation of the conserved quantities, see the Appendix A, was monitored.
3.2. Waves of depression and elevation on shallow water
Hammack & Segur (Reference Hammack and Segur1978) conducted a series of water-wave experiments in a long, narrow tank with ![]() $h_0=10$ cm, and a wavemaker at one end. The wavemaker was a rectangular, vertically moving piston located on the bottom of the tank next to a rigid wall at one end of the tank. The piston spanned the width of the tank and had a length of 61 cm, so the initial wavelength was 122 cm. This experiment is in the shallow-water regime because the ratio
$h_0=10$ cm, and a wavemaker at one end. The wavemaker was a rectangular, vertically moving piston located on the bottom of the tank next to a rigid wall at one end of the tank. The piston spanned the width of the tank and had a length of 61 cm, so the initial wavelength was 122 cm. This experiment is in the shallow-water regime because the ratio ![]() $r={h_0}/{L}=0.082\ll 1$ and
$r={h_0}/{L}=0.082\ll 1$ and ![]() $\tanh (2{\rm \pi} r)=0.47$. The experiments were initialized by rapidly moving the piston downward a prescribed amount. Time series were collected by wave gauges located
$\tanh (2{\rm \pi} r)=0.47$. The experiments were initialized by rapidly moving the piston downward a prescribed amount. Time series were collected by wave gauges located ![]() $61+500j$ cm for
$61+500j$ cm for ![]() $j=0,\dots,4$ from the upstream end of the tank. This means that the first gauge was located at the downstream edge of the wavemaker. The tank was long enough that waves reflecting from the downstream end of the tank did not impact the time series.
$j=0,\dots,4$ from the upstream end of the tank. This means that the first gauge was located at the downstream edge of the wavemaker. The tank was long enough that waves reflecting from the downstream end of the tank did not impact the time series.
We compare predictions from the tKdV, sKdV, tWhitham and sWhitham equations. The time series collected by the first gauge were used as initial conditions for the simulations of the spatial equations. The initial conditions for the temporal equations require knowledge of the surface displacement for all values of ![]() $x$ at the initial time, but that information was not recorded in these experiments. To approximate it, we used the following function as initial condition for the temporal equations:
$x$ at the initial time, but that information was not recorded in these experiments. To approximate it, we used the following function as initial condition for the temporal equations:
 \begin{equation} \eta(x,0)=\left\{\begin{array}{ll} 0 & -7869\leqslant x<{-}183,\\ -\tfrac{1}{2}A_0+\tfrac{1}{2}A_0\,\mbox{sn}(0.0925434x,0.9999^2) & -183\leqslant x\leqslant 61,\\ 0 & 61< x\leqslant 7747, \end{array} \right.\end{equation}
\begin{equation} \eta(x,0)=\left\{\begin{array}{ll} 0 & -7869\leqslant x<{-}183,\\ -\tfrac{1}{2}A_0+\tfrac{1}{2}A_0\,\mbox{sn}(0.0925434x,0.9999^2) & -183\leqslant x\leqslant 61,\\ 0 & 61< x\leqslant 7747, \end{array} \right.\end{equation}
where ![]() $A_0$ is the amplitude of the piston motion in centimetres,
$A_0$ is the amplitude of the piston motion in centimetres, ![]() $x$ is measured in centimetres and
$x$ is measured in centimetres and ![]() $\mbox {sn}({\cdot },m)$ is a Jacobi elliptic function with elliptic modulus
$\mbox {sn}({\cdot },m)$ is a Jacobi elliptic function with elliptic modulus ![]() $m$, see Byrd & Friedman (Reference Byrd and Friedman1971). This function represents a trough of 122 cm centred at
$m$, see Byrd & Friedman (Reference Byrd and Friedman1971). This function represents a trough of 122 cm centred at ![]() $x=-61$ cm.
$x=-61$ cm.
Figure 5 contains plots comparing the experimental time series with the predictions obtained from the tKdV and sKdV equations for the experiment with ![]() $A_0=1.5$ cm. Both equations do a reasonable job of predicting the experimental measurements. However, the sKdV equation more accurately predicts the phase speed. (This is especially visible at the downstream gauges.) It is important to note that part of the error in the tKdV prediction is due to the fact that the initial surface is estimated. This is a shortcoming of the temporal equations. Both models overpredict the amplitudes at the downstream gauges and this overprediction increases as the waves travel down the tank. This overprediction is due to the fact that both tKdV and sKdV are conservative models and the experiment contains dissipation. See § 3.3 for a discussion of the role dissipation plays.
$A_0=1.5$ cm. Both equations do a reasonable job of predicting the experimental measurements. However, the sKdV equation more accurately predicts the phase speed. (This is especially visible at the downstream gauges.) It is important to note that part of the error in the tKdV prediction is due to the fact that the initial surface is estimated. This is a shortcoming of the temporal equations. Both models overpredict the amplitudes at the downstream gauges and this overprediction increases as the waves travel down the tank. This overprediction is due to the fact that both tKdV and sKdV are conservative models and the experiment contains dissipation. See § 3.3 for a discussion of the role dissipation plays.

Figure 5. The experimental time series (blue curve) from the Hammack & Segur (Reference Hammack and Segur1978) experiment using ![]() $A_0=1.5$ cm with the predictions obtained from the tKdV (orange curve) and sKdV (red curve) equations. The plots are ordered from (a) to (e) by increasing distance from the wavemaker.
$A_0=1.5$ cm with the predictions obtained from the tKdV (orange curve) and sKdV (red curve) equations. The plots are ordered from (a) to (e) by increasing distance from the wavemaker.
Figure 6 contains plots comparing the experimental time series with the predictions obtained from the tWhitham and sWhitham equations for the same experiment. The differences between the tWhitham and sWhitham predictions are smaller than the differences between the tKdV and sKdV predictions. Note that part of the error in the tWhitham prediction is due to the fact that the initial surface displacement is estimated. Again, this highlights a shortcoming of the temporal equations. Both the tWhitham and sWhitham equations overpredict the amplitudes of the waves at the downstream gauges due to their conservative nature, see § 3.3.

Figure 6. The experimental times series (blue curve) from the Hammack & Segur (Reference Hammack and Segur1978) experiment using ![]() $A_0=1.5$ cm with the predictions obtained from the tWhitham (light green curve) and sWhitham (dark green curve) equations. The plots are ordered from (a) to (e) by increasing distance from the wavemaker.
$A_0=1.5$ cm with the predictions obtained from the tWhitham (light green curve) and sWhitham (dark green curve) equations. The plots are ordered from (a) to (e) by increasing distance from the wavemaker.
The predictions from all four models (tKdV, sKdV, tWhitham and sWhitham) for the Hammack & Segur (Reference Hammack and Segur1978) experiment with ![]() $A_0=0.5$ cm (plots omitted for conciseness) are more accurate than those in the
$A_0=0.5$ cm (plots omitted for conciseness) are more accurate than those in the ![]() $A_0=1.5$ cm case. However, the results were qualitatively the same: the spatial equations provide more accurate predictions than do the temporal equations; the Whitham equations provide more accurate predictions than do the KdV equations; all four equations overpredicted the wave amplitudes. Finally, we note that linear theory is not sufficient to model the time series from either the
$A_0=1.5$ cm case. However, the results were qualitatively the same: the spatial equations provide more accurate predictions than do the temporal equations; the Whitham equations provide more accurate predictions than do the KdV equations; all four equations overpredicted the wave amplitudes. Finally, we note that linear theory is not sufficient to model the time series from either the ![]() $A_0=0.5$ cm or
$A_0=0.5$ cm or ![]() $A_0=1.5$ cm experiments. Predictions obtained from linear theory (plots omitted for conciseness) are significantly worse than any of the models examined herein.
$A_0=1.5$ cm experiments. Predictions obtained from linear theory (plots omitted for conciseness) are significantly worse than any of the models examined herein.
Using the wave tank described above, Hammack & Segur (Reference Hammack and Segur1974) conducted another series of experiments in which the piston was rapidly moved upwards at the start of the experiment. Time series were recorded at ![]() $x=61$,
$x=61$, ![]() $161$,
$161$, ![]() $961$ and
$961$ and ![]() $2061$ cm from the upstream end of the tank. The experimental data from the first gauge was used as the initial condition for the spatial equations. For the temporal equations, we used the following initial condition:
$2061$ cm from the upstream end of the tank. The experimental data from the first gauge was used as the initial condition for the spatial equations. For the temporal equations, we used the following initial condition:
 \begin{equation} \eta(x,0)=\left\{\begin{array}{ll} 0 & -7869\leqslant x<{-}183,\\ 0.075-0.075\,\mbox{sn}(0.0925434x,0.9999^2) & -183\leqslant x\leqslant 61,\\ 0 & 61< x\leqslant 7747. \end{array} \right.\end{equation}
\begin{equation} \eta(x,0)=\left\{\begin{array}{ll} 0 & -7869\leqslant x<{-}183,\\ 0.075-0.075\,\mbox{sn}(0.0925434x,0.9999^2) & -183\leqslant x\leqslant 61,\\ 0 & 61< x\leqslant 7747. \end{array} \right.\end{equation}Figure 7 contains plots comparing the experimental times series with the predictions obtained from the tWhitham and sWhitham equations. The predictions from the tKdV and sKdV equations are similar. Both equations do reasonable jobs modelling the experimental data and the accuracy of the models is approximately the same as it is for the waves of depression experiments discussed above.

Figure 7. The experimental times series (blue curve) from one of the Hammack & Segur (Reference Hammack and Segur1974) experiments with the predictions obtained from the tWhitham (light green curve) and sWhitham (dark green curve) equations. The plots are ordered from (a) to (d) by increasing distance from the wavemaker.
3.3. Dissipative models of waves of depression on shallow water
Figures 5 and 6 show that the tKdV, sKdV, tWhitham and sWhitham equations overpredict the amplitudes of the waves measured in the experiments. This overprediction is due to the fact that the equations are conservative, while the experiments contain dissipation. Figure 8 shows that the dimensional quantity
where ![]() $\tau$ is the dimensional length of the time series, decays nearly exponentially as the waves travel down the tank for both the
$\tau$ is the dimensional length of the time series, decays nearly exponentially as the waves travel down the tank for both the ![]() $A_0=0.5$ cm and
$A_0=0.5$ cm and ![]() $A_0=1.5$ cm experiments. In order to address this, we consider the dimensional dissipative sKdV equation
$A_0=1.5$ cm experiments. In order to address this, we consider the dimensional dissipative sKdV equation
and propose the following ad hoc, dimensional dissipative generalization of the sWhitham equation:
 \begin{equation} \eta_x+\sqrt{\frac{h_0}{g}}\,\mathcal{K}^{{-}1}(\omega)*\eta-\frac{3}{2h_0\sqrt{gh_0}}\,\eta\eta_t+\delta\eta=0. \end{equation}
\begin{equation} \eta_x+\sqrt{\frac{h_0}{g}}\,\mathcal{K}^{{-}1}(\omega)*\eta-\frac{3}{2h_0\sqrt{gh_0}}\,\eta\eta_t+\delta\eta=0. \end{equation}
Here ![]() $\delta$ is a non-negative constant representing the sum total of all forms of dissipation in the experiment. We refer to (3.4) and (3.5) as the dissipative sKdV (dsKdV) and the dissipative sWhitham (dsWhitham) equations, respectively. These equations predict that
$\delta$ is a non-negative constant representing the sum total of all forms of dissipation in the experiment. We refer to (3.4) and (3.5) as the dissipative sKdV (dsKdV) and the dissipative sWhitham (dsWhitham) equations, respectively. These equations predict that ![]() $\mathcal {M}$ will decay exponentially as
$\mathcal {M}$ will decay exponentially as ![]() $x$ increases (i.e. as the waves travel down the tank). The single free parameter,
$x$ increases (i.e. as the waves travel down the tank). The single free parameter, ![]() $\delta$, is determined by best fitting the measured exponential decay of
$\delta$, is determined by best fitting the measured exponential decay of ![]() $\mathcal {M}$. The
$\mathcal {M}$. The ![]() $\delta$ values for the
$\delta$ values for the ![]() $A_0=0.5$ cm and
$A_0=0.5$ cm and ![]() $A_0=1.5$ cm experiments are
$A_0=1.5$ cm experiments are ![]() $7.14\times 10^{-5}\ {\rm cm}^{-1}$ and
$7.14\times 10^{-5}\ {\rm cm}^{-1}$ and ![]() $2.07\times 10^{-4}\ {\rm cm}^{-1}$, respectively. This form of dissipation assumes that waves of all periods decay with the same rate. It is not a wavenumber-dependent form of dissipation.
$2.07\times 10^{-4}\ {\rm cm}^{-1}$, respectively. This form of dissipation assumes that waves of all periods decay with the same rate. It is not a wavenumber-dependent form of dissipation.

Figure 8. Plots of ![]() $\mathcal {M}$ versus
$\mathcal {M}$ versus ![]() $x$ for the Hammack & Segur (Reference Hammack and Segur1978) experiments with (a)
$x$ for the Hammack & Segur (Reference Hammack and Segur1978) experiments with (a) ![]() $A_0=0.5$ cm and (b)
$A_0=0.5$ cm and (b) ![]() $A_0=1.5$ cm. The blue dots represent the experimental measurements, the magenta curves represent the best-fit exponentials of the experimental data and the black circles represent the dsWhitham predictions.
$A_0=1.5$ cm. The blue dots represent the experimental measurements, the magenta curves represent the best-fit exponentials of the experimental data and the black circles represent the dsWhitham predictions.
Figure 9 shows comparisons between the experimental time series and predictions from the dsKdV and dsWhitham equations. The dsKdV equation does a reasonable job, but incorrectly models the phase speeds. The dsWhitham equation does an excellent job modelling the ![]() $A_0=1.5$ cm experimental time series. The predictions obtained from the dsWhitham equation are much better than those obtained from the conservative models. The results for the
$A_0=1.5$ cm experimental time series. The predictions obtained from the dsWhitham equation are much better than those obtained from the conservative models. The results for the ![]() $A_0=0.5$ cm experiment (not shown) are similarly excellent.
$A_0=0.5$ cm experiment (not shown) are similarly excellent.

Figure 9. The experimental time series (blue curve) from the Hammack & Segur (Reference Hammack and Segur1978) experiment using ![]() $A_0=1.5$ cm and the predictions obtained from the dsKdV (grey curve) and dsWhitham (black curve) equations.
$A_0=1.5$ cm and the predictions obtained from the dsKdV (grey curve) and dsWhitham (black curve) equations.
3.4. Solitons on shallow water
We conducted experiments on solitons in a 1524 cm long (reflected waves did not impact the evolving soliton), 25.4 cm wide wave channel in the W.G. Pritchard Fluid Mechanics Laboratory. The tank is described in detail in Vasan et al. (Reference Vasan, Oliveras, Henderson and Deconinck2017). Briefly, the channel was cleaned with alcohol and filled with water to a desired depth. A wind was blown over the water surface along the length of the channel, creating a surface current that carried surface contaminants to the other end, where they were vacuumed with a wet-vac. The depth was then measured to be 5.30 cm. We generated solitons using a horizontal displacement of a piston: a vertical plate that spanned the width and height of the channel. The piston was programmed using the approach of Goring & Raichlen (Reference Goring and Raichlen1980), to take into account the real-time displacement of the plate. This approach and the details for our wavemaker are spelled out in Hammack et al. (Reference Hammack, Henderson, Guyenne and Yi2004). Herein, the wavemaker produced a repeatable soliton solution of the KdV equation with the desired height of 2.00 cm. Hammack et al. (Reference Hammack, Henderson, Guyenne and Yi2004) also generated a soliton solution of KdV with that height in water of depth 5.00 cm. They measured the spatial wavelength of the soliton to be approximately 80 cm. We did not measure a spatial wavelength, but the ratio in the present experiments is nearby this value. Therefore, the ratio of depth to wavelength, ![]() $r=h_0/\lambda \approx 0.066$, meaning these experiments are in the shallow-water regime. A capacitance-type wave gauge was used to measure the surface displacement. For a fixed soliton amplitude, we conducted seven experiments with the wave gauge moved to the different
$r=h_0/\lambda \approx 0.066$, meaning these experiments are in the shallow-water regime. A capacitance-type wave gauge was used to measure the surface displacement. For a fixed soliton amplitude, we conducted seven experiments with the wave gauge moved to the different ![]() $x$-locations,
$x$-locations, ![]() $x=50$, 150, 250, 350, 450, 550, 650 cm away from the piston in its rest position. In these seven experiments, the start time of the paddle was not synced to the start time of data collection, so that the time series had to be shifted by hand to match comparisons; hence, we did not test accuracy of wave speed.
$x=50$, 150, 250, 350, 450, 550, 650 cm away from the piston in its rest position. In these seven experiments, the start time of the paddle was not synced to the start time of data collection, so that the time series had to be shifted by hand to match comparisons; hence, we did not test accuracy of wave speed.
Figure 10 shows a comparison of the experimental time series and the predictions obtained from the dsWhitham equation for all seven gauges. The predictions line-up very closely with the experimental measurements. Figure 11 shows comparisons of the experimental time series and the predictions from the sKdV, dsKdV, sWhitham and dsWhitham equations for the last gauge. Although these experiments involve waves of elevation instead of waves of depression, the results are similar to those presented in the previous two subsections. The sWhitham predictions are more accurate than the sKdV predictions. The dsWhitham equation more accurately models the experimental time series than did the sWhitham equation. Other plots (omitted for conciseness) show that the dsWhitham equation is more accurate than the tKdV and sKdV equations. Additionally, the spatial equations more accurately predict the wave evolution than do the temporal equations.

Figure 10. The experimental time series (blue curve) from the experiment described in § 3.4 along with the prediction obtained from the dsWhitham equation (black curve). The plots are ordered by increasing distance from the wavemaker.

Figure 11. The experimental time series (blue curve) from the final gauge of the experiment described in § 3.4 along with the predictions obtained from (a) the sKdV (red curve) and sWhitham (dark green curve) equations and (b) the dsKdV (grey curve) and dsWhitham (black curve) equations.
3.5. Waves of depression and elevation on deep water
The tWhitham and sWhitham equations were proposed as models for waves on shallow water. However, they accurately reproduce the phase speeds of all unidirectional linear waves, regardless of the (uniform) depth of water. Because of this, it is reasonable to ask whether these equations accurately predict the evolution of waves on deep water.
In this section, we address this question by comparing predictions from the tWhitham and sWhitham equations with time series from our experiments of waves on deep water that are similar to those discussed above in §§ 3.2 and 3.3. In particular, we created an initial, localized, positive surface displacement and an initial, localized negative surface displacement. These surface displacements were created in the same tank used for the soliton experiments (see § 3.4) but with a horizontally aligned piston that had a vertical displacement. The piston for the experiments in this section was a horizontal plate that was 25.4 cm long in the ![]() $x$-direction, 1 cm tall in the vertical direction and spanned the width of the tank. The initial wave had a length of
$x$-direction, 1 cm tall in the vertical direction and spanned the width of the tank. The initial wave had a length of ![]() $\lambda =50.8$ cm, and the depth was approximately
$\lambda =50.8$ cm, and the depth was approximately ![]() $h_0=20$ cm. The resulting ratio of fluid depth to wavelength was
$h_0=20$ cm. The resulting ratio of fluid depth to wavelength was ![]() $r=h_0/\lambda =0.393$, with
$r=h_0/\lambda =0.393$, with ![]() $\tanh (2{\rm \pi} h_0/\lambda )=0.986$, so that the waves were effectively in the deep-water regime.
$\tanh (2{\rm \pi} h_0/\lambda )=0.986$, so that the waves were effectively in the deep-water regime.
The plate was impulsively lowered or raised 0.75 cm. To obtain the motion, the operator moved a Two-Servo Joystick, which sent a signal to a Servo Travel Tuner, in which we had programmed the desired plate displacement, and then to a Hi-Tec Linear Servo, which provided the plate motion. (All parts were from www.servocity.com.) There was a vertical barrier behind the plate so that the resulting waves were forced to travel in the ![]() $x>0$ direction.
$x>0$ direction.
For the positive initial displacement, the tank was filled, and the surface was cleaned as described above to a depth of 20.18 cm. The plate was levelled parallel to the quiescent water surface and submerged 0.25 cm. It was dropped 0.75 cm impulsively, creating a localized positive surface displacement. Four in situ, capacitance wave gauges, located at ![]() $x=4.0$, 225.5, 461.4, 679.0 cm from the edge of the plate, provided time series. Figure 12 shows the results. The data from the first gauge are used as the initial conditions for the spatial equations, so the model output and measurements agree exactly at that location. At the second gauge site, all three models predict well the oscillations that develop near the initial displacement and the wave packet that forms farther downstream. Dissipation becomes important in the surface displacement evolution by the third wave gauge. The conservative predictions agree qualitatively with the data, while the dsWhitham agrees both qualitatively and quantitatively, matching quite well even the radiation. By the fourth gauge, the agreement between the conservative predictions and the data worsens. The sWhitham prediction more accurately models the phase velocities than does the sKdV equation. The dsWhitham equation continues to produce a good quantitative comparison with the data.
$x=4.0$, 225.5, 461.4, 679.0 cm from the edge of the plate, provided time series. Figure 12 shows the results. The data from the first gauge are used as the initial conditions for the spatial equations, so the model output and measurements agree exactly at that location. At the second gauge site, all three models predict well the oscillations that develop near the initial displacement and the wave packet that forms farther downstream. Dissipation becomes important in the surface displacement evolution by the third wave gauge. The conservative predictions agree qualitatively with the data, while the dsWhitham agrees both qualitatively and quantitatively, matching quite well even the radiation. By the fourth gauge, the agreement between the conservative predictions and the data worsens. The sWhitham prediction more accurately models the phase velocities than does the sKdV equation. The dsWhitham equation continues to produce a good quantitative comparison with the data.

Figure 12. Comparisons of predictions from the (a) sKdV equation, (b) sWhitham equation and (c) dsWhitham equation when the initial surface displacement is positive. The blue curves correspond to the measured surface displacement, the red, dark green and black curves correspond to the sKdV, sWhitham and dsWhitham predictions, respectively. The plots are ordered from (i) to (iv) by increasing distance from the plate/wavemaker.
For the negative initial displacement, the tank was filled, and the surface was cleaned as described above to a depth of 20.22 cm. The plate was levelled parallel to the quiescent water surface and submerged 0.75 cm. It was lifted 0.75 cm impulsively, creating a localized negative surface displacement. The four in situ, capacitance wave gauges, located at ![]() $x=4.0$, 225.6, 462.5, 679.0 cm from the edge of the plate, provided time series. Figure 13 shows the results. The data from the first gauge is used as the initial condition, so the model output and measurements agree. Similar to the negative displacement experiments, all three models predict reasonably well the measured surface displacement at the second gauge site. Results from the sKdV and sWhitham equations are in qualitative agreement with data at the third and fourth gauge sites, while the dsWhitham equation predicts quite well the measured time series at the third and fourth gauge sites.
$x=4.0$, 225.6, 462.5, 679.0 cm from the edge of the plate, provided time series. Figure 13 shows the results. The data from the first gauge is used as the initial condition, so the model output and measurements agree. Similar to the negative displacement experiments, all three models predict reasonably well the measured surface displacement at the second gauge site. Results from the sKdV and sWhitham equations are in qualitative agreement with data at the third and fourth gauge sites, while the dsWhitham equation predicts quite well the measured time series at the third and fourth gauge sites.

Figure 13. Comparisons of predictions from the (a) the sKdV equation, (b) sWhitham equation and (c) dsWhitham equation when the initial surface displacement is negative. The blue curves correspond to the measured surface displacement, the red, dark green and black curves correspond to the sKdV, sWhitham and dsWhitham predictions, respectively. The plots are ordered from (i) to (iv) by increasing distance from the plate/wavemaker.
The sKdV predictions are less accurate than the sWhitham predictions due to phase velocity issues. Comparisons with sKdV and dsKdV are similar, although they do not align with the experimental data as well.
3.6. Wave packets on deep water
In order to further test the range of validity of the sWhitham and dsWhitham equations, we compare their predictions with experimental measurements of wave packets on deep water. Segur et al. (Reference Segur, Henderson, Carter, Hammack, Li, Pheiff and Socha2005) showed that the dissipative nonlinear Schrödinger (dNLS) equation provided much more accurate predictions, both quantitatively and qualitatively, than did the nonlinear Schrödinger (NLS) equation for the experiments discussed in this section. They also showed that although plane-wave solutions to the NLS equation are unstable with respect to the modulational instability (also known as the sideband instability), uniform-amplitude solutions to the dNLS equation are stable with respect to the modulational instability. Finally, they showed that although the dNLS equation is stable with respect to the modulational instability, the sidebands may grow a limited amount.
In the Segur et al. (Reference Segur, Henderson, Carter, Hammack, Li, Pheiff and Socha2005) experiments, the wave tank was 1311 cm long, 25.4 cm wide, had glass sidewalls and bottom and had a constant water depth of ![]() $h_0=20$ cm. A plunger-type wavemaker that spanned the width of the tank, had an exponential cross-section, and oscillated vertically was located at one end of the tank. The wavemaker created slowly modulated wavetrains with waves of wavelength
$h_0=20$ cm. A plunger-type wavemaker that spanned the width of the tank, had an exponential cross-section, and oscillated vertically was located at one end of the tank. The wavemaker created slowly modulated wavetrains with waves of wavelength ![]() $\lambda \approx 15$ cm. Since the ratio
$\lambda \approx 15$ cm. Since the ratio ![]() $r=h_0/\lambda \approx 1.33$ and
$r=h_0/\lambda \approx 1.33$ and ![]() $\tanh (2{\rm \pi} h_0/\lambda )\approx 1.00$, this experiment is in the deep-water regime.
$\tanh (2{\rm \pi} h_0/\lambda )\approx 1.00$, this experiment is in the deep-water regime.
Figure 14 shows comparisons between the time series recorded in an experiment from § 6.2 of Segur et al. (Reference Segur, Henderson, Carter, Hammack, Li, Pheiff and Socha2005) and the predictions from the sWhitham equation. Due to the complexity of the time series, we compare the magnitudes of the dominant Fourier coefficients instead of the time series themselves. The carrier wave frequency was ![]() $f_0=3.33$ Hz. The perturbation frequency was
$f_0=3.33$ Hz. The perturbation frequency was ![]() $f_p=0.17$ Hz. So the sideband frequencies were
$f_p=0.17$ Hz. So the sideband frequencies were ![]() $f_{\pm n}=f_0\pm n f_p$, for
$f_{\pm n}=f_0\pm n f_p$, for ![]() $n=1$, 2, 3. Figure 14(a) shows the evolution of the carrier wave and figure 14(b–g) show the six most dominant sidebands. The plots show that the sWhitham equation does not accurately predict the evolution of these Fourier coefficients. This discrepancy appears to be related to the modulational instability. The experimental sidebands grow in magnitude as the waves travel down the tank (the experimental parameters were chosen so that the sidebands would grow), while the sWhitham equation predicts no such growth. This is consistent with the fact that the sWhitham equation is not unstable with respect to the modulational instability. An accurate model of this experimental data must allow for the sidebands to grow.
$n=1$, 2, 3. Figure 14(a) shows the evolution of the carrier wave and figure 14(b–g) show the six most dominant sidebands. The plots show that the sWhitham equation does not accurately predict the evolution of these Fourier coefficients. This discrepancy appears to be related to the modulational instability. The experimental sidebands grow in magnitude as the waves travel down the tank (the experimental parameters were chosen so that the sidebands would grow), while the sWhitham equation predicts no such growth. This is consistent with the fact that the sWhitham equation is not unstable with respect to the modulational instability. An accurate model of this experimental data must allow for the sidebands to grow.

Figure 14. Plots comparing the experimental measurements (blue dots) from the deep-water experiment described in § 6.2 of Segur et al. (Reference Segur, Henderson, Carter, Hammack, Li, Pheiff and Socha2005) with the predictions from the sWhitham equation (dark green dots). Panel (a) is the carrier wave and (b–g) are the six most dominant sidebands.
Figure 15 shows comparisons between the same experimental data and the predictions from the dsWhitham equation. These plots show that the dsWhitham equation does not accurately model the experimental data either. Though, note that the dsWhitham equation accurately models the evolution of the carrier wave. Similar results are obtained when the other three deep-water experiments from Carter, Henderson & Butterfield (Reference Carter, Henderson and Butterfield2018) are examined. Therefore, it does not appear that the sWhitham or the dsWhitham equation can accurately model the evolution of wave packets on deep water.

Figure 15. Plots comparing the experimental measurements (blue dots) from the deep-water experiment described in § 6.2 of Segur et al. (Reference Segur, Henderson, Carter, Hammack, Li, Pheiff and Socha2005) with the predictions from the dsWhitham equation (black dots). Panel (a) is the carrier wave and (b–g) are the six most dominant sidebands.
4. Summary
We examined both the mathematical properties and applications of the sWhitham equation. Our main result is that the sWhitham equation performs better than the tWhitham equation and the spatial and temporal KdV equations for the experiments in both shallow and deep water considered here.
The sWhitham equation can be used to model how time series of surface displacement evolve as waves travel in space. Our analysis shows that the sWhitham equation admits periodic travelling-wave solutions, but does not appear to admit a periodic travelling-wave solution with maximal height. The sWhitham equation admits some solutions that are non-monotonic. Small- and moderate-amplitude travelling-wave solutions to the sWhitham equation are stable with respect to the modulational instability. Some larger-amplitude travelling-wave solutions are stable while others are unstable depending on wave period and wave height. There does not appear to be a simple relation between wave period and height that determines stability or instability.
Our comparisons with experiments show that for waves of depression and solitons on shallow water, the sWhitham equation provides more accurate predictions for experimental time series than do the tWhitham, tKdV and sKdV equations. Part of the reason that the spatial predictions are more accurate than the temporal predictions is that the initial conditions need to be approximated in the temporal case. Although the sWhitham equation was proposed as a model for shallow-water waves, we show that the sWhitham equation accurately models the evolution of initial waves of depression and elevation on deep water. The predictions obtained from the sWhitham equation are improved by adding dissipation. Finally, neither the sWhitham nor the dsWhitham equation accurately models the evolution of wave packets on deep water.
Acknowledgements
We thank R. Geist for constructing the laboratory apparatus, including the mechanical components, the motion control and the computer interfaces, for the shallow-water soliton and all of the deep-water experiments. We thank V. Vasan for writing the wavemaker program for experiments on the shallow-water soliton and the deep-water wave packets. We thank R. Crable for building and maintaining the capacitance wave gauges. We thank K. Hammack for digitizing the experimental data from Hammack & Segur (Reference Hammack and Segur1978). We thank E. Byrnes for helpful discussions.
Funding
J.D.C. and P.P. thank the Dirección General de Asuntos del Personal Académico (DGAPA) at the Universidad Nacional Autónoma de México (UNAM) for a Programa de Estancias de Investigación (PREI) grant that supported J.D.C.'s sabbatical stay at UNAM. P.P. acknowledges partial support by PAPIIT IG100522.
Declaration of interests
The authors report no conflict of interest.
Appendix A. Conserved quantities
The conserved quantities for the non-dimensional temporal KdV equation are
where ![]() $L$ is the
$L$ is the ![]() $\chi$-period of the solution. The conserved quantities for the non-dimensional tWhitham equation are
$\chi$-period of the solution. The conserved quantities for the non-dimensional tWhitham equation are ![]() $\mathcal {Q}_1$,
$\mathcal {Q}_1$, ![]() $\mathcal {Q}_2$ and
$\mathcal {Q}_2$ and
These quantities are conserved in ![]() $\tau$.
$\tau$.
The conserved quantities for the non-dimensional sKdV equation are
where ![]() $T$ is the
$T$ is the ![]() $\tau$-period of the solution. The conserved quantities for the non-dimensional sWhitham equation are
$\tau$-period of the solution. The conserved quantities for the non-dimensional sWhitham equation are ![]() $\mathcal {Q}_5$,
$\mathcal {Q}_5$, ![]() $\mathcal {Q}_6$ and
$\mathcal {Q}_6$ and
These quantities are conserved in ![]() $\chi$.
$\chi$.