Research Article
Spectra for Infinite Tensor Product Type Actions of Compact Groups
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 330-342
-
- Article
-
- You have access
- Export citation
Superresolution Rates in Prokhorov Metric
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 316-329
-
- Article
-
- You have access
- Export citation
Density of Resonances for Strictly Convex Analytic Obstacles
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 397-447
-
- Article
-
- You have access
- Export citation
Bergman Spaces on Disconnected Domains
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 225-243
-
- Article
-
- You have access
- Export citation
Hardy-Littlewood-Sobolev Theorems of Fractional Integration on Herz-Type Spaces and its Applications
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 363-380
-
- Article
-
- You have access
- Export citation
A Subnormal Operator and its Dual
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 381-396
-
- Article
-
- You have access
- Export citation
Acyclic Models
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 258-273
-
- Article
-
- You have access
- Export citation
Explicit Forms of Local Lifting for GL2
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 343-362
-
- Article
-
- You have access
- Export citation
On the Recovery of Analytic Functions
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 288-301
-
- Article
-
- You have access
- Export citation
Polynomial Lie Subalgebras of the Infinite Matrix Lie Algebra
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 274-287
-
- Article
-
- You have access
- Export citation
A Priori Estimates for Some Classes of Difference Schemes
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 244-257
-
- Article
-
- You have access
- Export citation
A Family of Generalized Riesz Products
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 302-315
-
- Article
-
- You have access
- Export citation

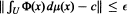 is
is 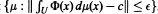 .
.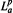 (
(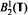 , there are invariant subspaces ℱ such that the dimension of ζℱ in ℱ is infinite.
, there are invariant subspaces ℱ such that the dimension of ζℱ in ℱ is infinite.