We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let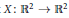 
$X:\,{{\mathbb{R}}^{2}}\to \,{{\mathbb{R}}^{2}}$ be a 
${{C}^{1}}$ map. Denote by 
$\text{Spec}(X)$ the set of (complex) eigenvalues of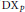 
$\text{D}{{\text{X}}_{p}}$ when 
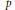$p$ varies in 
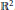${{\mathbb{R}}^{2}}$. If there exists 
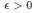$\in \,>\,0$ such that 
$\text{Spec(}X)\,\bigcap \,(-\in ,\,\in )\,=\,\varnothing $, then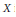 
$X$ is injective. Some applications of this result to the real Keller Jacobian conjecture are discussed.
