We consider the weak closure WZ of the set Z of all feasible pairs (solution, flow) of the
family of potential elliptic systems
$$
\begin{array}{c}\mbox{div}\left(\sum\limits_{s=1}^{s_0}\sigma_s(x)F_s^\prime
(\nabla u(x)+g(x))-f(x)\right)=0\;\mbox{in}\,\Omega,
u=(u_1,\dots, u_m)\in H_0^1(\Omega;{\bf R}^m),\;\sigma=(\sigma_1,\dots,\sigma_{s_0})\in S,\end{array}
$$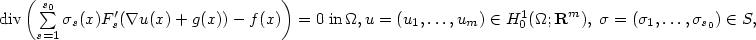 where Ω ⊂ Rn is a bounded Lipschitz domain, Fs are strictly convex smooth
functions with quadratic growth and $S=\{\sigma\,
measurable\,\mid\,\sigma_s(x)=0\;\mbox{or}\,1,\;s=1,\dots,s_0,\;\sigma_1(x)+\cdots +\sigma_{s_0}(x)=1\}$
where Ω ⊂ Rn is a bounded Lipschitz domain, Fs are strictly convex smooth
functions with quadratic growth and $S=\{\sigma\,
measurable\,\mid\,\sigma_s(x)=0\;\mbox{or}\,1,\;s=1,\dots,s_0,\;\sigma_1(x)+\cdots +\sigma_{s_0}(x)=1\}$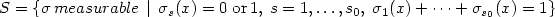 .
We show that WZ is the zero level set for an integral functional with the integrand $Q\cal F$
.
We show that WZ is the zero level set for an integral functional with the integrand $Q\cal F$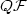 being
the A-quasiconvex envelope for a certain function $\cal F$
being
the A-quasiconvex envelope for a certain function $\cal F$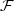 and the operator A = (curl,div)m.
If the functions Fs are isotropic, then on the characteristic cone Λ (defined by the operator
A) $Q{\cal F}$
and the operator A = (curl,div)m.
If the functions Fs are isotropic, then on the characteristic cone Λ (defined by the operator
A) $Q{\cal F}$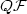 coincides with the
A-polyconvex envelope of $\cal F$
coincides with the
A-polyconvex envelope of $\cal F$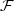 and can be computed by means of rank-one laminates.
and can be computed by means of rank-one laminates.